Two capacitors with greater than and are connected in series with a battery. Which of the following is true?
There is move charge stored on
There is more charge stored on
There is the same charge stored on each capacitor
There is the same potential difference across both capacitors
The correct Answer is:C
To solve the question regarding two capacitors CA and CB connected in series with a battery, we can follow these steps:
Step 1: Understand the Series Connection of Capacitors
In a series connection, the same charge Q flows through each capacitor. This means that the charge on both capacitors is equal.
Hint: Remember that in a series circuit, the charge on each component is the same.
Step 2: Analyze the Charge on Each Capacitor
Since the capacitors are in series, the charge on capacitor CA is equal to the charge on capacitor CB:
QA=QB=Q
Hint: Use the concept of charge conservation in series circuits.
Step 3: Relate Charge and Capacitance to Potential Difference
The potential difference across each capacitor can be expressed using the formula:
V=QC
For capacitor CA:
VA=QCA
For capacitor CB:
VB=QCB
Hint: Remember that the potential difference across a capacitor is inversely proportional to its capacitance.
Step 4: Compare the Potential Differences
Since CA>CB, we can conclude:
VA<VB
This means that the potential difference across capacitor CA is less than that across capacitor CB.
Hint: Think about how capacitance affects the voltage drop across each capacitor.
Step 5: Conclusion
From the analysis, we can conclude that:
- The charge on both capacitors is the same.
- The potential difference across each capacitor is different, with VA<VB.
Thus, the correct statement is that there is the same charge stored on each capacitor.
Final Answer: The correct option is that there is the same charge stored on each capacitor.
In a series connection, the same charge Q flows through each capacitor. This means that the charge on both capacitors is equal.
Since the capacitors are in series, the charge on capacitor CA is equal to the charge on capacitor CB:
QA=QB=Q
The potential difference across each capacitor can be expressed using the formula:
V=QC
For capacitor CA:
VA=QCA
For capacitor CB:
VB=QCB
Since CA>CB, we can conclude:
VA<VB
This means that the potential difference across capacitor CA is less than that across capacitor CB.
From the analysis, we can conclude that:
- The charge on both capacitors is the same.
- The potential difference across each capacitor is different, with VA<VB.
Topper's Solved these Questions
- CAPACITANCEBOOK - RESNICK AND HALLIDAYCHAPTER - CAPACITANCEEXERCISE - PRACTICE QUESTIONS (MORE THAN ONE CORRECT CHOICE TYPE)
12 Videos
- CAPACITANCEBOOK - RESNICK AND HALLIDAYCHAPTER - CAPACITANCEEXERCISE - PRACTICE QUESTIONS (LINKED COMPREHENSION)
8 Videos
- CAPACITANCEBOOK - RESNICK AND HALLIDAYCHAPTER - CAPACITANCEEXERCISE - PROBLEMS
48 Videos
- ALL ABOUT ATOMS BOOK - RESNICK AND HALLIDAYCHAPTER - ALL ABOUT ATOMS EXERCISE - CHECKPOINT
1 Videos
- CENTER OF MASS BOOK - RESNICK AND HALLIDAYCHAPTER - CENTER OF MASS EXERCISE - Practice Questions (Integer )
4 Videos
Similar Questions
Two capacitors of capacitance 10μFand20μF are connected in series with a 6 V battery. If E is the energy stored in 20μF capacitor what will be the total energy supplied by the battery in terms of E.
View SolutionTwo capacitors of capacitances 4μF and 6μF are connected in series with a battery of 20 V. find the energy supplied by the battery.
View Solution
Knowledge Check
Two unequal capacitors, initially uncharged, are connected in series across a battery. Which of the following is true.
AThe potential across each is the sameBThe charge on each is the sameCThe energy stored in each is the sameDThe equivalent capacitance is the sum of the two capacitancesA resistor, a capacitor and inductor are connected in series with a source of ac which of the following statement is true? The current in resistor lags behind the
Acurrent in capacitorBcurrent in inductorCvoltage across capacitorDvoltage across inductorTwo capacitors of 10μF and 20μF are connected in series with a 30V battery. The charge on the capacitors will be, respectively
A100μC,200μCB200μC,100μCC100μC,100μCD200μC,200μC
Two capacitors of capacitance of 6μFand12μF are connected in series with a battery. The voltage across the 6μF capacitor is 2V. Compute the total battery voltage.
View SolutionTwo capacitors of capacitance of 6μFand12μF are connected in series with a battery. The voltage across the 6μF capacitor is 2 V.Compute the total battery voltage.
View SolutionTwo capacitors A and B are connected in series with a battery as shown in the figure. When the switch S is closed and the two capacitors get charged fully, then
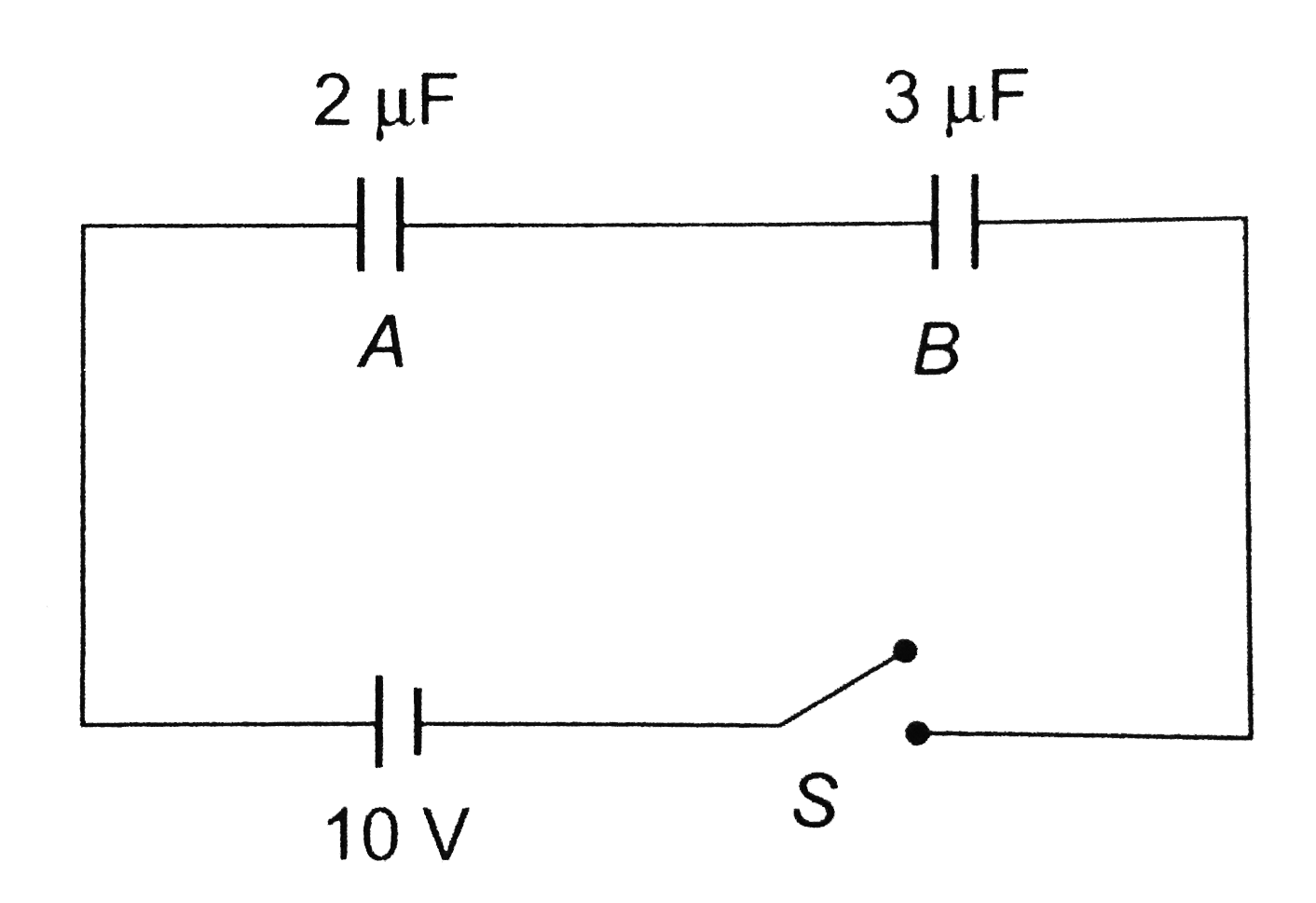
View SolutionTwo capacitors of capacities 1μF and 4μF are connected in series with battery of 200V. The voltage across them are in the ratio of
View SolutionTwo identical capacitor C1 and C2 are connected in series with a battery. They are fully charged. Now a dielectric slab is inserted between the plates of C2. The potential across C1 will :
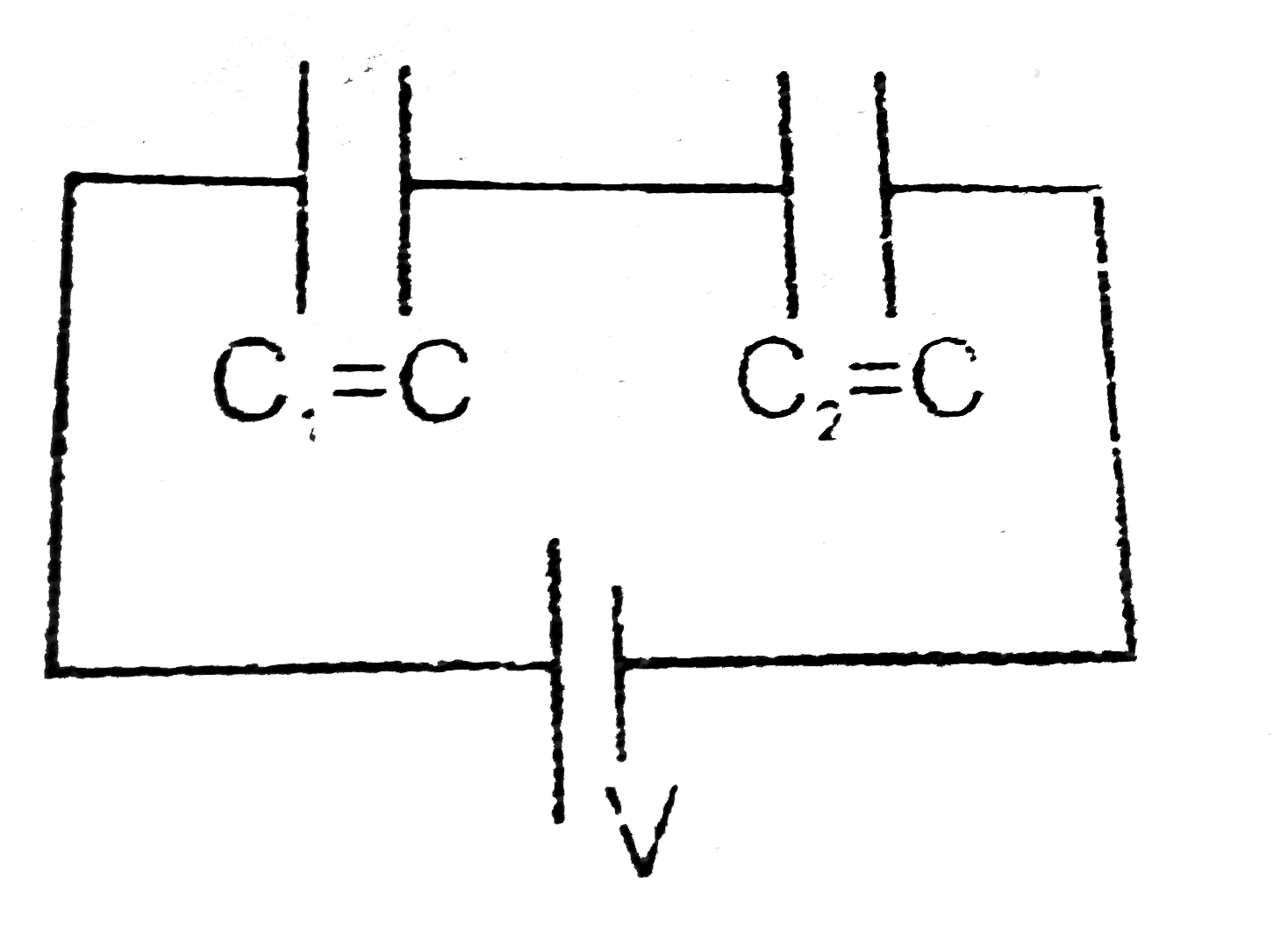
View Solution
