A point object P moves towards a convex mirror with a constant speed v, along its optic axis.The speed of the image
Is always less than v
May be greater than and equal to v or less than v depending on the position of P
Increases as P comes closer to the mirror
Decreases as P comes closer to the mirror
The correct Answer is:A, C
To find the speed of the image formed by a convex mirror when a point object P moves towards it with a constant speed v, we can follow these steps:
Step 1: Understand the Mirror Formula
The mirror formula for a convex mirror is given by:
1v+1u=1f
where:
- v = image distance (positive for virtual images in convex mirrors)
- u = object distance (negative for real objects in front of the mirror)
- f = focal length (positive for convex mirrors)
Step 2: Differentiate the Mirror Formula
To find the speed of the image, we need to differentiate the mirror formula with respect to time t:
ddt(1v)+ddt(1u)=0
This gives us:
−1v2dvdt−1u2dudt=0
Step 3: Rearranging the Equation
Rearranging the differentiated equation leads to:
dvdt=−u2v2dudt
Here, dudt is the speed of the object, which is given as v0 (the constant speed of the object moving towards the mirror).
Step 4: Substitute the Values
Substituting dudt=−v0 (negative because u is decreasing as the object approaches the mirror):
dvdt=u2v2v0
This indicates that the speed of the image dvdt is positive, meaning the image is moving away from the mirror.
Step 5: Analyze Image Speed
As the object approaches the mirror, u decreases. The image speed dvdt will increase as u decreases, indicating that the image moves faster as the object gets closer to the mirror.
Step 6: Conclusion
The speed of the image is always less than the speed of the object. As the object approaches the mirror, the speed of the image increases, reaching a maximum as the object approaches the focal point of the mirror.
Final Result
The speed of the image dvdt can be expressed as:
dvdt=u2v2v0
where v0 is the speed of the object.
---
The mirror formula for a convex mirror is given by:
1v+1u=1f
where:
- v = image distance (positive for virtual images in convex mirrors)
- u = object distance (negative for real objects in front of the mirror)
- f = focal length (positive for convex mirrors)
To find the speed of the image, we need to differentiate the mirror formula with respect to time t:
ddt(1v)+ddt(1u)=0
This gives us:
−1v2dvdt−1u2dudt=0
Rearranging the differentiated equation leads to:
dvdt=−u2v2dudt
Here, dudt is the speed of the object, which is given as v0 (the constant speed of the object moving towards the mirror).
Substituting dudt=−v0 (negative because u is decreasing as the object approaches the mirror):
dvdt=u2v2v0
This indicates that the speed of the image dvdt is positive, meaning the image is moving away from the mirror.
As the object approaches the mirror, u decreases. The image speed dvdt will increase as u decreases, indicating that the image moves faster as the object gets closer to the mirror.
The speed of the image is always less than the speed of the object. As the object approaches the mirror, the speed of the image increases, reaching a maximum as the object approaches the focal point of the mirror.
The speed of the image dvdt can be expressed as:
dvdt=u2v2v0
where v0 is the speed of the object.
Topper's Solved these Questions
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Linked Comprehension)2videos
GEOMETRICAL OPTICS : REFLECTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (Integer Type)3videos
GEOMETRICAL OPTICS : REFLECTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFLECTION EXERCISE - PRACTICE QUESTIONS (single correct choice type)27videos
GEOMETRICAL OPTICS : REFLECTION
- BOOK - RESNICK AND HALLIDAYCHAPTER - GAUSS' LAW EXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)3videos
GAUSS' LAW
- BOOK - RESNICK AND HALLIDAYCHAPTER - GEOMETRICAL OPTICS : REFRACTIONEXERCISE - Practice questions(Integers)4videos
GEOMETRICAL OPTICS : REFRACTION
Similar Questions
A particle is moving at a constant speed V from a large distance towards a concave mirror of radius R along its principal axis. Find the speed of the image formed by the mirror as a function of the distance x of the particle from the mirror.
View SolutionWhen an object is moved away from a convex mirror, the image
View Solution
Knowledge Check
- Question 1 - Select One
As an object moves towards a convex mirror, the image
Amagnification increasesBmoves towards the mirrorCNeither (a) nor (b) happensDBoth (1) and (2) happen - Question 2 - Select One
An object is moving towards a convex mirror with a constant velocity. P is the pole of mirror.
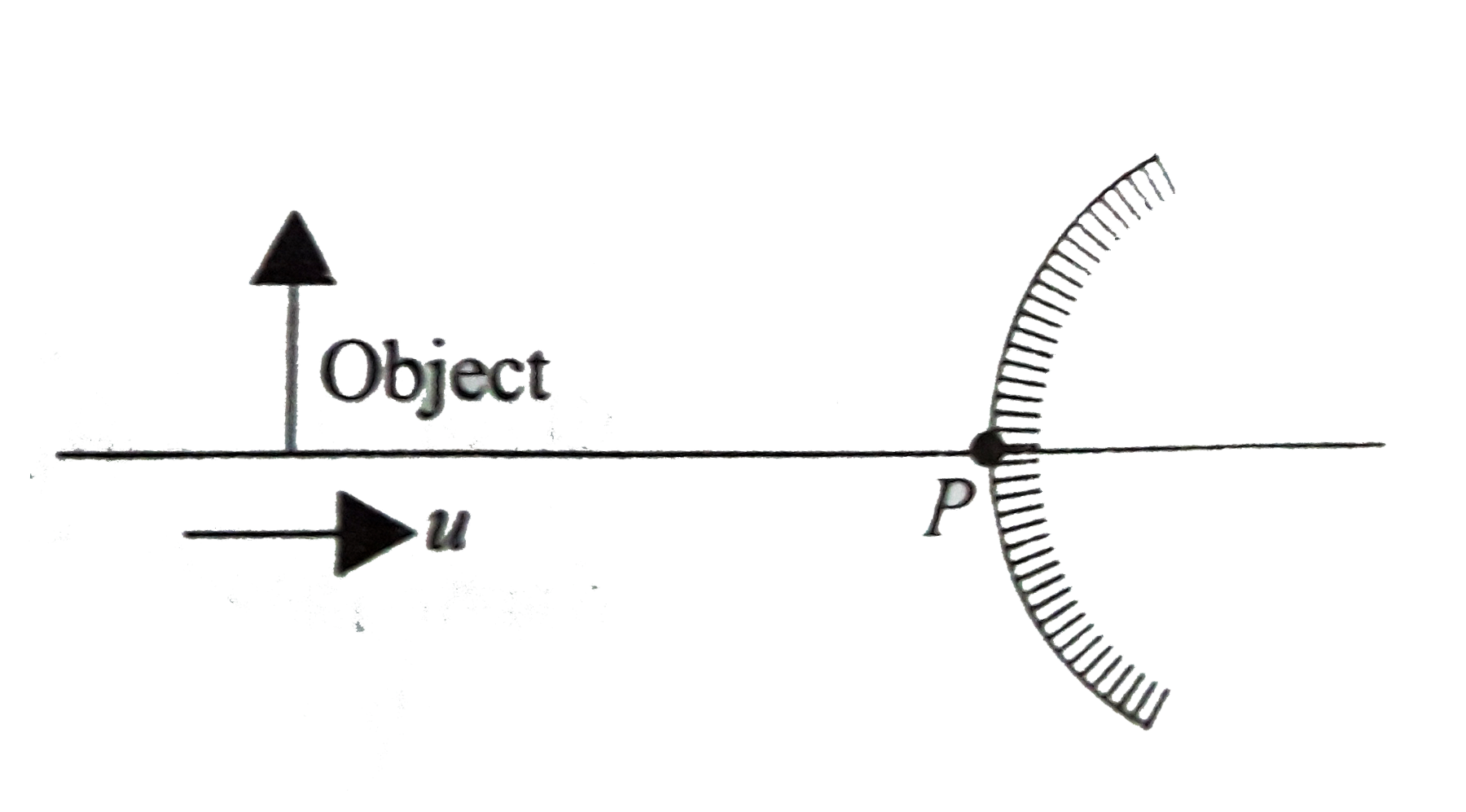 Amagnification of the image increases with time.Bmagnificatin of the image decreases with timeCvelocity of the image increases with timeDvelocity of the image decreases with time
Amagnification of the image increases with time.Bmagnificatin of the image decreases with timeCvelocity of the image increases with timeDvelocity of the image decreases with time - Question 3 - Select One
A particle moves perpendicular towards a plane mirror with a constant speed of 4cm/s. What is the speed of the image observed by an observed moving with 2cm/s along the same direction? Mirror is also moving with a speed of 10cm/s in the opposite direction.
(All speeds are with respect to ground frame of reference)A4cm/sB12cm/sC14cm/sD26cm/s

If an object moves towards a plane mirror with a speed v at an angle θ to the perpendicular to the plane of the mirror, find the relative velocity between the object and the image.View SolutionWhen an object moves towards a convex lens, the size of the image
View SolutionAn observer moves towards a plane mirror with a speed of 2msec. The speed of the image with respect to the observer is
View SolutionAn observer moves towards a plane mirror with a speed of 2 m/s . The speed of the image with respect to the observer is
View SolutionWhen an object moves towards a convex lens, the size of the image
View Solution

