If one mole of electrons is passing from point P to another point Q on a straight metal wire in time interval of 1 ms, then what will be the magnitude of current in the wire ? What is the direction of current ?
To solve the problem, we need to calculate the magnitude of the current flowing through the wire when one mole of electrons passes from point P to point Q in a time interval of 1 millisecond.
Step 1: Calculate the total charge (Q)
One mole of electrons contains Avogadro's number of electrons, which is approximately 6.022×1023 electrons. The charge of a single electron is approximately 1.6×10−19 coulombs.
The total charge (Q) can be calculated using the formula:
Q=n×e
where:
- n is the number of moles of electrons (1 mole)
- e is the charge of a single electron (1.6×10−19 coulombs)
Substituting the values:
Q=1×6.022×1023×1.6×10−19
Calculating this gives:
Q=6.022×1.6×104≈9.6352coulombs
Step 2: Convert time to seconds
The time interval given is 1 millisecond. We need to convert this to seconds:
t=1ms=1×10−3s
Step 3: Calculate the current (I)
The current (I) can be calculated using the formula:
I=Qt
Substituting the values we calculated:
I=9.63521×10−3=9635.2A
Step 4: Determine the direction of current
The direction of conventional current is opposite to the flow of electrons. Since electrons are moving from point P to point Q, the conventional current flows from point Q to point P.
Final Answer
The magnitude of the current in the wire is approximately 9635.2A and the direction of the current is from point Q to point P.
---
One mole of electrons contains Avogadro's number of electrons, which is approximately 6.022×1023 electrons. The charge of a single electron is approximately 1.6×10−19 coulombs.
Q=n×e
where:
- n is the number of moles of electrons (1 mole)
- e is the charge of a single electron (1.6×10−19 coulombs)
Q=1×6.022×1023×1.6×10−19
Calculating this gives:
Q=6.022×1.6×104≈9.6352coulombs
The time interval given is 1 millisecond. We need to convert this to seconds:
t=1ms=1×10−3s
The current (I) can be calculated using the formula:
I=Qt
Substituting the values we calculated:
I=9.63521×10−3=9635.2A
The direction of conventional current is opposite to the flow of electrons. Since electrons are moving from point P to point Q, the conventional current flows from point Q to point P.
The magnitude of the current in the wire is approximately 9635.2A and the direction of the current is from point Q to point P.
Topper's Solved these Questions
- BOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE PROBLEMS71videos
CURRENT ELECTRICITY
- BOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - CONCEPTUAL QUESTIONS35videos
CURRENT ELECTRICITY
- BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test16videos
ATOMS
- BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST15videos
DUAL NATURE OF RADIATION AND MATTER
Similar Questions
Knowledge Check
- Question 1 - Select One
What constitutes current in a metal wire ?
AElectronsBProtonsCAtomsDMolecules - Question 2 - Select One
What constitutes current in a metal wire?
AElectronsBProtonsCNeutronsDNone of these - Question 3 - Select One
What constitutes current in a metal wire ?
AelectronsBprotonsCatomsDmolecules
If 106 electrons pass from a point towards another point B in a conductor in one microsecond. Find the magnitude and direction of current. Give charge of an electron is 1.6×10−19 C.
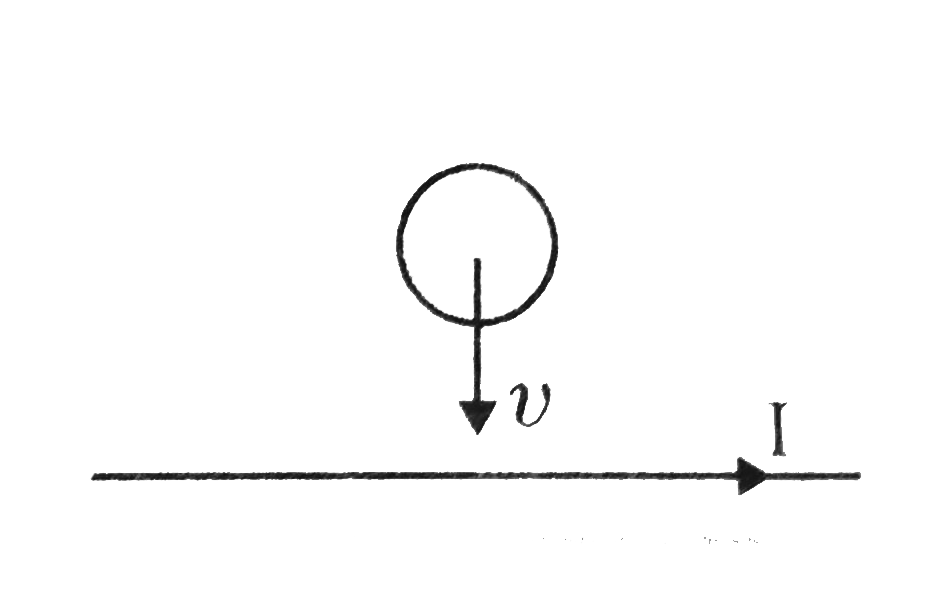
View SolutionA metal ring moves towards a straingt wire carrying current. What is the direction of induced current in the ring ?
 View Solution
View SolutionA long straight wire carries a current of 35 A. What is the magnitude of the field →B at a point 20 cm from the wire?
View SolutionTen million electrons pass from point P to point Q m one micro second. The current and its direction is
 View Solution
View SolutionIf a long straight wire carries a current of 40 A, then the magnitude of the field B at a point 15 cm away from the wire is
View Solution