A thin copper rod of radius 1mm having an electrical resistance of 100Ω is connected to battery of emf 12 volts and negligible internal resistance through a wire of negligible resistance. Calculate current density in the wire. Also , calculate the number of electrons entering one and of the rod per seconds.
To solve the problem, we will follow these steps:
Step 1: Calculate the Current (I) using Ohm's Law
Ohm's Law states that V=I⋅R, where:
- V is the voltage (emf of the battery),
- I is the current,
- R is the resistance.
Given:
- V=12volts
- R=100Ω
Rearranging the formula to find I:
I=VR=12volts100Ω=0.12A
Step 2: Calculate the Cross-sectional Area (A) of the Rod
The cross-sectional area A of a circular rod can be calculated using the formula:
A=πr2
Where r is the radius of the rod. Given:
- Radius r=1mm=1×10−3m
Calculating the area:
A=π(1×10−3)2=π(1×10−6)≈3.14×10−6m2
Step 3: Calculate the Current Density (J)
Current density J is defined as the current per unit area:
J=IA
Substituting the values we calculated:
J=0.12A3.14×10−6m2≈3.83×104A/m2
Step 4: Calculate the Number of Electrons Entering One End of the Rod per Second
The total charge Q flowing through the rod in one second can be calculated using:
Q=I⋅t
Where t=1s:
Q=0.12A⋅1s=0.12C
The number of electrons n can be calculated using the formula:
n=Qe
Where e (the charge of an electron) is approximately 1.6×10−19C:
n=0.12C1.6×10−19C/electron≈7.5×1017electrons
Summary of Results
- Current Density J≈3.83×104A/m2
- Number of Electrons per Second n≈7.5×1017
Ohm's Law states that V=I⋅R, where:
- V is the voltage (emf of the battery),
- I is the current,
- R is the resistance.
- V=12volts
- R=100Ω
I=VR=12volts100Ω=0.12A
The cross-sectional area A of a circular rod can be calculated using the formula:
A=πr2
Where r is the radius of the rod. Given:
- Radius r=1mm=1×10−3m
A=π(1×10−3)2=π(1×10−6)≈3.14×10−6m2
Current density J is defined as the current per unit area:
J=IA
Substituting the values we calculated:
J=0.12A3.14×10−6m2≈3.83×104A/m2
The total charge Q flowing through the rod in one second can be calculated using:
Q=I⋅t
Where t=1s:
Q=0.12A⋅1s=0.12C
n=Qe
Where e (the charge of an electron) is approximately 1.6×10−19C:
n=0.12C1.6×10−19C/electron≈7.5×1017electrons
- Current Density J≈3.83×104A/m2
- Number of Electrons per Second n≈7.5×1017
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE PROBLEMS
71 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - CONCEPTUAL QUESTIONS
35 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
A bulb of resistance 5Ω is connected to a battery of emf 4.2 V and internal resistance 1Ω. Find the current through the bulb.
View SolutionA potentiometer wire is 10 m long and has a resistance of 18Ω. It is connected to a battery of emf 5 V and internal resistance 2Ω. Calculate the potential gradient along the wire.
View Solution
Knowledge Check
A wire of length 1.6m has a resistance 8Ω is connected to a battery of 2 volts and internal resistance 2Ω. What is the potential gradient ?
A1.5V/mB0.5V/mC1V/mD2V/mA 10 m long wire ofresistance 20Ω is connected in series with battery of EMF 3V and negligible internal resistance and a resistance of 10Ω. The potential gradient along the wire is :
A0.02 V/mB0.1 V/mC0.2 V/mD1.2 V/mA potentiometer wire has length 10m and resistance 10Ω. It is connected to battery of EMF 11 volt and internal resistance 1Ω, then the potential gradient in the wire is
A10V/mB1V/mC0.1V/mDnone
A potentiometer wire , 4 m long and having a resistance of 10 Ω, is connected in series with an external resistance of 38Ω and a cell , of emf 2 V and internal resistance 2Ω. Calculate the potential gradient along the wire.
View SolutionA copper wire of radius 0.1mm and resistance 1k(Ω)is connected across a power supply of 20 V.(a)How man electorns are transferred per second between the supply and the wire at one end?(b) Write down the current density in the wire.
View SolutionA copper wire of radius 0.1 mm and resistance 1kΩ is connected across a power supply of 20 V. (i) How many electrons are transferred per second between the supply and the wire at one end ? (ii) Write down the current density in the wire.
View Solution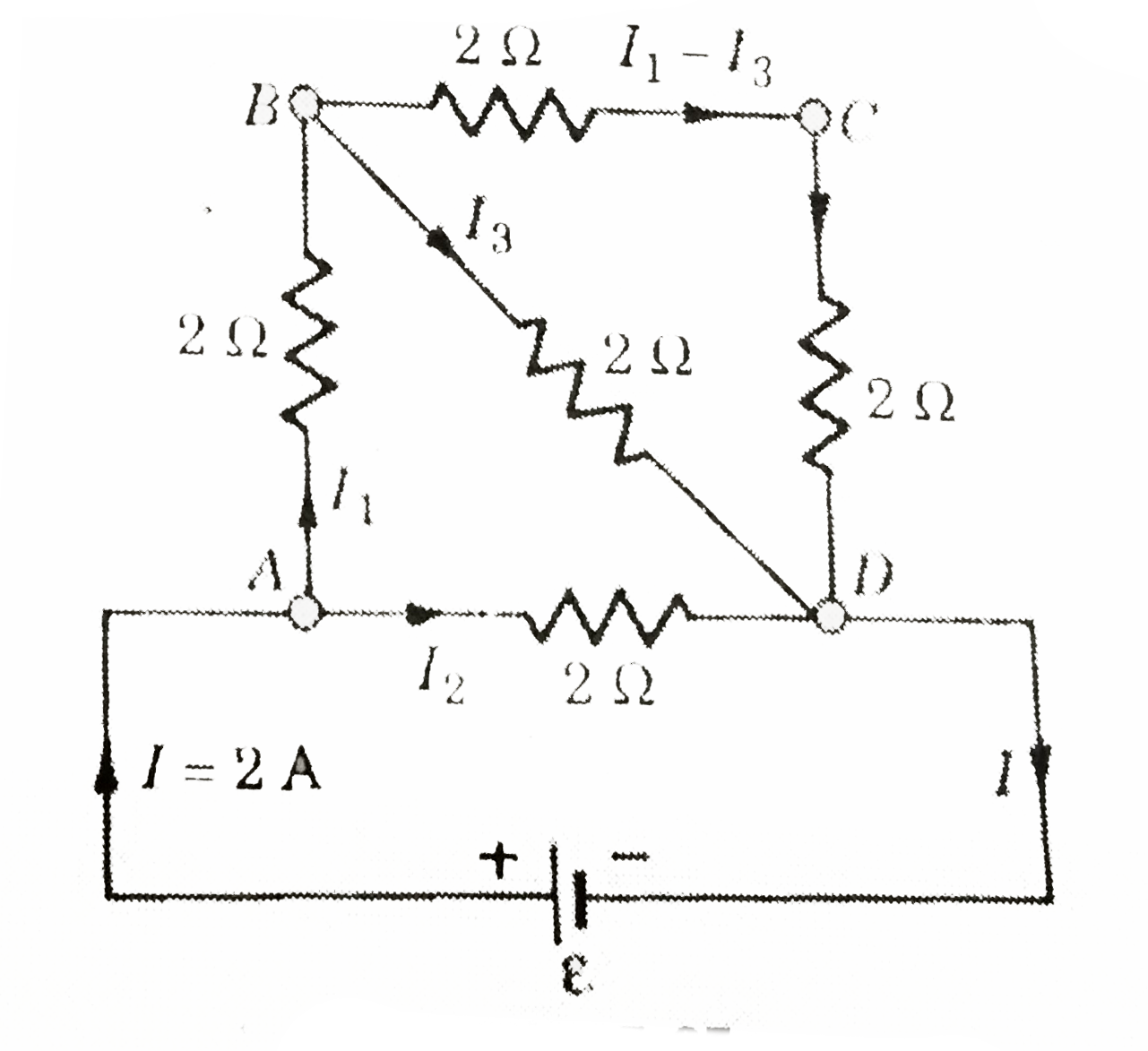
A network of resistors is connected to a battery of negligible internal resistance, as shown in figure. Calculate the equivalent resistance between the point A and D, and the value of the current I3.
View SolutionPotentiometer wire length is 10m, having a total resistance of 10Ω, if a battery of emf 2 volt negligible internal resistance and a rheostat is connected to it then potential gradient is 20mV/m find the resistance applied through rheostat:-
View Solution
