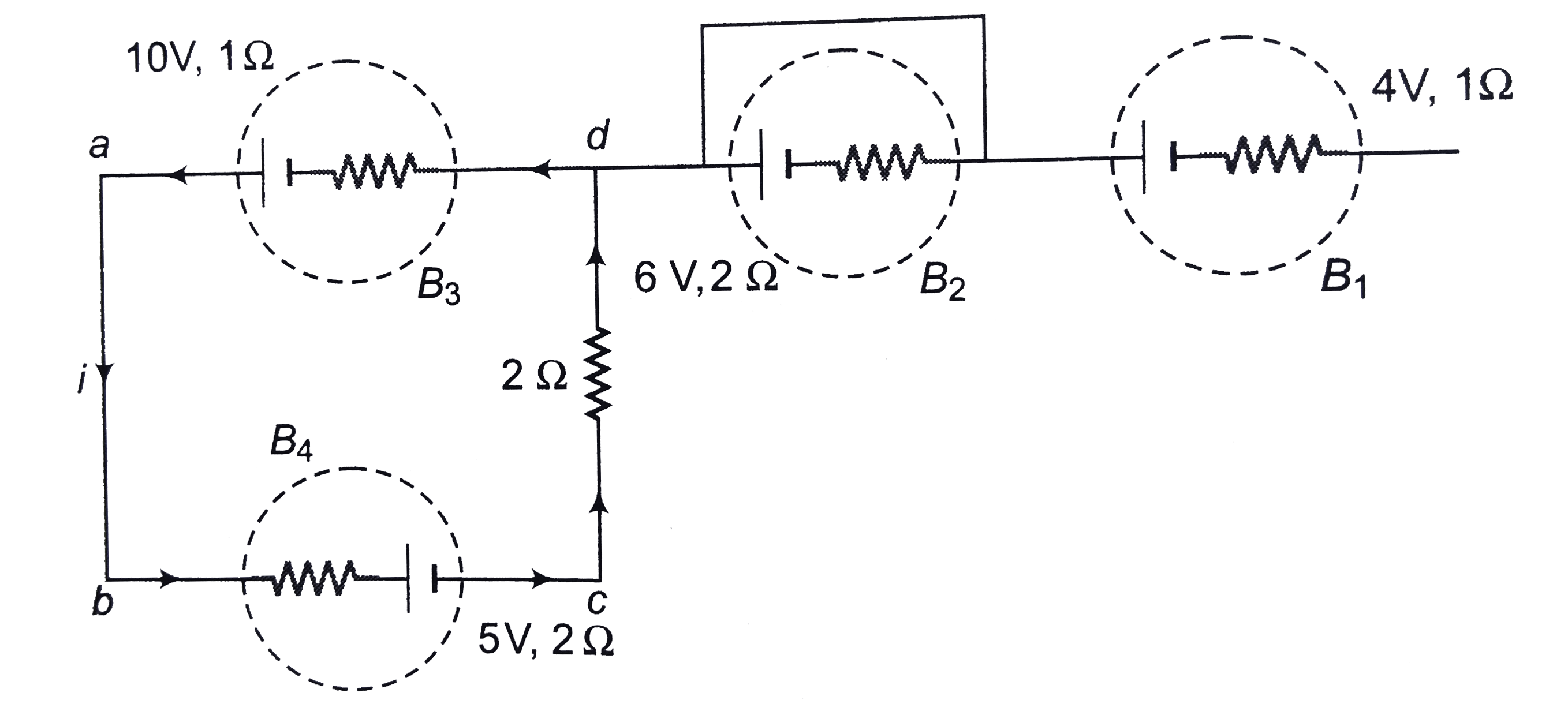
Find the potential difference across each cell and the rate of energy dissipated in R in the given figure .

Text Solution

Applying KVL to loop ADCBA, we get: −4(i1+i2)−2i1+12=0
⇒4(i1+i2)+2i1−12=0
⇒6i1+4i2−12=0 …(i)
For loop ADEFA, we have
−4(i1+i2)−i2+6=0
4(i1+i2)+i2−6=0
4i1+5i2−6=0 ...(ii)
Solving (i) and (ii), we get:
i1+187Aandi2=67A
Potential difference across R=(i1+i2)×R
=(187−67)×4=487V
Potential difference across each cell= Potential difference across R
=487V
Energy dissipated in R is given by: H=(i1+i2)2R
=(127)2×4=57649J=11.75J
|
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - PRACTICE PROBLEMS
71 Videos
- CURRENT ELECTRICITYBOOK - MODERN PUBLICATIONCHAPTER - CURRENT ELECTRICITYEXERCISE - CONCEPTUAL QUESTIONS
35 Videos
- ATOMS BOOK - MODERN PUBLICATIONCHAPTER - ATOMS EXERCISE - Chapter Practice Test
16 Videos
- DUAL NATURE OF RADIATION AND MATTER BOOK - MODERN PUBLICATIONCHAPTER - DUAL NATURE OF RADIATION AND MATTER EXERCISE - CHAPTER PRACTICE TEST
15 Videos
Similar Questions
Knowledge Check
Potential different across the terminals of the battery shown in figure is
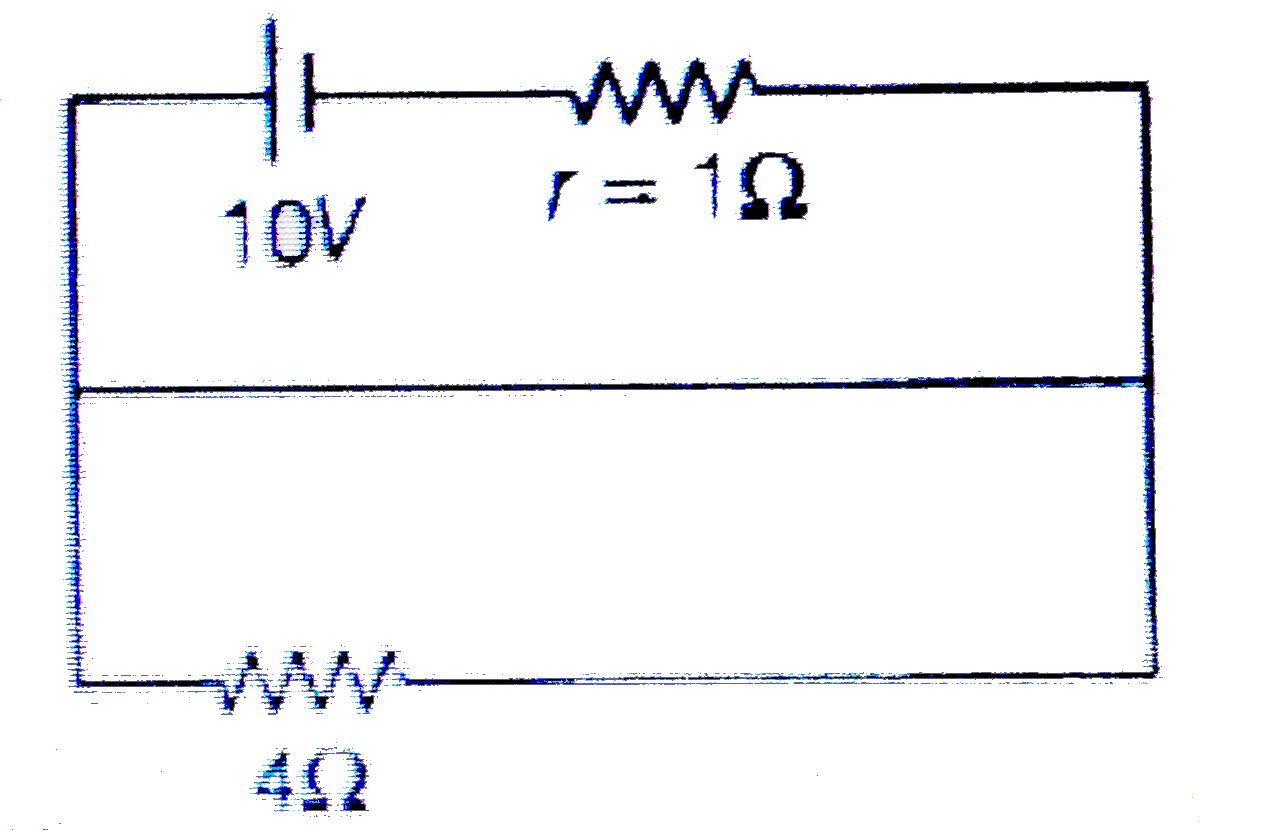 A8 VB10 VC6 VDzero
A8 VB10 VC6 VDzero
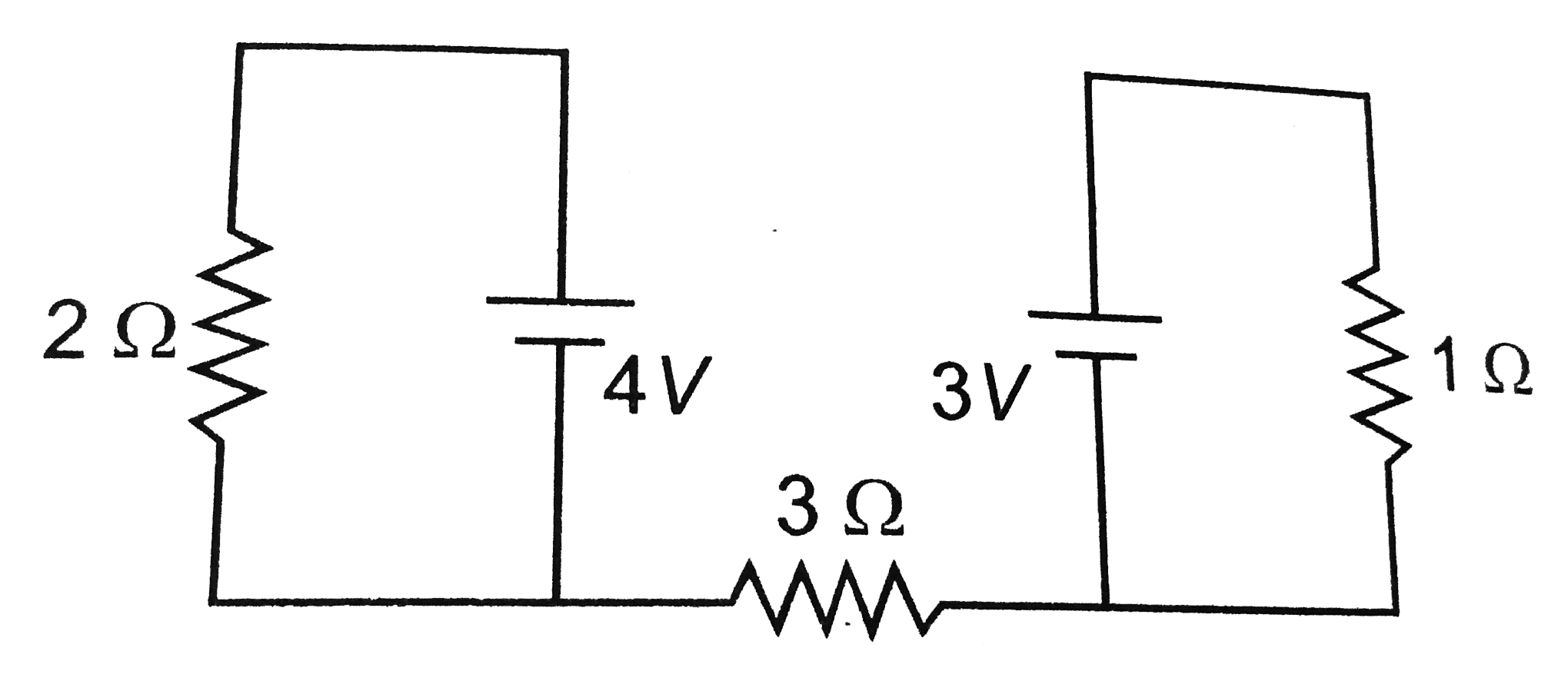
The potential difference across the 3 resistor shown in figure is
View SolutionPotential difference across AB in the circuit as shown in figure.
View SolutionPotential difference across AB in the network shown in figure.
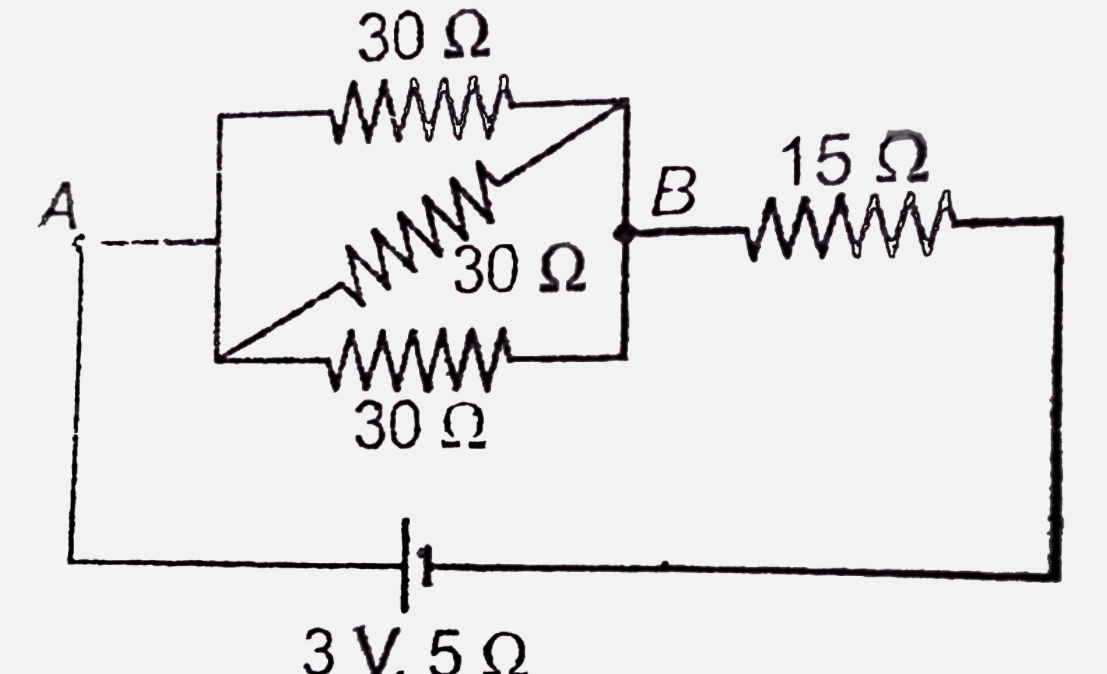
View SolutionPotential difference across AB in the circuit as shown in figure.

View SolutionPotential difference across AB in the network shown in figure.

View SolutionPotential different across the terminals of the battery shown in figure is
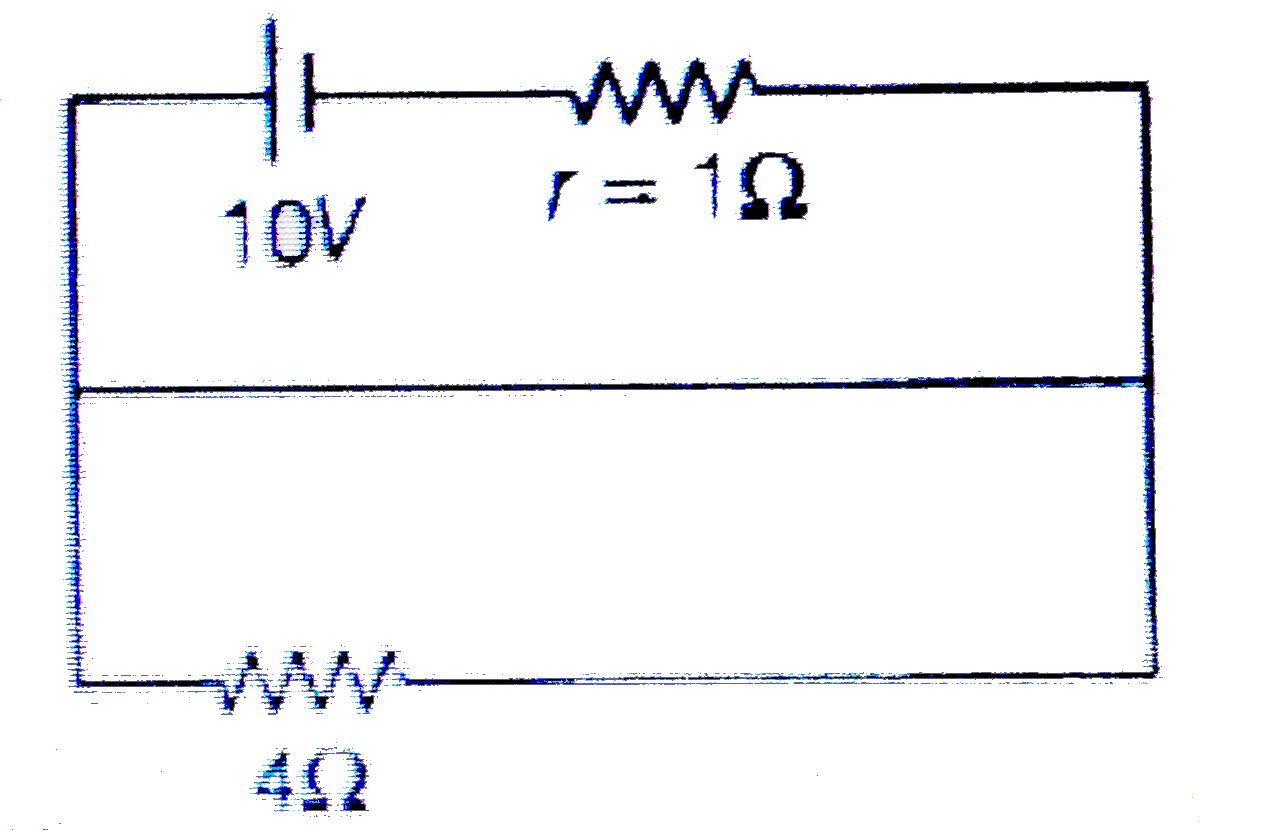
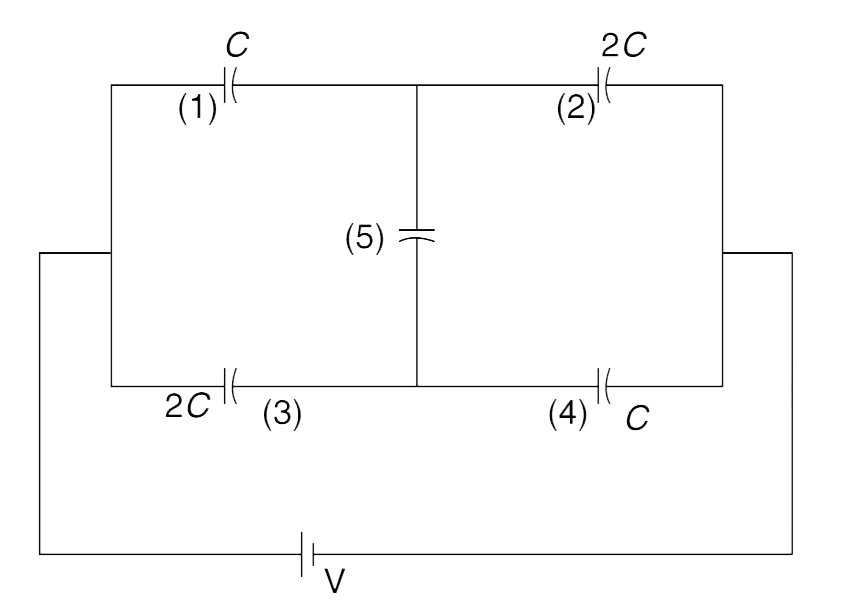
View SolutionIn the circuit shown in the Figure, find the ratio of potential difference across capacitor 1 and 2. The capacitance values are as indicated in the Figure.
View Solution