एक रेखाखण्ड AB पर AD और BC दो बराबर लम्ब रेखाखण्ड है | ( देखीए आकृति ) | दर्शाइए कि CD, रेखाखण्ड AB को समद्धिभाजीत करता है |
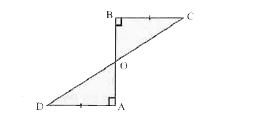
The correct Answer is:AO -= BO
Topper's Solved these Questions
- त्रिभुज BOOK - SCIENCE PUBLICATIONCHAPTER - त्रिभुज EXERCISE - प्रश्नावली 7.2
8 Videos
- त्रिभुज BOOK - SCIENCE PUBLICATIONCHAPTER - त्रिभुज EXERCISE - प्रश्नावली 7.3
5 Videos
- त्रिभुज BOOK - SCIENCE PUBLICATIONCHAPTER - त्रिभुज EXERCISE - प्रश्नावली 7.4
6 Videos
- चतुर्भुज BOOK - SCIENCE PUBLICATIONCHAPTER - चतुर्भुज EXERCISE - प्रश्नावली 8.2 पर आधारित अभ्यास प्रश्न
7 Videos
- दो चरों वाले रैखिक समीकरणBOOK - SCIENCE PUBLICATIONCHAPTER - दो चरों वाले रैखिक समीकरणEXERCISE - प्रश्नावली 4.4 पर आधारित अभ्यास प्रश्न
1 Videos
Similar Questions
एक समद्धिबाहू त्रिभुज ABC में जिसमे AB -= AC है, ∠B और ∠C के समद्धिभाजक परस्पर बिन्दु O पर प्रतिच्छेद करते है | A और O को जोडिए | दर्शाइए कि
(i) OB -= OC
(ii) AO कोण A को समद्धिभाजित करता है |
View SolutionΔABC और ΔDBC एक ही आधार BC पर बने दो समद्धिबाहू त्रिभुज इस प्रकार है कि A और D भुजा BC के एक ही ओर स्थित है | (देखिए आक्रति ) | यदि AD बढ़ाने पर BC को P पर प्रतिच्छेद करे, तो दर्शाइए कि-
(i) ΔABD ~-= ΔACD
(ii) ΔABP ~-= ΔACP
(iii) AP कोण A और कोण D दोनों को समद्धिभाजित करता है |
(iv) AP रेखाखण्ड BC का लम्ब समद्धिभाजक है |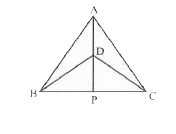
View Solutionदर्शाइए क समकोण त्रिभुज में कोण सबसे लम्बी भुजा होती है |
View SolutionP, Q और R क्रमशः एक समबाहु त्रिभुज ABC की भुजाओं BC, CA और AB के मध्य बिन्दु है। सिद्ध कीजिए कि ΔPQR भी एक समबाहु त्रिभुज हैं।
View Solutionनिम्नलिखित समीकरणों को (i) संख्या रेखा और तथा (ii) कार्तिए तल पर प्रदर्शित कीजिए।
(a) x−2=0 " (b)2x+4=3x+1
(c) y+5=0
View SolutionIn the given figure (not to scale), O is the centre of the circle. A,B,C and D are concyclic and AB=CD . If /_MON =120^(@) , then find /_ OPN .
View SolutionHow many lines of symmetry does the above figure have ?
View SolutionWhich of the following are correct chain isomers of butane ? (i)
View SolutionDetermine the point of symmetry of a regular hexagon.
View SolutionDtermine the images of the following figure about the given line :
View SolutionIn the figure above (not to scale), ¯¯¯¯¯¯AB⊥¯¯¯¯¯¯CDandAD is the bisector of ∠BAE.AB=3cm and AC=5cm. Find CD.
View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionMatch the following Column A to Column B

View SolutionThe inequation represented by the graph given below is :
View SolutionThe inequation that best describes the graph given below is _________
View Solution
