Let AP and BQ be two vertical poles at points A and B, respectively. If AP = 16 m, BQ = 22 m and AB = 20 m, then find the distance of a point R on AB from the point A such that RP2+RQ2 is minimum.Solution in Bengali
Step by step video & image solution for Let AP and BQ be two vertical poles at points A and B, respectively. If AP = 16 m, BQ = 22 m and AB = 20 m, then find the distance of a point R on AB from the point A such that RP^(2) + RQ^(2) is minimum. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- APPLICATION OF DERIVATIVESBOOK - NCERT BANGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.1
18 Videos
- APPLICATION OF DERIVATIVESBOOK - NCERT BANGLISHCHAPTER - APPLICATION OF DERIVATIVESEXERCISE - EXERCISE 6.2
23 Videos
- APPLICATION OF INTEGRALSBOOK - NCERT BANGLISHCHAPTER - APPLICATION OF INTEGRALSEXERCISE - Miscellaneous Exercise
19 Videos
Similar Questions
Find the rate of change of the area of a circle with respect to its radius r when
(a) r=3 cm (b) r=4 cm
View SolutionShow that the function given by f (x) = 3x + 17 is increasing on R.
View SolutionFind the area under the given curves and given lines:
(i) y=x2,x=1,x=2 and x-axis
(ii) y=x4,x=1,x=5 and x-axis
View SolutionLet AP and BQ be two vertical poles at points A and B, respectively. If AP=16m,BQ=22mandAB=20m, then find the distance of a point R on AB from the point A such that RP2+RQ2is minimum.
View SolutionIn Figure, AP and BQ are perpendiculars to the line segment AB and AP=BQ. Prove that O is the mid-point of line segment AB and PQ.
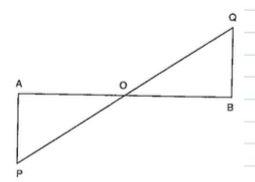
View SolutionM is the midpoint of side AB of equilateral triangle ABC.P is a point on BC such that (AP+PM) is minimum.If AB=20 then AP+PM is
View SolutionIn Figure, AP and BQ are perpendiculars to the line segment AB and AP=BQ . Prove that O is the mid-point of line segment AB and PQ
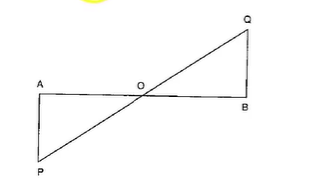
View SolutionLet AP and BQ be two vertical poles at points A and B respectively. If AP=16m, BQ=22m and AB=20m , then find the distance of a point R on AB from the point A such that RP2+RQ2 is minimum.
View Solutionमान लीजिए बिंदु A और B पर क्रमशः AP तथा BQ दो उर्ध्वाधर स्तंभ है| यदि AP = 16 m, BQ = 22 m और AB = 20 m हों तो AB पर एक ऐसा बिंदु R ज्ञात कीजिए ताकि RP2+RQ2 निम्नतम है |
View Solutionमान लीजिए बिंदु A और B पर क्रमशः AP तथा BQ दो ऊध्वर्रधकर स्तम्भ है । यदि AP =16 मि, BQ =22 मि और AB = 20 मि हो तो AB पर एक ऐसा बिंदु R ज्ञात कीजिए ताकि RP2+RQ2 निम्नतम हो।
View SolutionThe line segments AB and PQ intersect each other at the point C. AP and BQ are perpendiculars on AB respectively. If CA=20cm, CB=8cm and AP=10cm, then find the value of BQ.
View SolutionIf the tangent at the point (a,b) on the curve x3+y3=a3+b3 meets the curve again at the point (p,q) then
1) ab+pq=0 2) bq+ab+ap=0 3) bp+aq+ab=0 4) bq+pq+ap=0
View SolutionLet AP and BQ be two vertical poles at points A and B, respectively. If AP = 16 m, BQ = 22 m and AB = 20 m, then find the distance of a point R on AB from the point A such that RP2+RQ2 is minimum.
View SolutionLet AP and BQ be two vertical poles at points A and B, respectively. If AP = 16 m, BQ = 22 m and AB = 20 m, then find the distance of a point R on AB from the point A such that RP2+RQ2 is minimum.
View SolutionLet AP and BQ be two vertical poles at points A and B, respectively. If AP = 16 m, BQ = 22 m and AB = 20 m, then find the distance of a point R on AB from the point A such that RP2+RQ2 is minimum.
View Solution
