Question

If a circle of radius R passes through the origin O and intersects the coordinate axes at A and B, then the locus of the foot of perpendicular from O on AB is

Options

A. `(x^(2)+y^(2))^(2)=4R^(2)x^(2)y^(2)`
B. `(x^(2)+y^(2))^(3)=4R^(2)x^(2)y^(2)`
C. `(x^(2)+y^(2))(x+y) =R^(2)xy`
D. `(x^(2)+y^(2))^(2)=4Rx^(2)y^(2)`

Answer

B

Explanation

Let the foot of perpendicular be P(h,k). Then, the slope of line ` OP = (k)/(h)`

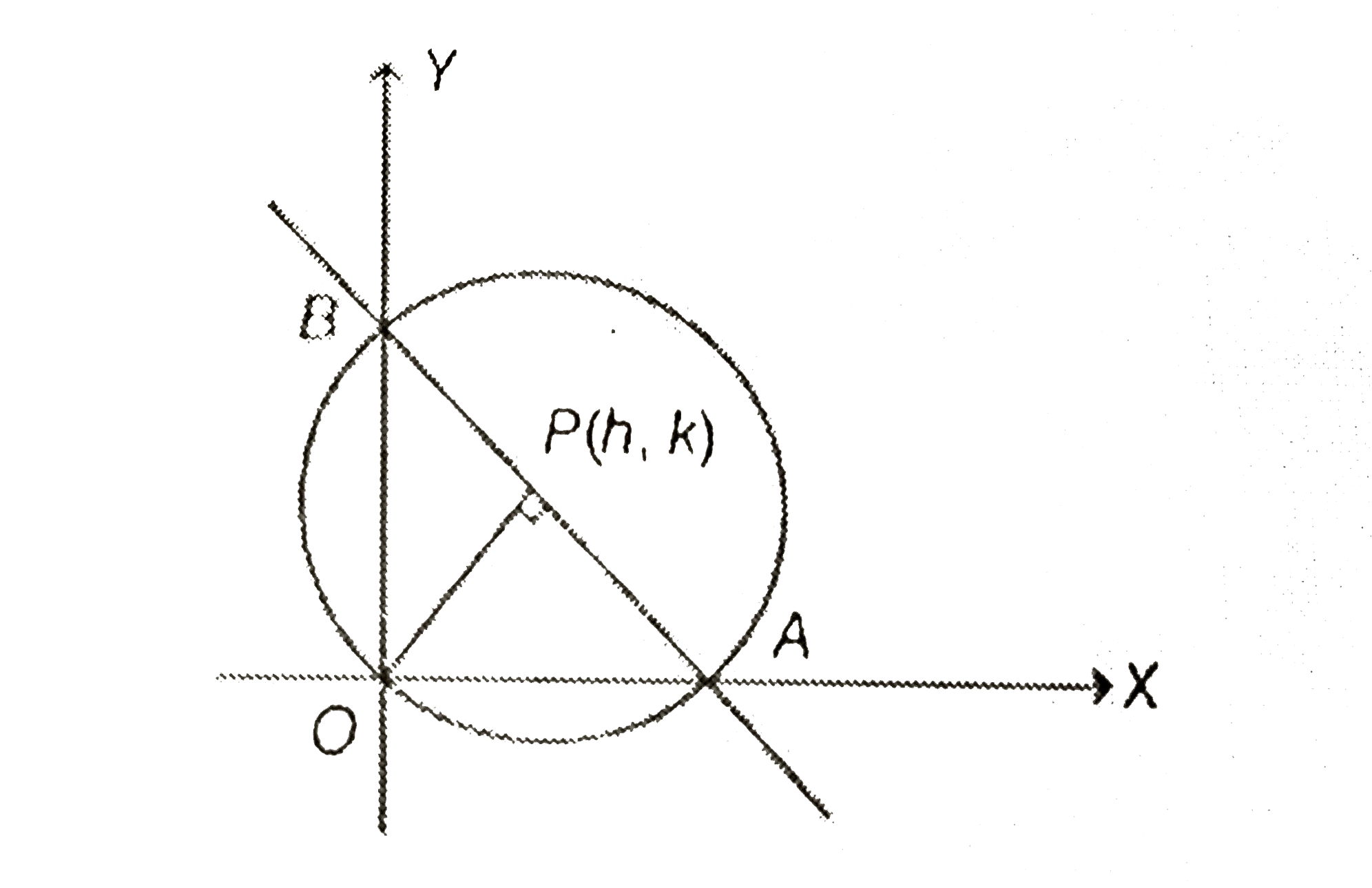
`therefore` Line AB is perpendicular to line OP, so slope of line `AB = -(h)/(k)` [`therefore` product of slope of two perpendicular line s is (-1)]
Now, the equation of line AB is
`y-k=-(h)/(k)(x-h)rArrhx+ky=h^(2)+k^(2)`
or `(x)/(((h^(2)+k^(2))/(h)))+(y)/(((h^(2)+k^(2))/(k)))=1`
So, point `A((h^(2)+k^(2))/(h),0) and B(0,(h^(2)+k^(2))/(h))`
`therefore Delta AOB` is a right angled triangle, so AB is one of the diameter of the circle having radius R (given).
`rArr AB = 2R`
`rArrsqrt(((h^(2)+k^(2))/(k))^(2)+((h^(2)+k^(2))/(k)))=2R`
`rArr (h^(2)+k^(2))^(2)((1)/(h^(2))+(1)/(k^(2)))=4R^(2)`
`rArr (h^(2)+k^(2)) =4R^(2) h^(2)k^(2)`
On replacing h by x and k by y, we get
`(x^(2)+y^(2))^(3)=4R^(2)x^(2)y^(2)`,
which is teh required locus.