Two circles, each of radius 5 units, touch each other at (1, 2). If the equation of their common tangents is $4 x + 3 y = 10$ , find the equations of the circles.

Video Solution

Text Solution

Verified by Experts

The correct Answer is: ${(x - 5)}^{2} + {(y - 5)}^{2} = 5^{2} and {(x + 3)}^{2} + {(y + 1)}^{2} = 5^{2}$

We have,
Slope of the common tangent $= - \frac{4}{3}$
$∴$ Slope of $C_{1} C_{2} = \frac{3}{4}$
If $C_{1} C_{2}$ makes an angle $θ$ with X-axis, then $cos θ = \frac{4}{5} and sin θ = \frac{3}{5}$

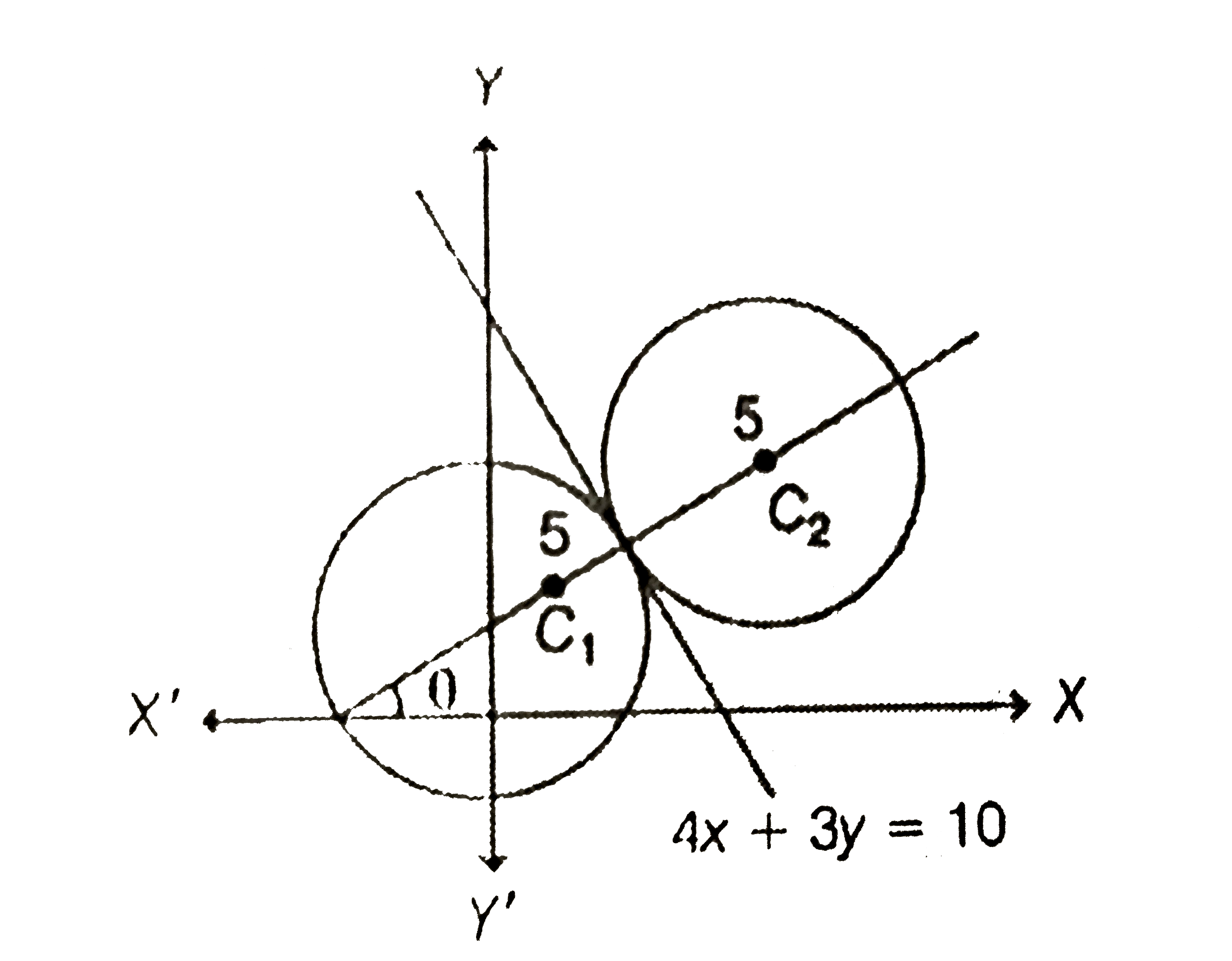
So, the equation of $C_{1} C_{2}$ in parametric form is
$\frac{x - 1}{4 / 5} = \frac{y - 2}{3 / 5} ... (i)$
SInce, $C_{1} and C_{2}$ are points on Eq. (i) at a distance of 5 units from P.
So, the coordinates of $C_{1} and C_{2}$ are given by
$\frac{x - 1}{4 / 5} = \frac{y - 2}{3 / 5} = \pm 5 \Rightarrow x = 1 \pm 4$
and $y = 2 \pm 3$ .
Thus, the coordinates of $C_{1} and C_{2}$ are (5, 5) and (-3, -1), respectively.
Hence, the equations of the two circles are
${(x - 5)}^{2} + {(y - 5)}^{2} = 5^{2}$
and ${(x + 3)}^{2} + {(y + 1)}^{2} = 5^{2}$

|

Updated on:21/07/2023

Class 12 MATHS CIRCLE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Two circles each of radius 5 units touch each other at (1, 2). If the equation of their common tangent is $4 x + 3 y = 10$ , then the centres of the two circles are
A(3,4),(-1,0)
B(5,7),(-3,-3)
C(5,5),(-3,-1)
Dnone of these
Question 2 - Select One
Two circles of radii 8 cm and 6 cm touch each other externally. The length of the direct common tangent is:
8 सेमी तथा 6 सेमी त्रिज्याओं वाले दो वृत्त एक-दूसरे को बाहर से स्पर्श करते हैं | प्रत्यक्ष उभयनिष्ठ स्पर्श रेखा की लंबाई कितनी है ?
A20
B24
C $13.86$
D $10.12$
Question 3 - Select One
Two circles, each of radius 5, have a common tangent at (1, 1) whose equation is $3 x + 4 y - 7 = 0$ . Then their centres, are
A(4, -5), (-2, 3)
B(4, -3), (-2, 5)
C(4, 5), (-2, -3)
Dnone of these

IIT JEE PREVIOUS YEAR-CIRCLE-Topic 3 Analytical & Descriptive Questions

Home
Ask Doubt
Profile