In Fig 16-46, a sinusoidal wave moving along a string is shown twice as crest A travels in the positive direction of any x axis by distance d=6.0 cm in 3.0 ms. The tick marks along the axis are separated by 10cm, height H=6.00MM. The equation for the wave is in the form y(x,t) =ymsin(kx±ωt). so what are (a) ym,(b)k,(c)ω and (d) the correct choice of sign in front of ω? 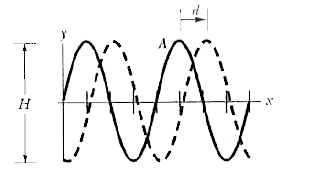
The correct Answer is:(a) 3.0 mm; (b) 16 rad/m; (c) 3.2×102 rad/s; (d) minus sign
Topper's Solved these Questions
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (Single Correct Choice Type)
33 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Practice Questions (More than One Coorect Choice Type)
10 Videos
- WAVES-IBOOK - RESNICK AND HALLIDAYCHAPTER - WAVES-IEXERCISE - Checkpoint
8 Videos
- WAVE - IIBOOK - RESNICK AND HALLIDAYCHAPTER - WAVE - IIEXERCISE - PRACTICE QUESTIONS (INTEGER TYPE)
5 Videos
- WORK, POWER, AND ENERGYBOOK - RESNICK AND HALLIDAYCHAPTER - WORK, POWER, AND ENERGYEXERCISE - PRACTICE QUESTIONS (Integer Type)
5 Videos
Similar Questions
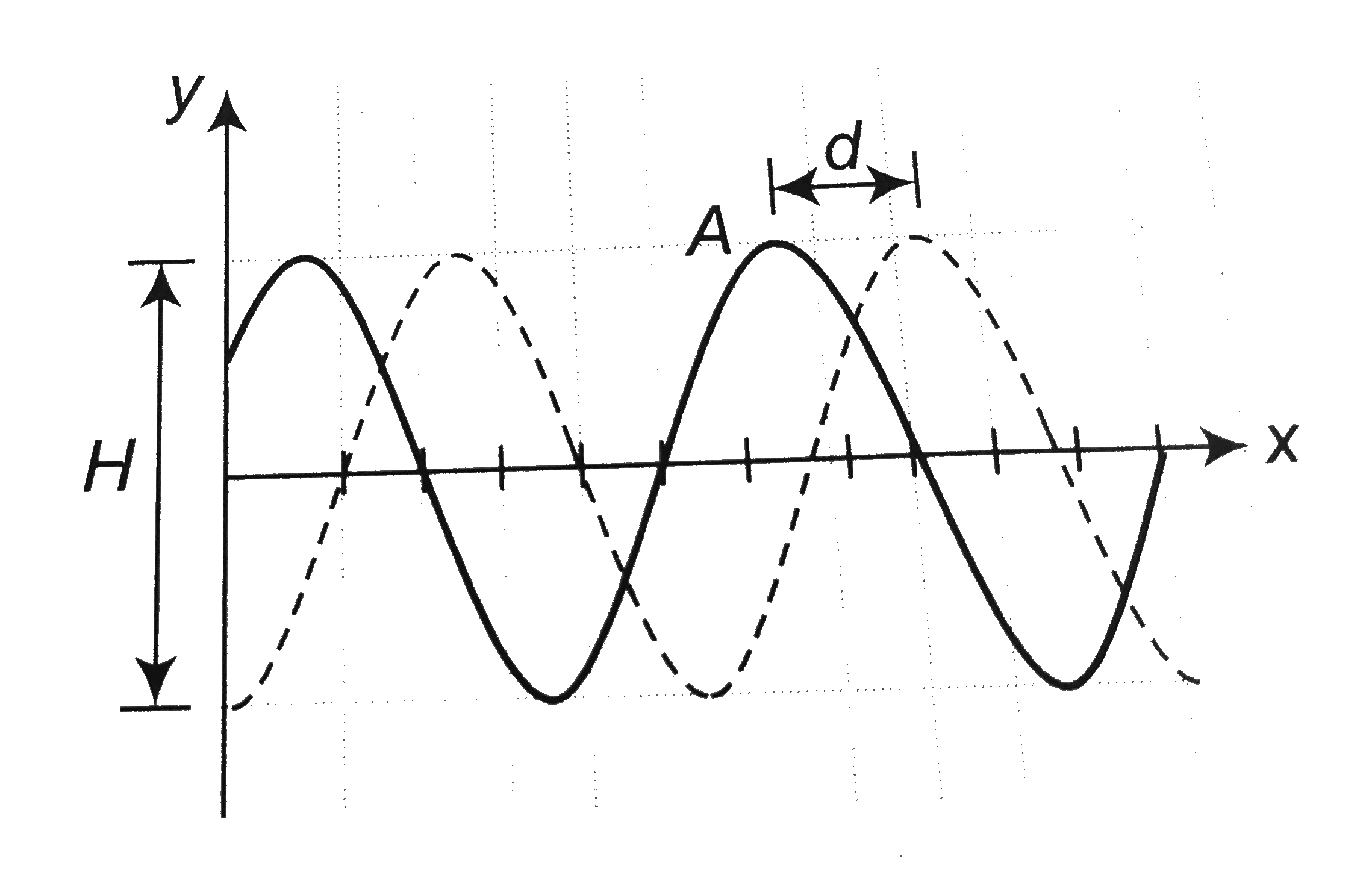
A sinusoidal wave moving along a string is shown twice in the figure. As crest A travels in the positive direction of an x axis by distance d=6.0 cm in 4.0ms. The tick marks along the axis are separated by 10cm, height H=6.00mm. The wave equation is
View SolutionTwo sinusoidal waves with the same amplitude of 6.00 mm and the same wavelength travel together along a string that is stretched along an x axis. Their resultant wave is shown twice in Fig. 16 39. as velley travels in the negative direction of the x axis by distance d= 56.0 cm in 8.0 ms. The lick matks along the axis are separated by 10cm, and height H is 8.0mm. Let the equation for one wive be of the form y(x,t) =ymsin(kx±ωt+ϕ1), where ϕ1=0 and you must choose the correct sign in front of ω. For the equation for the other wave, what are (a) ym,(b)k,(c)ω,(d)ϕ2, and (e) the sign in front of ω?
View Solution
Knowledge Check

A sinusoidal wave moving along a string is shown twice in the figure. As crest A travels in the positive direction of an x axis by distance d=6.0 cm in 4.0m. The tick marks along the axis are separated by 10cm, height H=6.00mm. The wave equation isAy=(3mm)sin[16x−2.4×102t]By=(3mm)sin[16x+2.4×102t]Cy=(3mm)sin[8x+2.4×102t]Dy=(3mm)sin[8x−2.4×102t]The amplitude of a wave produced in a string is 5 cm. The wave is moving along positive direction of X-axis with a speed of 150 m/s and 5 vibrations are completed in 3 m length of the string. The equation representing the wave will be
A0.05sin(5t−100t)B0.05sin(10.4x−1571t)C0.09sin(10.9x−2000t)D0.05sin(9x−1000t)
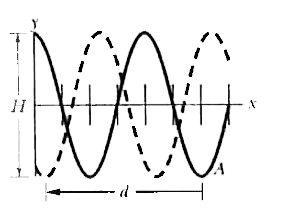
Two sinusoidal waves with the same amplitude and wavelength travel through each other along a string that is stretched along an x axis. Their resultant wave is shown twice in Fig. 16-53, as the antinode A travels from an extreme upward displacement to an extreme downward displacement in 6.0 ms. The tick marks along the axis are separated by 15 cm, height H is 1.20 cm. Let the equation for one of the two waves be of the form y(x,t)=ymsin(kx+ω). In the equation for the other wave, what are (a)ym(b)k,(c)ω, and (d) the sign in front of ω?

View SolutionA stretched string has a mass per unit length of 5.00 g/cm and a tension of 10.0 N A sinusoidal wave on this string has an amplitude of 0.16 mm and a frequency of 100 Hz and is traveling in the negative direction of an x axis. If the wave equation is of the form y(x,t)= ymsin(kx±ωt), what are (a) ym (b) k. (c) ω, and (d) the correct choice of sign in front of ω?
View SolutionTwo waves are generated on a string of length 4.0 m to produce a three-loop standing wave with an amplitude of 1.0 cm.The wave speed is 100 m/s. Let the equation for one of the waves be of the form y(x,t)=ymsin(kx+ωt). In the equation for the other wave, what are (a) ym (b) k, (c) ω and (d) the sign in front of w!
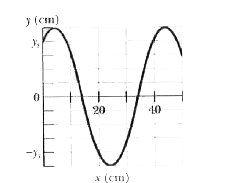
View SolutionA sinusoidal transverse wave is traveling along a string in the negative direction of an x axis. Figure 16-S1 shows a plot of the displacement as a function of position at time t = 0, the scale of the y axis is set by y = 4.0 cm. The string tension is 3.6 N, and its linear density is 28 g/m. Find the (a) amplitude, (b) wavelength, (e) wave speed, and (d) period of the wave (e) Find the maximum transverse speed of a particle in the string. If the wave is of the form y(x,t)=ymsin(kx±ωt+ϕ), what are (f) k, (g) ω(h)ϕ, and (i) the correct choice of sign in front of ω?
View SolutionA transverse sinusoidal wave is moving along a string in the opposite direction of an x axis with a speed of 70m/s. At t=0 the string particle at x=0 has a transverse displacement of 4.0 cm and is not moving. The maximum transverse speed of the string particle at x=0 is 16m/s. (a) What is the frequency of the wave? (b) What is the wavelength of the wave? If y(x,t) =ymsin(kx±ωt+ϕ) is the form of the wave equation, what are (c) ym,(d)k,(e)ω(f)ϕ, and (g) the correct of sign in front of ω?
View SolutionA sinusoidal transverse wave of wavelength 18 cm travels along a string in the positive direction of an x axis. The displacement y of the string particle at x= 0) is given in Fig. 16-41 as a function of time t. The scale of the vertical axis is set by y1=4.0cm, The wave equation is to be in the form y(x,t)=ynIsin(kx±ωt+ϕ). (a) At t= 0), is a plot of y versus x in the shape of a positive sine function on a legative sinc function? What are (b)ynI(c)k,(d)ω(e)ϕ, (f) the sign in front of ω, and (g) the speed of the wave? (h) What is the transverse velocity of the particle at x=0 when t=5.0 s?
View Solution
