An electron falls throgh a distance of 1.5 cm in a uniform electric field of magnitude 2.0×104Nc−1 the direction of the field is reversed keeping its magnitude unchanged and a proton falls through the same distance compute the time of falls in each case contrast the situation with that of free fall under gravity
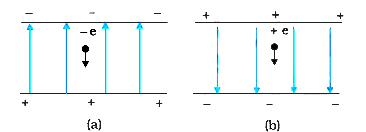
The field is upward so the negatively charged the magnitude of the electric of the electron is ae=eE/me
where me is the mas of the electron
distance h is given by te=√2hae=√2hmeeE
For e=1.6×10−19C,me=9.11×10−31 kg
te=2.9n×10−9 S
where mp is the mass of the proton mp=1.67×10−27 kg the time of fall for the proton is
tp=√2hap=√2hmpeE=1.3×10−7 S
ap=eEmp
=1.9×1012ms−2
which is enormous compared to the value of g (9.8 ms−2) the acceleration due to gravity the thus the effect of acceleration due to gravity can be ignored in this example
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDS BOOK - NCERT BANGLISHCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - EXERCISES
29 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERT BANGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - EXERCISES
41 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT BANGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - ADDITIONAL EXERCISES
7 Videos
Similar Questions
An electron falls through a distance of 1.5 cm in a uniform electric field of magnitude 2.4×104NC−1 [Fig.1.12 (a)]. The direction of the field is reversed keeping its magnitude unchagned and a proton falls through the same distance [Fig. 1.12 (b) ]. Complute the time of fall in each case. Contrast the situation (a) with that of free fall under gravity.
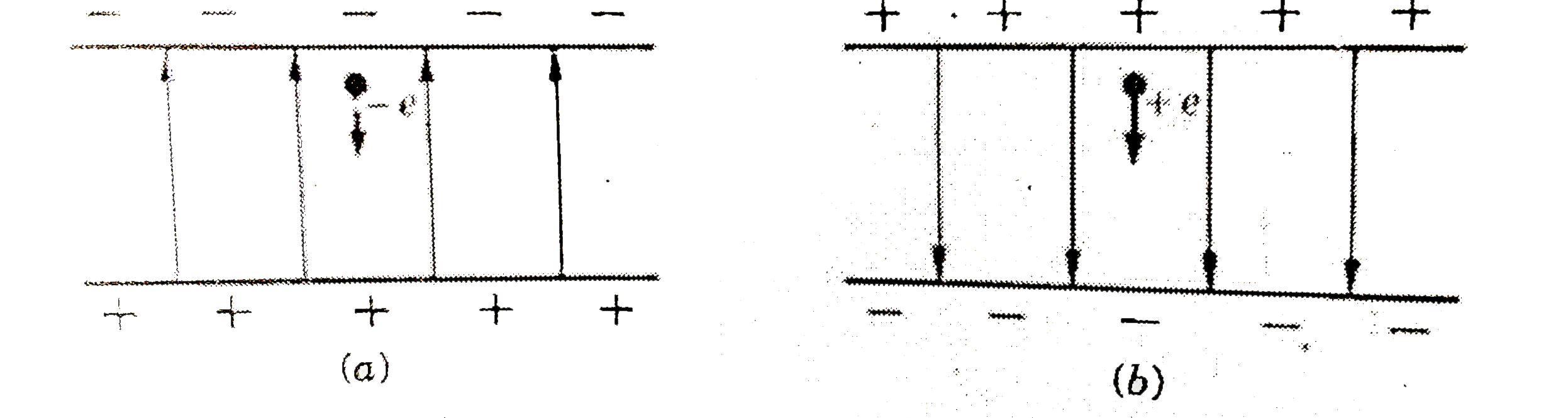
View SolutionAn electron falls through a distance of 1.5 cm in a uniform electric field of magnitude 2.4×104NC−1 [Fig.1.12 (a)]. The direction of the field is reversed keeping its magnitude unchagned and a proton falls through the same distance [Fig. 1.12 (b) ]. Complute the time of fall in each case. Contrast the situation (a) with that of free fall under gravity.

View Solution
Knowledge Check
An electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
ASame in both casesBMore in the case of electronCMore in the case of protonDIndependent of chargeAn electron initially at rest falls a distance of 1.5 cm in a uniform electric field of magnitude 2×104N/C. The time taken by the electron to fall this distance is
A1.3×102sB2.1×10−12sC1.6×10−10sD2.9×10−9sAn electron initially at rest falls a distance of 1.5 cm in a uniform electric field of magnitude 2×104N/C. The time taken by the electron to fall this distance is
A1.3×102sB2.1×10−2sC1.6×10−10sD2.9×10−9s
An electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
View SolutionAn electron falls through a small distance in a uniform electric field of magnitude 2×104NC−1. The direction of the field reversed keeping the magnitude unchanged and a proton falls through the same distance. The time of fall will be
View SolutionAn electron falls through a distance of 1.5cm in a uniform electric field of value 2×104N/C, opposite to direction of fall. Compare the time of fall with 'free fall under gravity'.
View SolutionAn electron initially at rest falls a distance of 1.5 cm in a uniform electric field of magnitude 2×104N/C. The time taken by the electron to fall this distance is
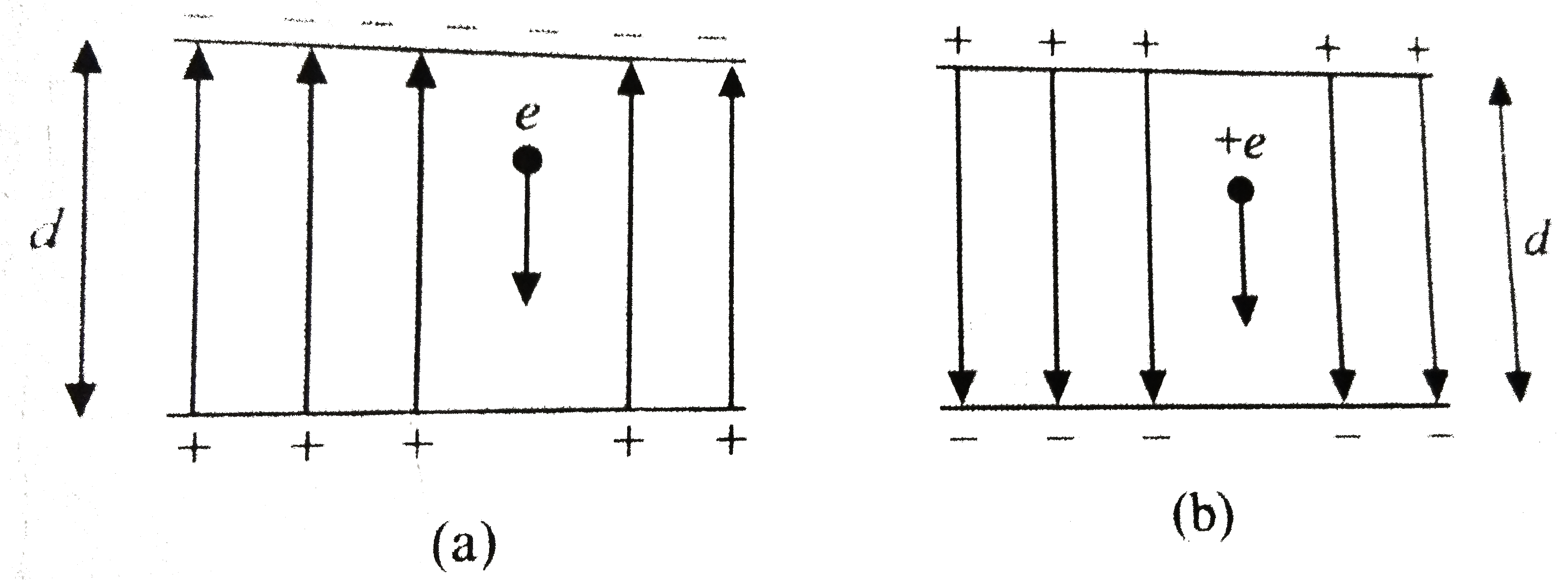
View SolutionAn electron (mass me)falls through a distance d in a uniform electric field of magnitude E.
 ,
,
The direction of the field is reversed keeping its magnitudes unchanged, and a proton(mass mp) falls through the same distance. If the times taken by the electrons and the protons to fall the distance d is telectron and tproton respectively, then the ratio telectron/tproton.
View Solution
