Question

A circle circumscribing an equilateral triangle with centroid at $(0,0)$ of a side a isdrawn and a square is drawn touching its four sides to circle. The equation ofcircle circumscribing the square is :

Options

A. `x^(2)+y^(2)=2a^(2)`
B. `3x^(2)+3y^(2)=2a^(2)`
C. `5x^(2)+5y^(2)=3a^(2)`
D. none of these

Answer

B

Explanation

Clearly, centre of the circumcircle is at the origin and radius is two-third of the altitude `(sqrt(3))/(2)` a. So, the equation of the circumcircle is
`x^(2)+y^(2)=((a)/(sqrt(3)))^(2)`

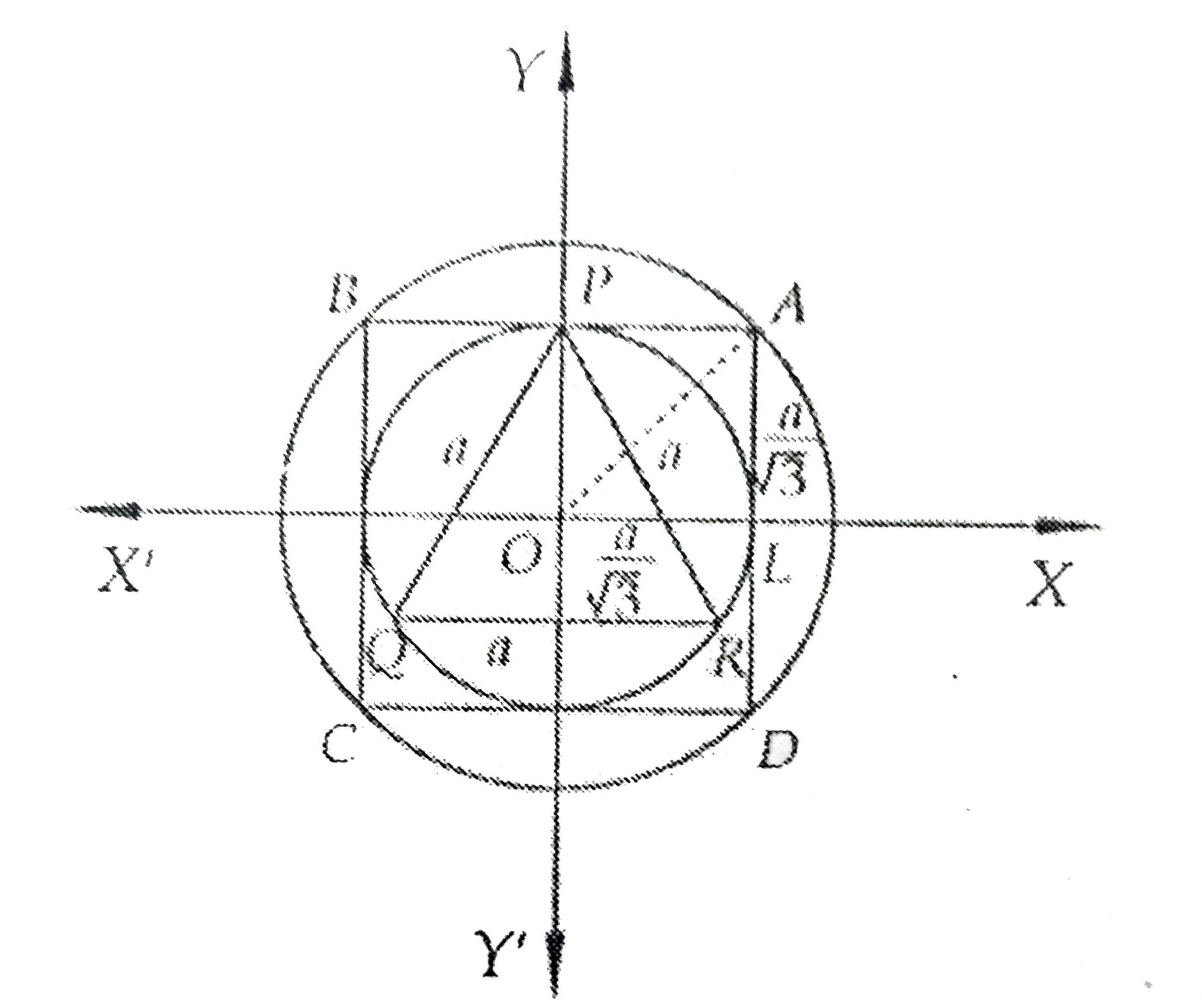
Clearly, Radius of the circumcircle of the square ABCD
`=OA=sqrt(OL^(2)+AL^(2))=sqrt((a^(2))/(3)+(a^(2))/(3))=sqrt((2)/(3))a`
So, the equation of the circumcircle is
`x^(2)+y^(2)=(2)/(3)a^(2)rArr 3 (x^(2)+y^(2))=2a^(2)`