Define the term corner point of a feasible region in an LPP. Solution in Kannada
Step by step video solution for Define the term corner point of a feasible region in an LPP. by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - OSWAAL PUBLICATIONCHAPTER - LINEAR PROGRAMMING EXERCISE - Long Answer Type Questions-lI26videos
LINEAR PROGRAMMING
- BOOK - OSWAAL PUBLICATIONCHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - SHORT ANSWER TYPE QUESTIONS - II43videos
INVERSE TRIGONOMETRIC FUNCTIONS
- BOOK - OSWAAL PUBLICATIONCHAPTER - MATRICES EXERCISE - ELEMENTARY OPERATIONS OR TRANSFORMATION OF A MATRIX ( Long Answer Type Questions- II )3videos
MATRICES
Similar Questions
(Allocation problem) A cooperative society of farmers has 50 hectare of land to grow two crops X and Y. The profit from crops X and Y per hectare are estimated as Rs 10,500 and Rs 9,000 respectively. To control weeds, a liquid herbicide has to be
00:35View SolutionSolve : tan−1(x−1)x−2+tan−1(x+1)x+2=π4
01:14View Solution⎡⎢⎣−112123311⎤⎥⎦
01:11View SolutionThe feasible region for an LPP is always a..polygon
01:24View SolutionMaximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
02:06View SolutionIn a LPP, the maximum value of the objective function Z = ax +by is always 0, if origin is one of the corner point of the feasible region.
03:01View SolutionDefine the term Feasible Solution in a LPP.
09:17View SolutionDefine the term corner point of a feasible region in an LPP.
00:58View SolutionDefine a corner point of a feasible region.
02:22View SolutionThe feasible region for a LPP is shown in the following figure. Evaluate Z=4x+y at each of the corner points of the region. Find the minimum value of Z, if it exists
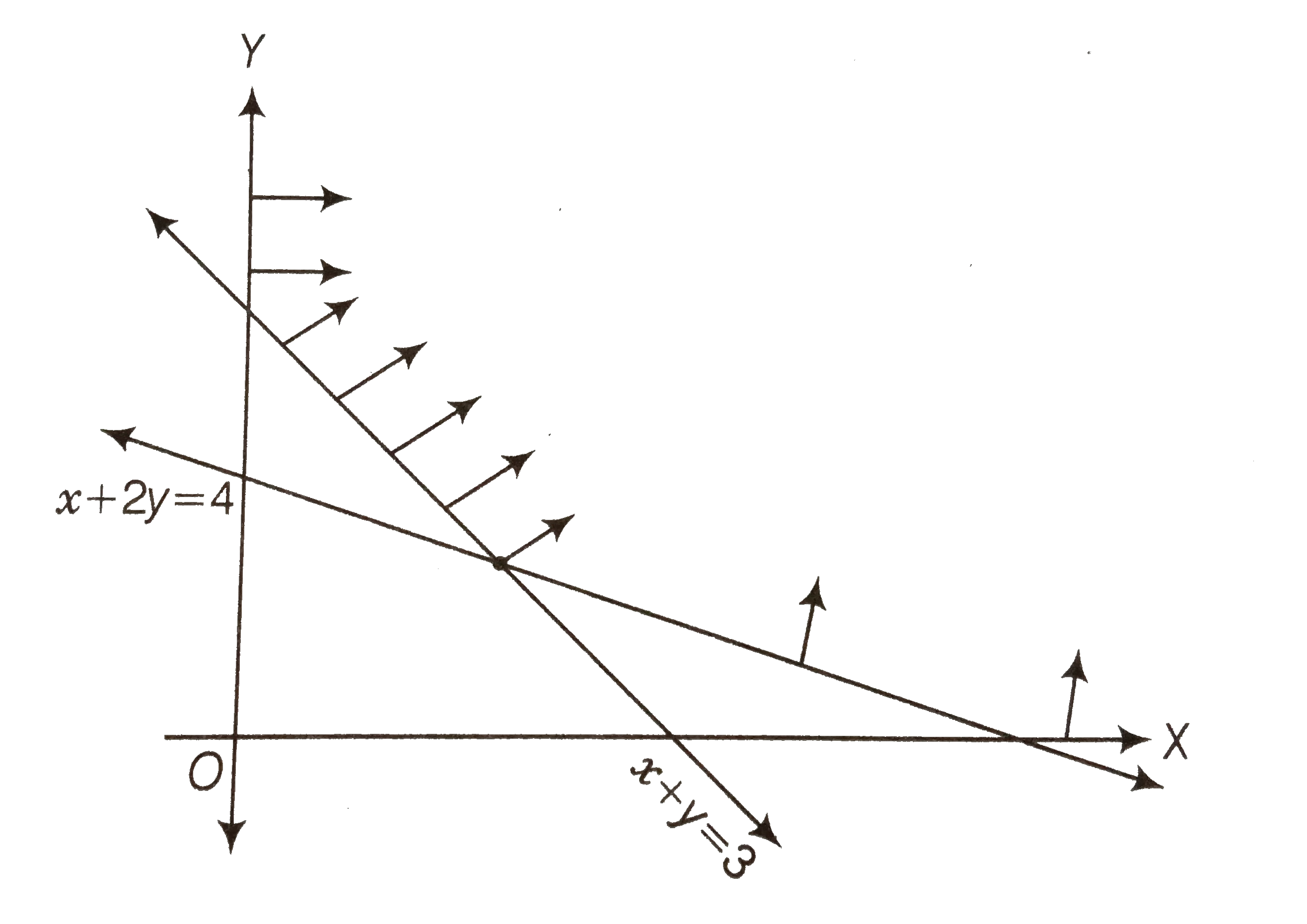 01:50View Solution
01:50View SolutionThe feasible region for an LPP is always a..polygon
03:50View SolutionMaximum value of the objective function Z = ax +by in a LPP always occurs at only one corner point of the feasible region.
02:08View SolutionIn a LPP, the maximum value of the objective function Z = ax +by is always 0, if origin is one of the corner point of the feasible region.
02:20View SolutionDefine Feasible region in LPP.
View SolutionMaximum value of the objective function Z = ax + by in a LPP always occurs at only one corner point of the feasible region.
03:11View SolutionIn a LPP, the minimum value of the objective function Z = ax + by is always 0, if origin is one of the corner point of the feasible region.
03:00View Solution

