A uniform rope of length 12m and mass 6kg hangs vertically from a rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse whwn it reaches the top of the rope?
To solve the problem, we need to determine the wavelength of a transverse pulse when it reaches the top of a vertically hanging rope. Let's break down the solution step by step:
Step 1: Understand the system
We have a rope of length 12 m and mass 6 kg hanging vertically. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse with a wavelength of 0.06 m is generated at the lower end of the rope.
Step 2: Calculate the tension at the lower end of the rope
At the lower end of the rope, the only force acting on the rope is the weight of the block attached to it. The tension (T1) at this point is given by:
T1=mblock⋅g
Where:
- mblock=2kg
- g=9.8m/s2
Calculating the tension:
T1=2kg⋅9.8m/s2=19.6N
Step 3: Calculate the mass per unit length (μ) of the rope
The mass per unit length (μ) of the rope can be calculated as:
μ=mropeL
Where:
- mrope=6kg
- L=12m
Calculating μ:
μ=6kg12m=0.5kg/m
Step 4: Calculate the tension at the top of the rope
At the top of the rope, the tension (T2) is the sum of the weight of the block and the weight of the entire rope:
T2=mblock⋅g+mrope⋅g
Calculating T2:
T2=(2kg+6kg)⋅9.8m/s2=8kg⋅9.8m/s2=78.4N
Step 5: Calculate the velocities at the lower and upper ends of the rope
The velocity of a wave on a string is given by:
V=√Tμ
Calculating the velocity at the lower end (V1):
V1=√T1μ=√19.6N0.5kg/m=√39.2≈6.26m/s
Calculating the velocity at the upper end (V2):
V2=√T2μ=√78.4N0.5kg/m=√156.8≈12.52m/s
Step 6: Relate the velocities and wavelengths
Since the frequency (f) of the wave remains constant, we can use the relationship:
V=f⋅λ
Thus, the ratio of the velocities is equal to the ratio of the wavelengths:
V1V2=λ1λ2
Substituting the values:
6.2612.52=0.06λ2
Step 7: Solve for the new wavelength (λ2)
Cross-multiplying gives:
6.26⋅λ2=0.06⋅12.52
Calculating:
λ2=0.06⋅12.526.26≈0.12m
Final Answer
The wavelength of the pulse when it reaches the top of the rope is approximately 0.12 m.
---
We have a rope of length 12 m and mass 6 kg hanging vertically. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse with a wavelength of 0.06 m is generated at the lower end of the rope.
At the lower end of the rope, the only force acting on the rope is the weight of the block attached to it. The tension (T1) at this point is given by:
T1=mblock⋅g
Where:
- mblock=2kg
- g=9.8m/s2
T1=2kg⋅9.8m/s2=19.6N
The mass per unit length (μ) of the rope can be calculated as:
μ=mropeL
Where:
- mrope=6kg
- L=12m
μ=6kg12m=0.5kg/m
At the top of the rope, the tension (T2) is the sum of the weight of the block and the weight of the entire rope:
T2=mblock⋅g+mrope⋅g
Calculating T2:
T2=(2kg+6kg)⋅9.8m/s2=8kg⋅9.8m/s2=78.4N
The velocity of a wave on a string is given by:
V=√Tμ
V1=√T1μ=√19.6N0.5kg/m=√39.2≈6.26m/s
V2=√T2μ=√78.4N0.5kg/m=√156.8≈12.52m/s
Since the frequency (f) of the wave remains constant, we can use the relationship:
V=f⋅λ
Thus, the ratio of the velocities is equal to the ratio of the wavelengths:
V1V2=λ1λ2
6.2612.52=0.06λ2
Cross-multiplying gives:
6.26⋅λ2=0.06⋅12.52
Calculating:
λ2=0.06⋅12.526.26≈0.12m
The wavelength of the pulse when it reaches the top of the rope is approximately 0.12 m.
Topper's Solved these Questions
Similar Questions
A uniform rope of length 12m and mass 6kg hangs vertically from a rigid support . A block of mass 2kg is attached to the free end of the rope . A transverse pulse of wavelengths 0.06m is produced at the lower end of the rope . What is the wavelength of the pulse when it reaches the top of the rope?
View SolutionA uniform rope of length 12 mm and mass 6 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top of the rope?
View Solution
Knowledge Check
A uniform rope of length 10 m and mass 3 kg hangs vertically from a rigid support. A block of mass 1 kg is attached to the free end of the rople. A transverse pulse of wavelength 0.05 m is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is
A0.10 mB0.12 mC0.16 mD0.18 mA uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1+m2m1B√m1m2C√m1+m2m2D√m2m1A uniform rope of legnth L and mass m1 hangs vertically from a rigid support. A block of mass m2 is attached to the free end of the rope. A transverse pulse of wavelength λ1 is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is λ2. The ratio λ2λ1 is
A√m1m2B√m1+m2m2C√m2m1D√m1+m2m1
A uniform rope of length 20m and mass 8kg hangs vertically froma rigid support. A block of mass 2kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06m is produced at the lower end of the rope. What is the wavelength of the pulse when it reaches the top os the rope?
View SolutionA uniform rope of length 10 cm and mass 4 kg hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.06 m is produced at the lower end of the rope, what is the wavelength of the pulse when it reaches the top of the rope?
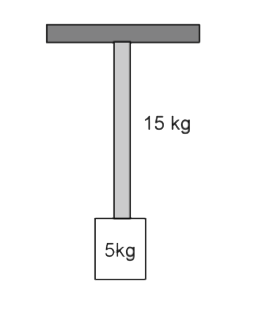
View SolutionA uniform rope of length 10 m and mass 15 kg hangs vertically from a rigid support. A block of mass 5 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.08 m is produced at the lower end of the 3 rope. The wavelength of the pulse when it reaches the top of the rope will be-
View SolutionA uniform rope of elngth l and mass m hangs vertically from a rigid support. A block of mass m is attached to the free end of the rope. A transverse pulse of wavelength λ is produced at the lower end of the rope. The wavelength of the pulse, when it reaches the top of the rope. is aλ. Find a2

View SolutionA uniform rope of mass 6 kg and length 6 m hangs vertically from a rigid support. A block of mass 2 kg is attached to the free end of the rope. A transverse pulse of wavelength 0.075 m is produced at the lower end of the rope. The wavelength of the pulse when it reaches the top of the rope is K cm where K is
View Solution
