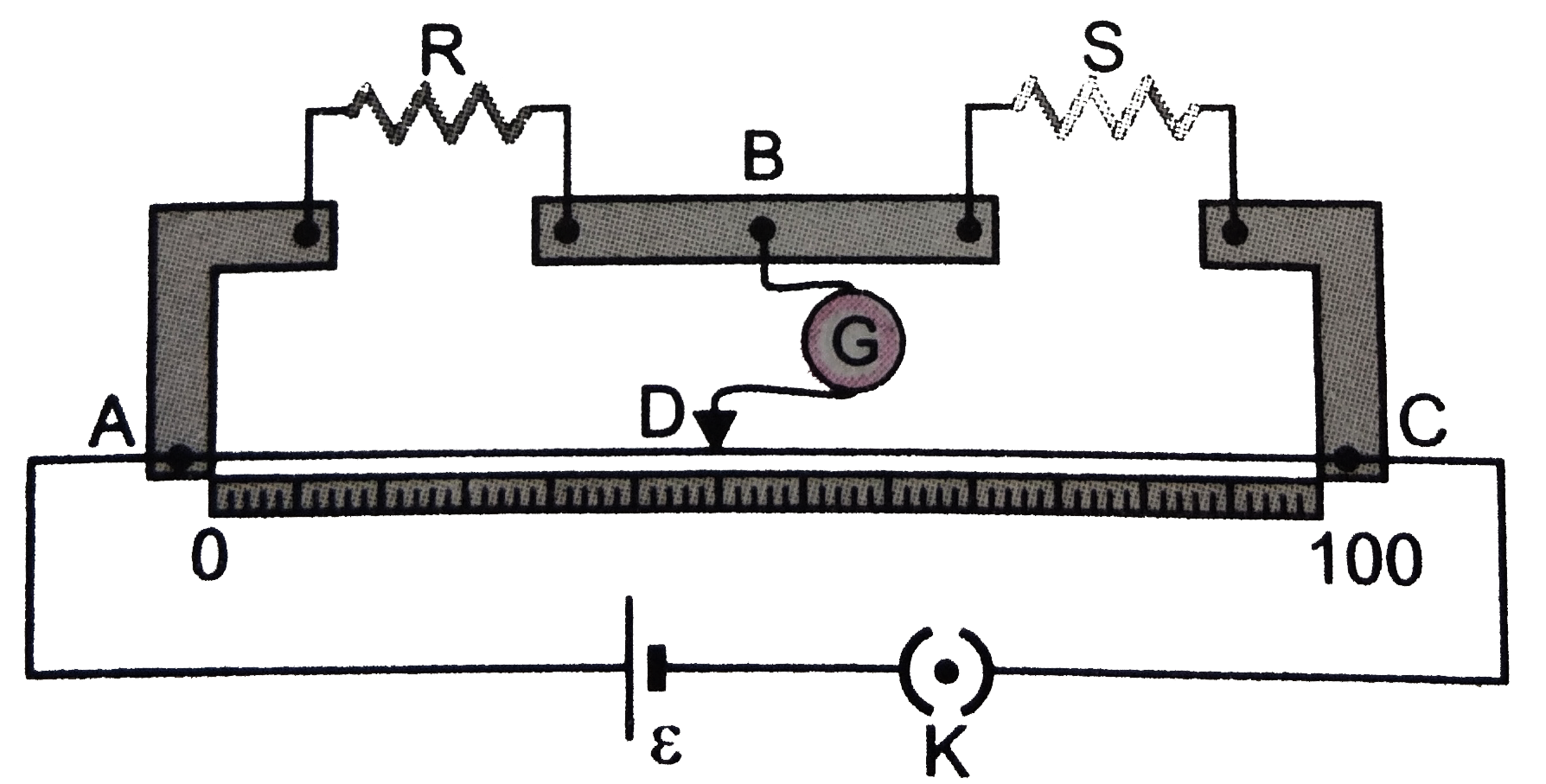
(i) State the principle of working of a meter bridge.
(ii) In a meter bridge balance point is found at a distance I1 with resistances R and S as shown in the figure. When an unknown resistance X is connected in parallel with the resistance S, the balance point shifts to a distance I2. Find the expression for X in terms of l1,l2 and S.
(i) A slide wire bridge is known as Metre bridge. It is constructed on the principle of balanced wheatstone bridge. When a Wheaststone bridge is balanced then,
PQ=l100−l
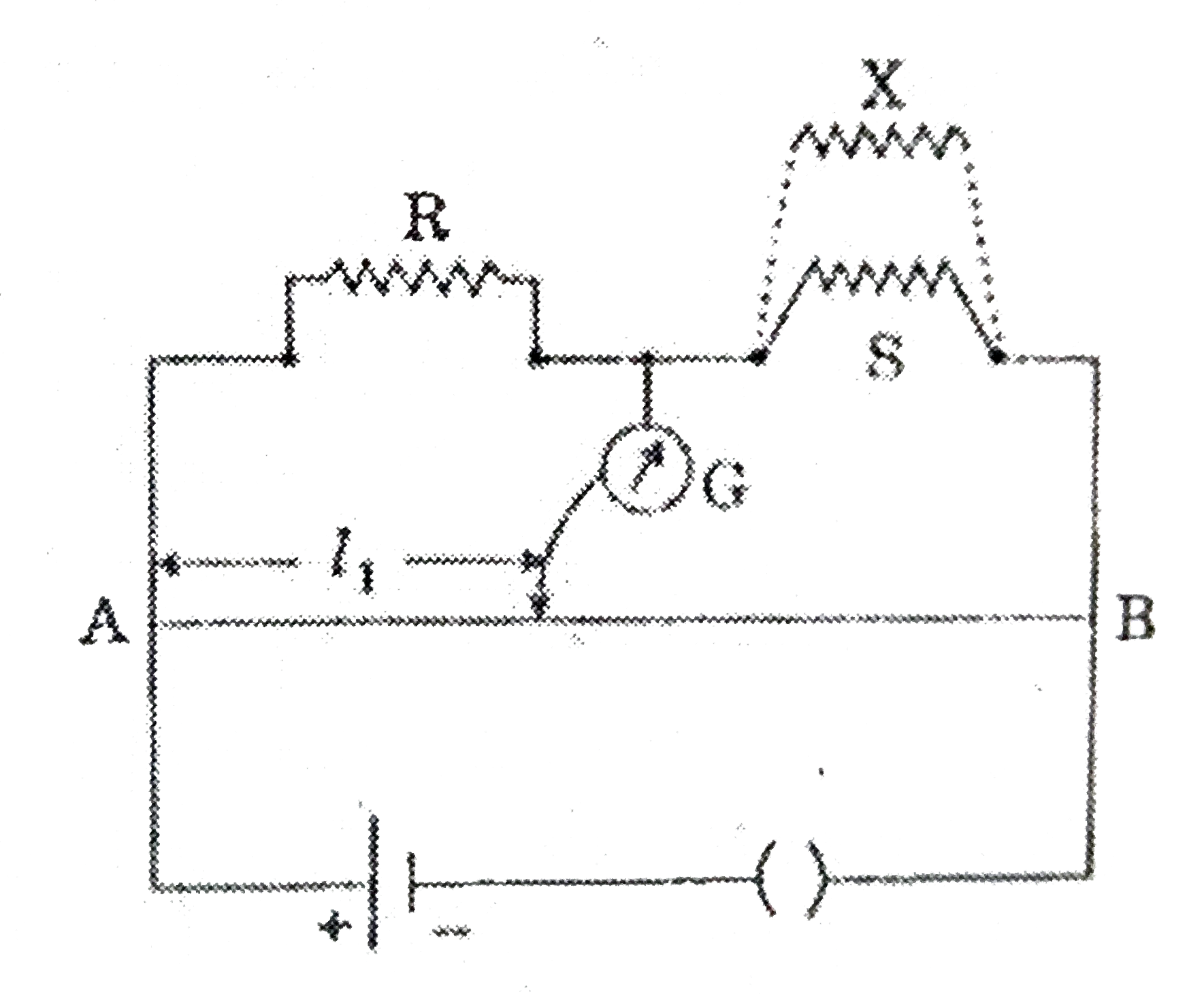
(ii) When resistace R and S are connected : Since, balance point is found at a distance l1 from the zero and
∴RS=l1100−l1 ...(i)
When unknown resistance X is connected in parallel to S.
∴ Total resistance in the right hand gap is S1=SXS+X [:]1R=1R1+1R2⇒R=R1R2R1+R2]
Since, balance point is obtained at a distance l2 from the zero end
∴RS1=l2100−l2
Putting the value of S1 we get
RSXS+X=l2100−l2
R(S+X)SX=l2100−l2 ...(ii)
Dividing (ii) by (i), we get
R(S+X)SX.SR=l2100−l2×100−l1l1 , S+XX=l2(100−l1)l1(100−l2)
SX+XX=l2(100−l1)l1(100−l2) ⇒ SX+1=l2(100−l1)l1(100−l2)
SX+XX=l2(100−l1)l1(100−l2) ⇒ SX+1=l2(100−l1)l1(100−l2)
SX=l2(100−l1)l1(100−l2)−1
=100l2−l1l2−100l1+l1l2l1(100−l2), SX=100(l2−l1)l1(100−l2)
Hence, X=l1(100−l2)100(l2−l1).S ∴ X=100l1s−l1l2s100(l2−l1)
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET-II
35 Videos
- XII BOARDSBOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET-III
25 Videos
- XII BOARDSBOOK - XII BOARDS PREVIOUS YEARCHAPTER - XII BOARDSEXERCISE - SET III
9 Videos
- SAMPLE PAPER 2019BOOK - XII BOARDS PREVIOUS YEARCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
(i) State the principle of working of a metre bridge.
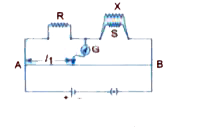
(ii) In a metre bridge balance point is found at a distance l1 with resistances R and S as shown in the figure.
When an unknown resistance X is connected in parallel with the resistance S, the balance point shifts to a distance l. Find the expression for X in terms of l1,l2 and S.
View SolutionAnswer the following:
(a) Write the principle of working of a metre bridge.
(b) In a metre bridge, the balance point is found at a distance I1 with resistances R and S as shown in the figure.

An unknown resistance X is now connected in parallel to the resistance S and the balance point is found at a distance I2 , Obtain a formula for X in terms of I1,I2 and S .
View Solution
Knowledge Check
In a meter bridge experiment, resistances are connected as shown in figure. The balancing length l1=55cm. Now, an unknown resistance x is connected in series with P and the new balancing length is found to be 75 cm. What is the value of The value of 11x (in ohm)? (Given, P=4Ω)
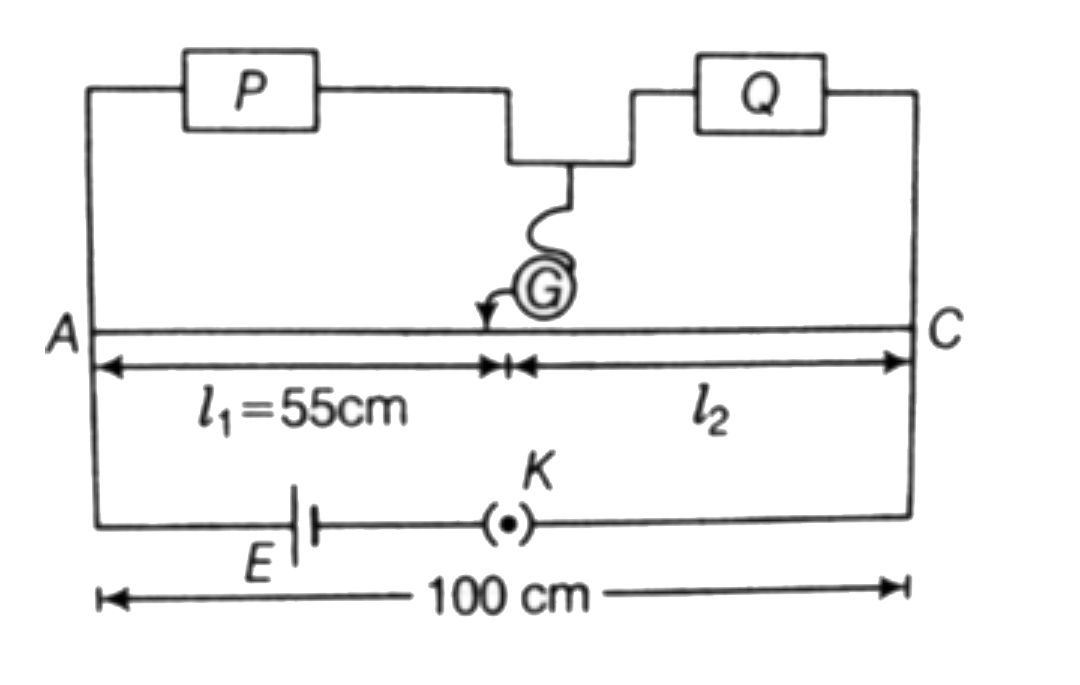 A5413ΩB2011ΩC4811ΩD1148Ω
A5413ΩB2011ΩC4811ΩD1148ΩResistance in the two gaps of a meter bridge are 10ohm and 30ohm respectively. If the resistances are interchanged the balance point shifts by
A33.3cmB66.67cmC25cmD50cm
When two known resistance R and S are connected in the left and right gaps of a meter bridge, the balance point is found at a distance l1 from the zero end of the meter bridge wire. An unknown resistance X is now connected in parallel to the resistance S and the balance point is found at a distance l2 from the zero end of the meter bridge wire,
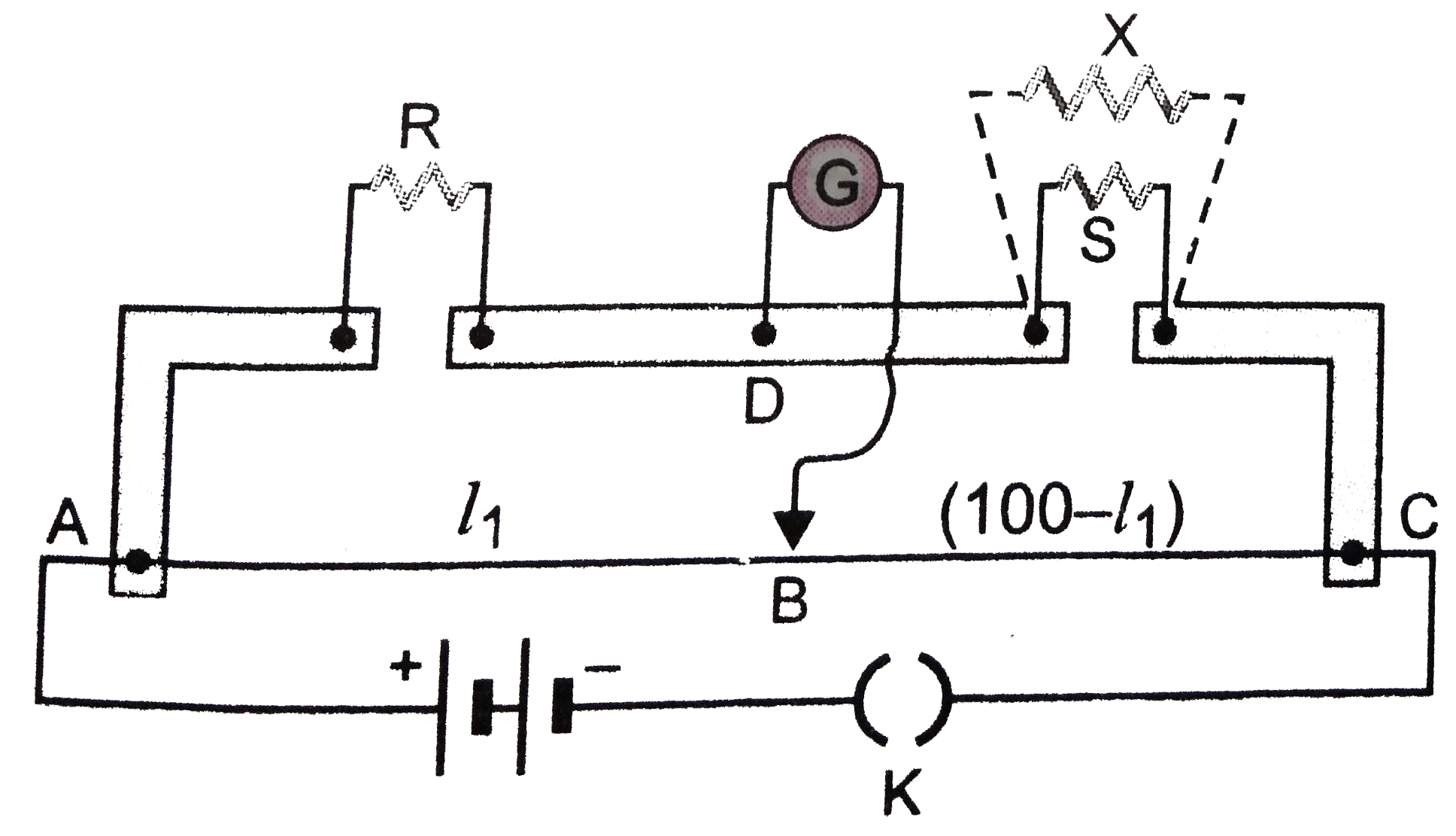
Obtain a formula for X in terms of l1,l2 and S.
View SolutionIn a meter bridge,
the null points is found at a distance of 33.7 cm from A. If now a resistance of 12Ω is connected in parallel with S, the null point occurs at 51.9 cm. Determine the values of R and S.
View SolutionIn a meter bridge,

the null points is found at a distance of 33.7 cm from A. If now a resistance of 12Ω is connected in parallel with S, the null point occurs at 51.9 cm. Determine the values of R and S.
View SolutionIn a meter bridge, the null point is found at a distance of l1cm from A. if now a resistance of X is connected in parallel with S, the null point occurs at l2cm. Obtain a formula for X is terms of l1,l2andS.
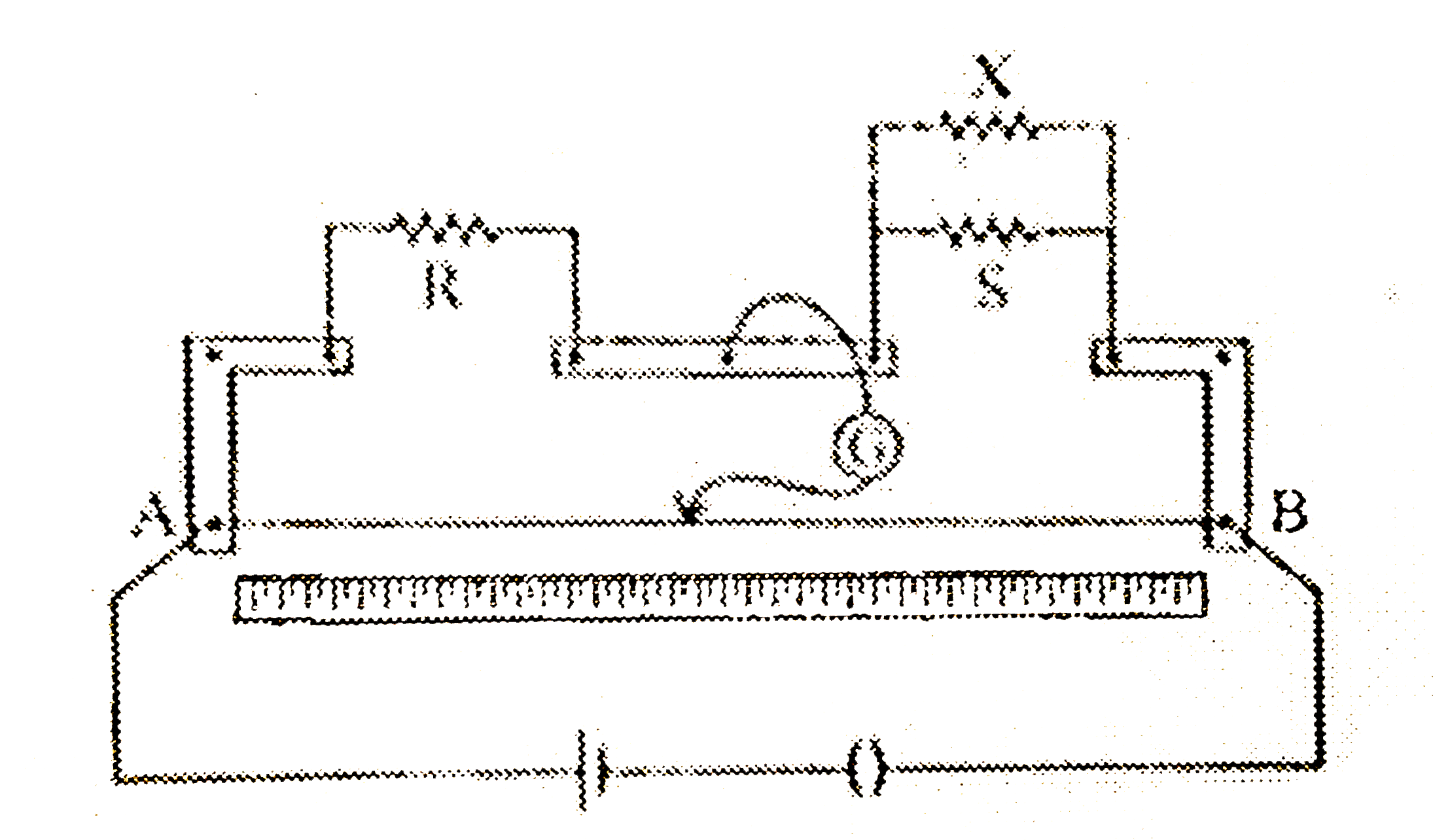
View SolutionResistance in the two gaps of a meter bridge are 10ohm and 30ohm respectively. If the resistances are interchanged he balance point shifts by
View SolutionIn a meter bridge, the null point is found at a distance of 40cm from A. If a resistance of 12Ω in connected in parallel with S the null point occurs at 50.0cm from A Determine the value of R and S

View Solution
