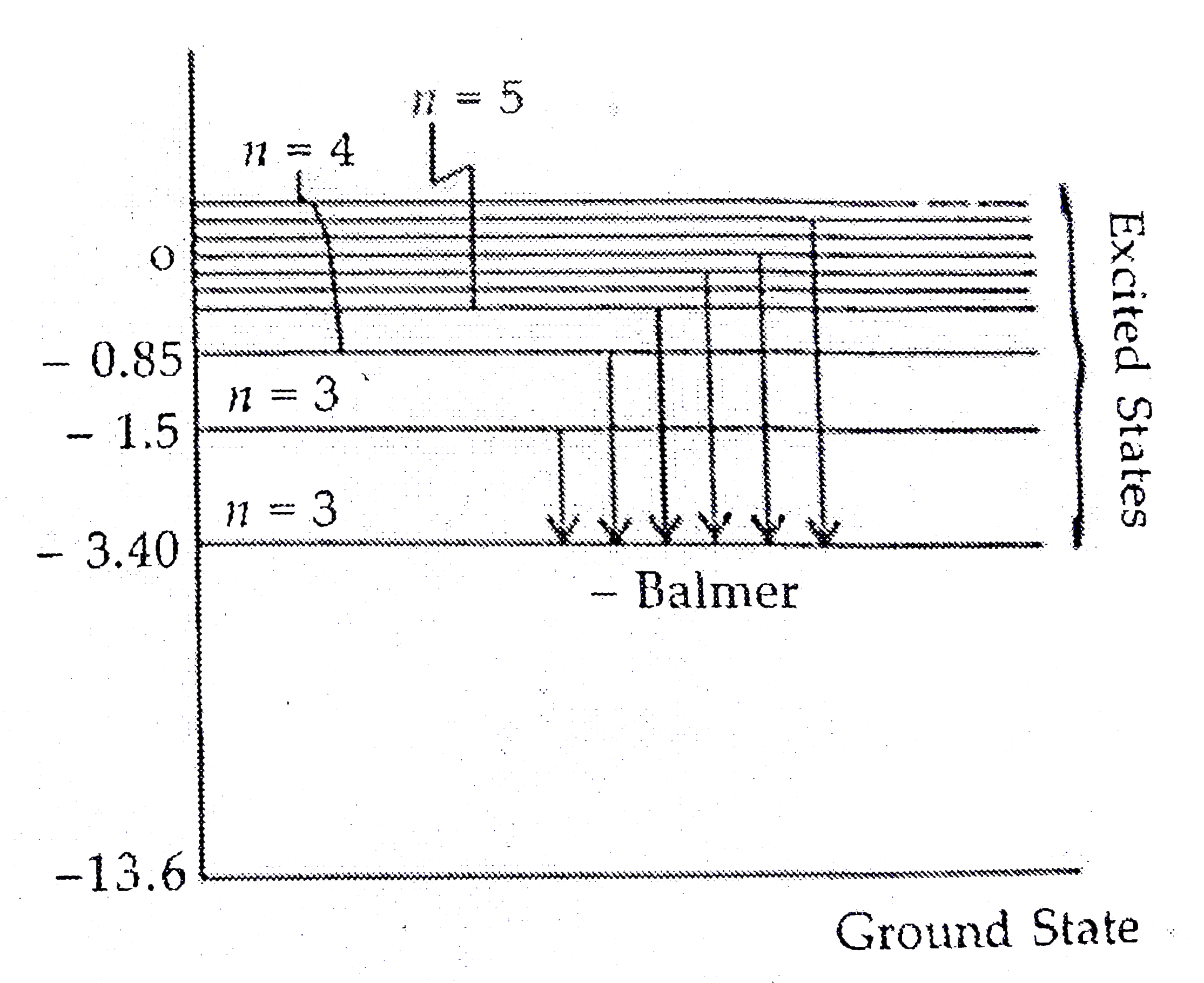
Using Bohor's postulates, obtain the expression for the total energy of the electron in the stationary states of the hydrogen atom. Hence draw the energy level diagram showing how the line spectra corresponding to Balmer series occur due to transition between energy levels.

Text Solution

Verified by Experts

According to Bohr's postulates, in a hydrogen atom, a single electron revolves arround a nucleus of charge +e. For an electron moving with a uniform speed in a circular orbit as a given radius, the centripetal force is provided by Coulomb force of attraction between the electron and the nucleus. The gravitational attraction may be neglected as the mass of electron and proton is very small.
$S o, \frac{M v^{2}}{r} = \frac{k e^{2}}{r^{2}} where \begin{matrix} m \to mass of electron r \to radius of electronic orbit v \to velocity of electron \end{matrix}$
$or m v^{2} = \frac{k e^{2}}{r}$ ...(i)
$Again, m v r = \frac{n h}{2 π}$
$v = \frac{n λ}{2 π m r}$
From equation (i), we get
$m (\frac{n λ}{2 π m r}) = \frac{k e^{2}}{r} = r = \frac{n^{2} h^{2}}{4 π^{2} k m e^{2}}$ ...(ii)
(i) Kinetic energy of electron,
$E_{k} = \frac{1}{2} m v^{2} = \frac{k e^{2}}{2 r}$
Using eq' (ii), we get
$E_{k} = \frac{k e^{2}}{2} \frac{4 π^{2} k m e^{2}}{n^{2} h^{2}} = \frac{2 π^{2} k^{2} m e^{4}}{n^{2} h^{2}}$
(ii) Potential energy
$E_{p} = \frac{- k (e) \times (e)}{r} = \frac{- k e^{2}}{r}$
Using equation (ii), we get,
$E_{p} = - k e^{2} \times \frac{4 π^{2} k m e^{2}}{n^{2} h^{2}} = \frac{- 4 π^{2} k^{2} m e^{4}}{n^{2} h^{2}}$
Hence, total energy of the electron in the nth orbit
$E = E_{p} + E_{k}$
$= \frac{- 4 π^{2} k^{2} m e^{4}}{n^{2} h^{2}} + \frac{2 π^{2} k^{2} m e^{4}}{n^{2} h^{2}} = \frac{- 2 π^{2} k^{2} m e^{4}}{n^{2} h^{2}} = - \frac{13.6}{n^{2}} e V .$
When the electron in a hydrogen atom jumps from higher energy level to the lower energy level, the difference of energies of the two energy levels is emitted as a radiation of particular wavelength. It is called a spectral line.
In H-atom, when an electron jumps from the orbit $n_{i}$ to orbit $n_{f'}$ the wavelength. It is called a spectral line.
in H-atom, when an electron jumps from the orbit $n_{i}$ to orbit $n_{f}$ the wavelength of the emitted radiation is given by,
$\frac{1}{λ} = R ⎛ ⎝ \frac{1}{n_{f}^{2}} - \frac{1}{n_{i}^{2}} ⎞ ⎠$
where $R \to Rydberg's constant = 1.09678 \times 10^{7} m^{- 1} .$
For Balmer series, $n_{f} = 2 and n_{i} = 3, 4, 5, ...$
$\frac{1}{λ} = R (\frac{1}{2^{2}} - \frac{1}{n_{i}^{2}})$
where, $n_{i} = 3, 4, 5, ...$
These spectral lines lie in the visible region.

Updated on:21/07/2023

Total energy of an electron in nth stationary orcit of hydrogen atom can be obtained by
A $E_{n} = \frac{13.6}{n^{2}} e V$
B $E_{n} = \frac{1.36}{n^{2}} e V$
C $E_{n} = - 13.6 \times n^{2} e V$
D $E_{n} = \frac{13.6}{n^{2}} e V$
Total energy of an electron in nth stationary orcit of hydrogen atom can be obtained by
A $E_{n} = \frac{13.6}{n^{2}} e V$
B $E_{n} = - \frac{13.6}{n^{2}} e V$
C $E_{n} = - \frac{13.6}{n^{2}} e V$
D $E_{n} = 13.6 x n^{2} e V$