When a plane wave front of light, of wavelength λ, is incident on a narrow slit, an intensity distribution pattern, of the form shown is observed on a screen, suitably kept behind the slit. Name the phenomenon observed.
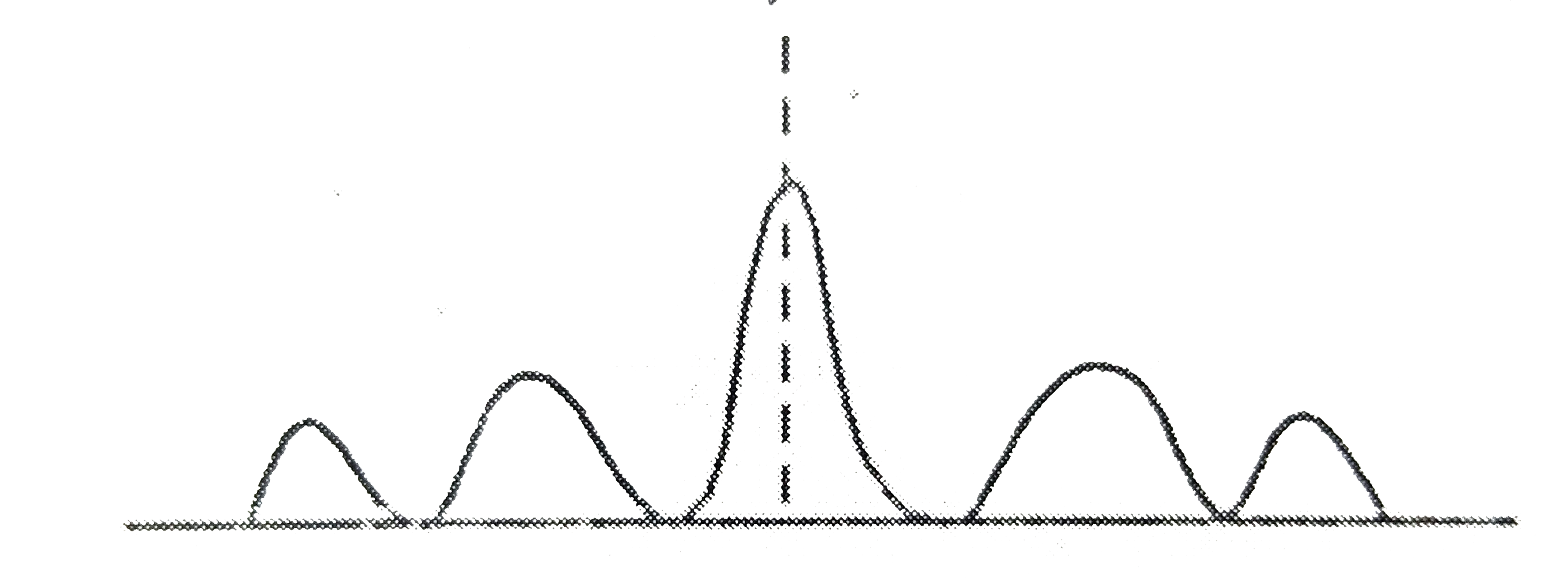
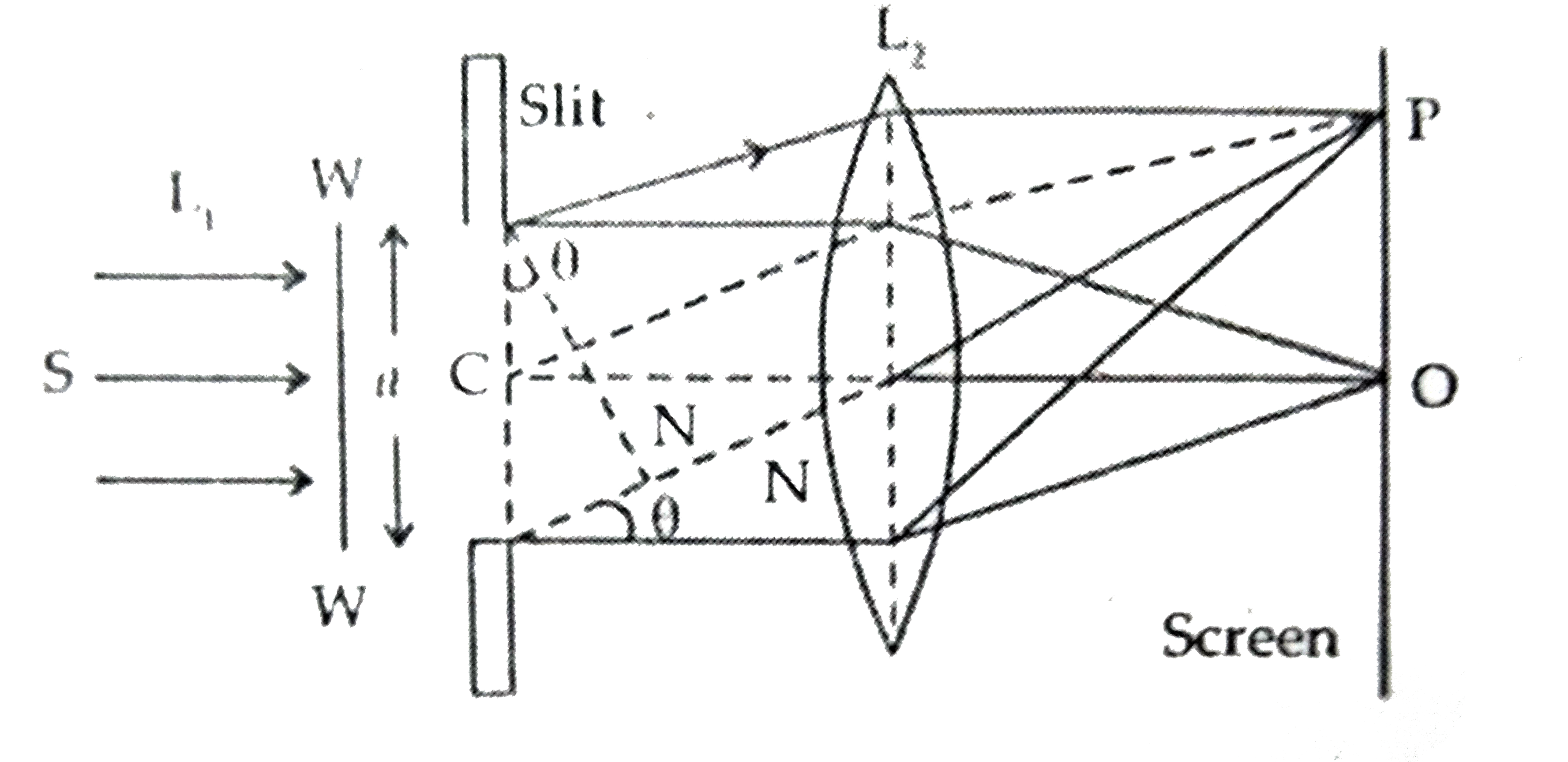
(a) Obtain the conditions for the formation of central maximum and secondary maxima and the minima.
(b) Why is there significant fall in intensity of the secondary maxima compared to the central maximum, whereas in double slit experiment all the bright fringes are of the same intensity ?
(c) When the width of the slit is made double the original width, how is the size of the central band affected ?
Phenomenon is Diffraction. All the secondary wavelets going straight across the slit are focussed at the central point of the screen. The wavelets from any two corresponding points of the two halves of the slit reach the point O in the same phase, they add constructively to produce a central bright fringe. The condition for first dark fringe is
asinθ1=λ
Similarly, the condition for second dark fringe will be
asinθ2=2λ
Hence, the condition for n th dark fringe can be written as
asinθn=nλ,n=1,2,3,...
The directions of various minima are given by
θn≈sinθn=nλa [As λ<<a, so sinθn=θn]
Suppose the point P is so located that p=3λ2
When θ=θ1, then a sinθ1=32λ
We can divide the slit into three equal parts. The path difference between two corresponding points of the first two parts will be λ2. The wavelets from these points will interfere destructively. However, the wavelets from the third part of the slit will contribute to some intensity forming a secondary maximum. The intensity of this maximum is much less than that of the central maximum.
Thus, the condition for the first secondary maximum is
ssinθ1'=32λ.
Similarly, the condition for the second secondary maximum is
asinθ2'52λ.
Hence the condition for nth secondary maximum can be written as
asinθn'=(2n+1)λ2,n=1,2,3.......
The directions of secondary maxima are given by
θn'≈θn'(2n+1)λ2a.
Similar Questions
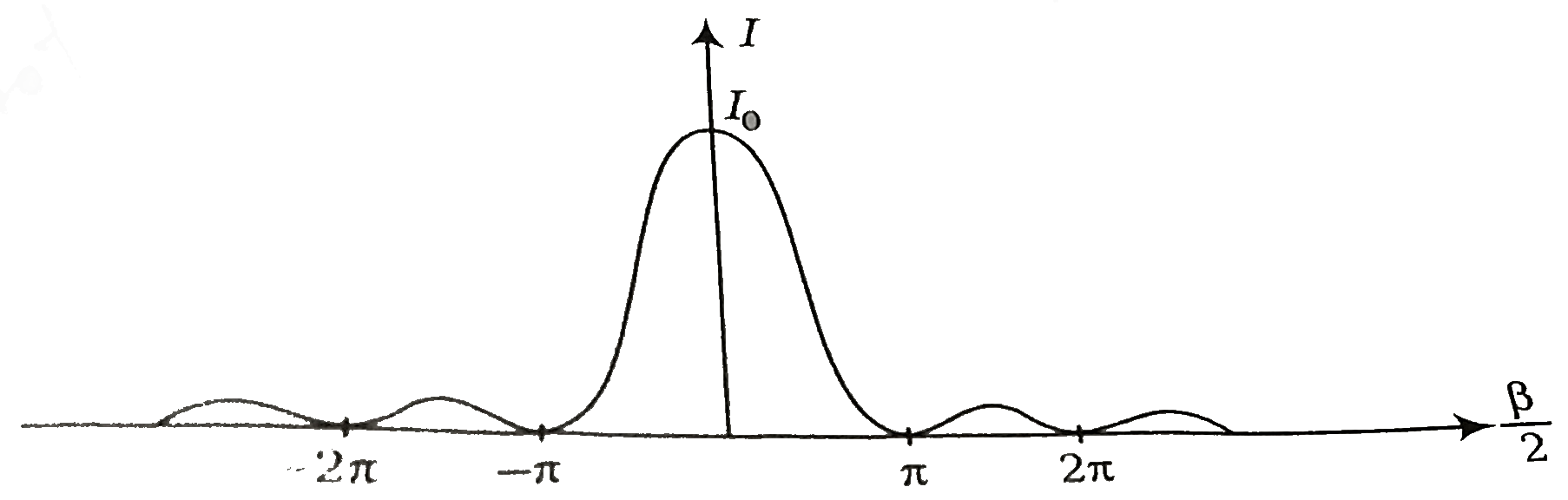
Find the ratio of the intensities of the secondary maxima to the intensity of the central maximum for the single slit Fraunhofer diffraction pattern.
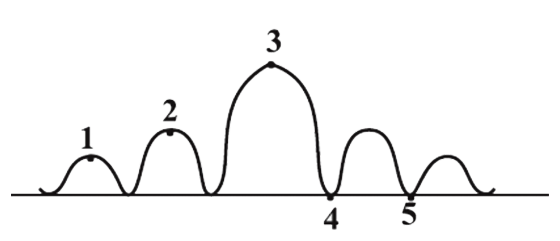
View SolutionThe diffraction pattern of a single slit is shown in the figure. The point at which the path difference of the extreme rays is two times the wavelength is
View Solution
Knowledge Check
In a Young’s double slit experiment, the ratio of the slit’s width is 4 : 1. The ratio of the intensity of maxima to minima, close to the central fringe on the screen, will be:
A(√3+1)4:16B25:9C4:1D9:1In a single slit diffraction of light of wavelength λ by a slit of width e, the size of the central maximum on a screen at a distance b is
A(a) 2bλ+eB(b) 2bλeC(c) 2bλe=eD(d) 2bλe−e
A beam of light of wavelength 400 nm falls on a narrow slit and the resulting diffraction pattern is observed on a screen 2 m away from the slit. It is observed that 2nd order minima occurs at a distance of 2 mm from the position of central maxima. Find the width of hte slit.
View SolutionA beam of light of wavelength 400 nm falls on a narrow slit and the resulting diffraction pattern is observed on a screen 2 m away from the slit. It is observed that 2nd order minima occurs at a distance of 2 mm from the position of central maxima. Find the width of the slit.
View SolutionIn single slit diffraction pattern, why is intensity of secondary maximum less than the intensity of central maximum ?
View SolutionIn a Young’s double slit experiment, the ratio of the slit’s width is 4 : 1. The ratio of the intensity of maxima to minima, close to the central fringe on the screen, will be:
View SolutionA monochromatic light of wavelength λ is incident normally on a narrow slit of width a to produce a diffraction pattern on the screen placed at a distacne D from the slit. With the help of a relevant diagram, deduce the condition for maxima and minima on the screen. use these condition to show that angular width of central maximum is twice the angular width of secondary maximum.
View SolutionIn a single slit diffraction of light of wavelength λ by a slit of width e, the size of the central maximum on a screen at a distance b is
View Solution
