A charge is distributed uniformly over a ring of radius 'a' Obtain an expression for the electric intensity E at a point on the axis of the ring . Hence show that for point a lerge distance from the ring it behaves like a point charge .
Suppose that the ring is placed with its plane perpendicular to the x-axis as shown in Fig. Consider a small element dl of the ring .
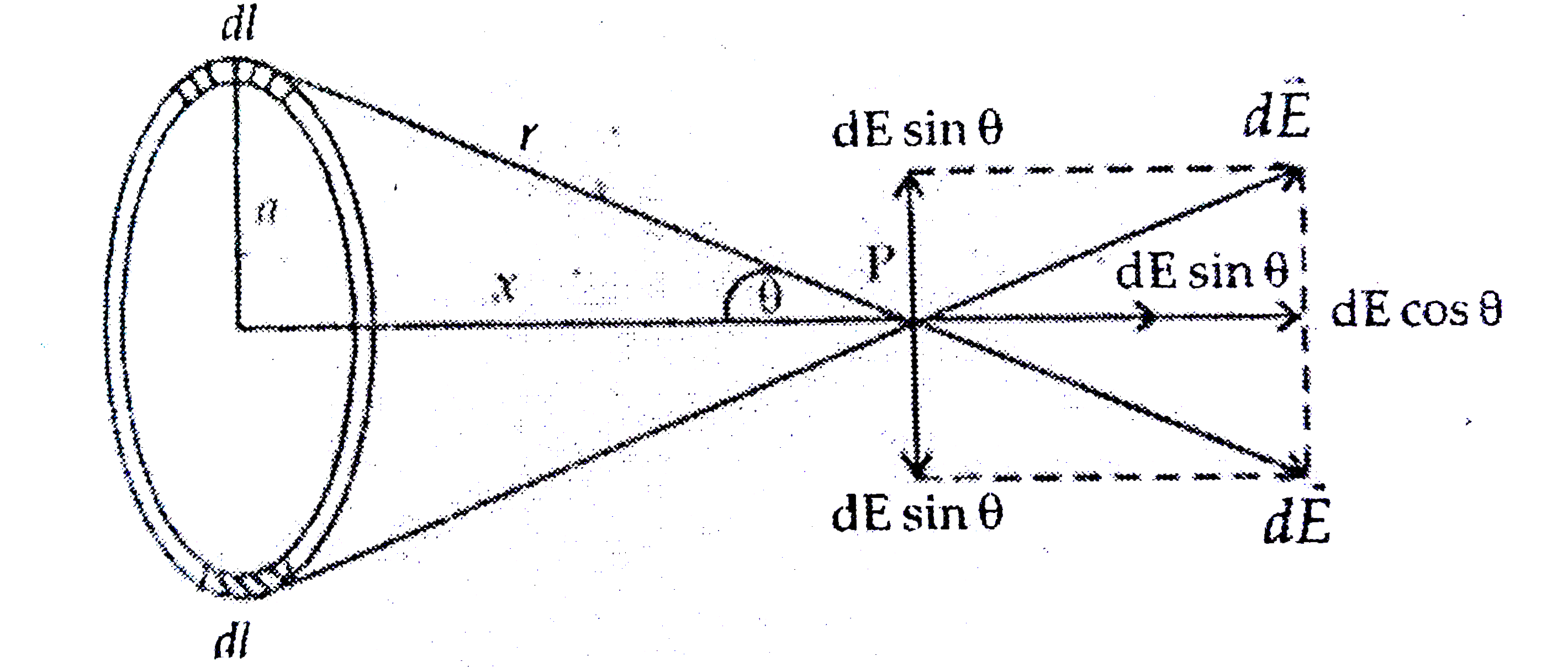
As the total charge q is uniformly distributed the charge dq on the element dl is dq =q2πa.dl
∴ The magnitude of the field d ¯¯¯E produced by the element dl at the field point P is
¯¯¯¯¯¯DE=k.dqr2=kq2πa.dlr2
The field d¯¯¯E has two components
(a) the axial component dE cos θ , and
(b) the perpendicular component dE sin θ
Since the perpendicular components of any two diametrically opposite elements are equal and opposite they all cancel out in pairs .Only the axial components will add up to produce the resultant field ¯¯¯E at point P which is given by
E=2πa∫0dE cos θ [∵only the axial components contribute towardsE]
=2πa∫0kq2πa,dlr2.xr=kpx2πa.1r32πa∫0dl
=kpx2πa.1r3[l]2πa0=kpx2πa.1(x2+a2)3/2.2πa [∵r2=x2+a2]
or E=kpx(x2+a2)3/2=14πε0.qx(x2+a2)3/2 ltbgt if x>>a
x2+a2≈x2
E=14πε0.qxx3⇒E=14πε0.qx2
i.e., When the point is far away from the centre charged ring acts like a point charge .
Topper's Solved these Questions
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:Section-D
Explore 18 Videos
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:Section-E
Explore 18 Videos
- XII BOARDSBook:XII BOARDS PREVIOUS YEARChapter:XII BOARDSExercise:Section -B
Explore 12 Videos
- SAMPLE PAPER 2019Book:XII BOARDS PREVIOUS YEARChapter:SAMPLE PAPER 2019Exercise:SECTION D
Explore 6 Videos
Similar Questions
A charge is distributed uniformly over a ring of radius 'a'. Obtain an expression for the electric intensity E at a point on the axis of the ring . Hence show that for points at large distances from the ring it behaves like a point charge .
View SolutionA charge is distributed uniformaly over is ring of radius 'a' .Obtain an expression for the electric intensity E at a point on the axis of the ring. Hence show that for points at large distances from the ring, it behaves like a point charge.
View SolutionA charge is distributed uniformly over a ring of radius ‘a’. Obtain an expression for the electric intensity E at a point on the axis of the ring. Hence show that for points at large distances from the ring, it behaves like a point charge.
View SolutionA charge is distributed uniformly over a ring of radius ‘a’. Obtain an expression for the electric intensity E at a point on the axis of the ring. Hence show that for points at large distances from the ring, it behaves like a point charge.
View SolutionA charge Q is uniformly distributed over a ring of radius a.Obtain an expression for electric field intensity at a point on the axis of ring. show that at far point ring behaves as a point charge.
View SolutionA charge Q is distributed uniformly on a ring of radius R as shown in the following diagrams. Find the electric potential at the centre O of the ring

View SolutionA thin circular ring of radius r is charged uniformly so that its linear charge density becomes λ . Derive an expression for the electric field at a point P at a distance x from it along the axis of the ring. Hence, prove that at large distances (r >> r), the ring behaves as a point charge.
View SolutionA charge Q is distributed uniformly on a ring of radius R as shown in the following diagrams. Find the electric potential at the center O of the ring.
(a)
View SolutionMass of 10 kg is distributed uniformly over a ring of radius 1m. Find the gravitational potential at a point lies on the axis of the ring at a distance of 1m from the centre.
View SolutionMass of 1 kg is distributed uniformly over a ring of radius 1m. Find the gravitational potential at a point lies on the axis of the ring at a distance of 1m from the centre.
View Solution
