(a) Derive the expression for the electric potential at any point P, at distance r from the centre of an electric dipole, making angle $α$ , with its axis.
(b) Two point charges $4 μ C and + 1 μ C$ are separated by a distance of 2m in air. Find the point on the line joining charges at which the net electric field of the system is zero.

Video Solution

Text Solution

Verified by Experts

(a) $V_{1}$ = Potentil at P due to $(- q) = \frac{1}{4 π ε_{0}} \times \frac{- q}{r_{1}}$
$V_{2} =$ Potential at P due to $q = \frac{1}{4 π ε_{0}} \times \frac{q}{r_{2}}$
Potential at $P = V = V_{1} + V_{2}$
$V = \frac{- 1}{4 π ε_{0}} \times \frac{q}{r_{1}} + \frac{1}{4 π ε_{0}} \times \frac{q}{r_{2}}$
$\Rightarrow V = \frac{q}{4 π ε_{0}} [\frac{1}{r_{2}} - \frac{1}{r_{1}}]$
$r_{1} = A P = C P = r + 1 cos θ and r_{2} B P = D P = r - l cos θ$
$V = \frac{q}{4 π ε_{0}} [\frac{1}{(r - l cos θ)} - \frac{1}{(r + l cos θ)}]$

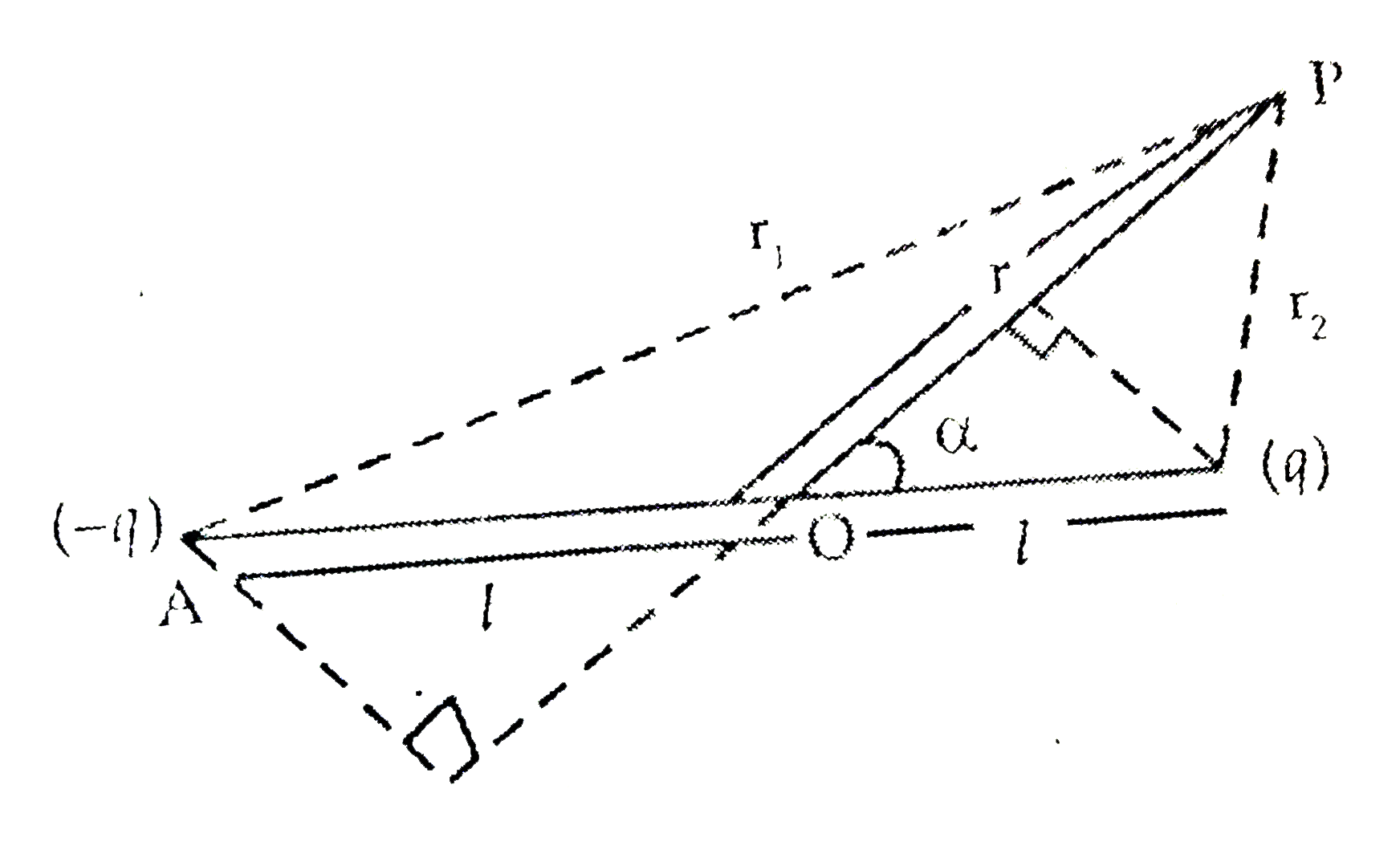
$\Rightarrow V = \frac{q}{4 π ε_{0}} [\frac{r + l cos θ r + l cos θ}{r^{2} - l^{2} {cos}^{2} θ}]$
$V = \frac{q}{4 π ε_{0}} [\frac{2 l cos θ}{r^{2} - l^{2} {cos}^{2} θ}]$
$V = \frac{2 l q cos θ}{4 π ε_{0} (r^{2} - l^{2} {cos}^{2} θ)} = \frac{P cos θ}{4 π ε_{0} (r^{2} - l^{2} {cos}^{2} θ)}$
(b) $E = \frac{1}{4 π ε_{0}} \frac{q}{r^{2}} \Rightarrow E_{1} = \frac{1}{4 π ε_{0}} \times \frac{4 \times 10^{- 6}}{x^{2}} and E_{2} = \frac{1}{4 π ε_{0}} \times \frac{1 \times 10^{- 6}}{{(2 - x)}^{2}}$
ATQ $E_{1} = E_{2} or \frac{1}{4 π ε_{0}} \times \frac{4 \times 10^{- 6}}{x^{2}} = \frac{1}{4 π ε_{0}} \times \frac{4 \times 10^{- 6}}{{(2 - x)}^{2}}$
$\frac{2}{x} = \frac{1}{2 - x} \Rightarrow 4 - 2 x = x x = .^{4} /_{3} m and 2 - x = .^{2} /_{3} m$

Updated on:21/07/2023

The two point charges $4 μ C$ and $1 μ C$ are separated by a distance of 2 m in air. Find the point on the line joining the charges at which net electric field of the system is zero.
A $\frac{4}{3} m$ from $1 μ C$
B $\frac{2}{3} m$ from $1 μ C$
C $\frac{4}{3} m$ from $4 μ C$
D $\frac{2}{3} m$ from $4 μ C$
Two positive point charges $q_{1} = 16 μ C$ and $q_{2} = 4 μ C$ are separated in vacuum by a distance of $3.0 m$ . Find the point on the line between the charges where the net electric field is zero.-
A $2 m$ from $q_{1}$
B $1 m$ from $q_{1}$
C $2 m$ from $q_{2}$
D $0.5 m$ from $q_{1}$