A 12.5 eV electron beam is used to bombard gaseous hydrogen at room temperature. What series of wavelengths will be emitted ?

Text Solution
Verified by Experts

We know that energy of an electron in nth orbit of hydrogen is En=−13.6n2 and energy in ground state of hydrogen is -13.6 eV.
When an electron beam of 12.5 eV is used , hydrogen atom cannot be ionised but at the most excited to n = 3 state where energy of the electron will be −13.6(3)2=−1.51eV (as maximum energy of electron can be −13.6+12.5=−1.1eV and electron cannot be excited to n = 4 state having energy - 0.85 eV)
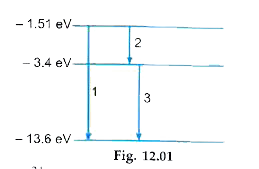
So only three transitions are possible
So only three transitions are possible as shwon in Fig. 12.01.
Here transtions No.1 and 3 represent lins of Lyman series .
and transition No.2 represents line of Balmer series .
Wavelength of light emiited in a transition will be .
λ=cv=ch(Ei−Ef)
∴ λ1=3×108×6.63×10−34[(−1.51−(−13.6)]×1.6×10−19)=3×108×6.63×10−3412.09×1.60×10−19=1.03×10−7m=103nm
λ2=3×10×6.63×10−34[(−1.51−(−3.4)]×1.6×10)−19=3×108×6.63×10−341.89×1.6×10−19=6.56×10−7m=656nm
and λ3=3×108×6.63×10−34[(−3.4−(−13.6)]×1.6×10−19)=3×108×6.63×10−3410.2×1.6×10−19=1.22×10−7m=122nm
ocr_image

Updated on:21/07/2023
Doubtnut Promotions Banner
|
Class 12PHYSICSATOMS

Topper's Solved these Questions

Similar Questions