Question

In a circle of diameter 40 cm. The length of a chord is 20 cm. Find the length of minor arc of the chord.

Explanation

Diameter of the circle =40 cm
∴ Radius (r) of the circle = `40/2 cm`
​

`=20 cm`


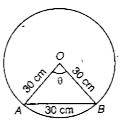
Let AB be a chord (length = `20 cm`) of the circle.
In `△OAB, OA = OB` = Radius of circle = `20 cm`



Also, `AB = 20 cm`



Thus, `△OAB` is an equilateral triangle.


`∴θ=60^2 = pi/3 radian`
​


We know that in a circle of radius r unit, if an arc of length I unit subtends an angle θ radian at the centre, then ` θ= 1/r`


then, `pi/3 = arc (AB)/ 20 = arc AB = 20 pi/3` cm