The base of an equilateral triangle with side 2a lies along the y–axis such that the mid–point of the base is at the origin. Find vertices of the triangle.
To find the vertices of an equilateral triangle with a base of length 2a lying along the y-axis and with the midpoint of the base at the origin, we can follow these steps:
Step 1: Identify the Midpoint and Length of the Base
Given that the midpoint of the base is at the origin (0, 0), and the length of the base is 2a, we can determine the coordinates of the endpoints of the base. The base lies along the y-axis.
Step 2: Determine the Coordinates of the Base Vertices
Since the base is centered at the origin and has a length of 2a, the coordinates of the endpoints of the base can be calculated as follows:
- The point at the top of the base (point A) will be at (0,a).
- The point at the bottom of the base (point B) will be at (0,−a).
Step 3: Find the Third Vertex of the Triangle
To find the third vertex (point C) of the equilateral triangle, we need to use the property of equilateral triangles where all sides are equal. The distance from point A to point C and from point B to point C should both equal 2a.
Step 4: Use the Pythagorean Theorem
We can consider the triangle formed by points A, B, and C. The distance from the origin to point C will be the x-coordinate (let's call it x) and the y-coordinate will be 0 since point C lies on the x-axis.
Using the Pythagorean theorem:
(2a)2=a2+x2
This simplifies to:
4a2=a2+x2
Step 5: Solve for x
Rearranging the equation gives:
x2=4a2−a2=3a2
Taking the square root of both sides:
x=√3a
Step 6: Write the Coordinates of Point C
Thus, the coordinates of point C are:
C=(√3a,0)
Final Coordinates of the Triangle Vertices
The vertices of the equilateral triangle are:
- Point A: (0,a)
- Point B: (0,−a)
- Point C: (√3a,0)
Summary
The vertices of the equilateral triangle are:
- A(0,a)
- B(0,−a)
- C(√3a,0)
---
Given that the midpoint of the base is at the origin (0, 0), and the length of the base is 2a, we can determine the coordinates of the endpoints of the base. The base lies along the y-axis.
Since the base is centered at the origin and has a length of 2a, the coordinates of the endpoints of the base can be calculated as follows:
- The point at the top of the base (point A) will be at (0,a).
- The point at the bottom of the base (point B) will be at (0,−a).
To find the third vertex (point C) of the equilateral triangle, we need to use the property of equilateral triangles where all sides are equal. The distance from point A to point C and from point B to point C should both equal 2a.
We can consider the triangle formed by points A, B, and C. The distance from the origin to point C will be the x-coordinate (let's call it x) and the y-coordinate will be 0 since point C lies on the x-axis.
(2a)2=a2+x2
This simplifies to:
4a2=a2+x2
Rearranging the equation gives:
x2=4a2−a2=3a2
Taking the square root of both sides:
x=√3a
Thus, the coordinates of point C are:
C=(√3a,0)
The vertices of the equilateral triangle are:
- Point A: (0,a)
- Point B: (0,−a)
- Point C: (√3a,0)
The vertices of the equilateral triangle are:
- A(0,a)
- B(0,−a)
- C(√3a,0)
Topper's Solved these Questions
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 10.2
20 Videos
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE 10.5
2 Videos
- STRAIGHT LINESBOOK - NCERT ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - MISCELLANEOUS EXERCISE
24 Videos
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - EXERCISE 15.2
10 Videos
- TRIGONOMETRIC FUNCTIONSBOOK - NCERT ENGLISHCHAPTER - TRIGONOMETRIC FUNCTIONSEXERCISE - All Questions
110 Videos
Similar Questions
The base of an equilateral triangle with side 2a lies along the y-axis such that the mid point of the base is at the origin. Find the vertices of the triangle.
View SolutionThe base of an equilateral triangle with side 20cm lies along x-axis such that the mid-point of the base is at the origin. Find the vertices of the triangle
View Solution
Knowledge Check
What is the area of equilateral triangle if the base BC = 6 ?
 A9√3B18√3C26√3D30√3
A9√3B18√3C26√3D30√3
Two uniform spheres A (Hollow) and B (solid) of same radius R (√125GmPr, where P is _____
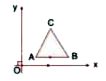
View SolutionThe base AB of two equilateral triangles ABC and ABC' with side 2a lies along the X -axis such that the mid-point of AB is at the origin as shown in . Find the coordinates of the vertices C and C' of the triangles.
View SolutionLet the point P lies in the interior of an equilateral triangle with side lengths 2 units, each. Find the sum of the shortest distances from P to the sides of the triangle.
View SolutionIf (-4,3) and (4,3) are two vertices of an equilateral triangle, then find the coordinates of the third vertex, given that the origin lies in the interior of the triangle.
View SolutionThree identical positive charges Q are arranged at the vertices of an equilateral triangle. The side of the triangle is a. Find the intensity of the field at the vertex of a regular tetrahedron of which the triangle is the base.
View SolutionThe side AB of an equilateral triangle ABC is parallel to the x-axis. Find the slopes of all its sides.
View SolutionA side of an equilarteral triangle is 20 cm long .A second equilateral triangle is inscribed in it by joning the mid -point of the sides of the first triangle . The process is caontinued as shown in the accompanying diagram . find the perimeter of the sixth inscribed equilateral triangle .
View Solution
