The base of an equilateral triangle with side 2a lies along the y-axis such that the mid point of the base is at the origin. Find the vertices of the triangle.
To find the vertices of the equilateral triangle with a base of length 2a lying along the y-axis and the midpoint of the base at the origin, we can follow these steps:
Step 1: Understand the Configuration
The base of the triangle is along the y-axis, and the midpoint of the base is at the origin (0, 0). Since the length of the base is 2a, each half of the base will be a.
Step 2: Determine the Coordinates of the Base Vertices
Since the base lies along the y-axis and is centered at the origin, the coordinates of the vertices of the base can be determined as follows:
- The lower vertex will be at (0,−a)
- The upper vertex will be at (0,a)
Thus, the coordinates of the base vertices are:
- B(0,a)
- C(0,−a)
Step 3: Find the Vertex of the Triangle
The third vertex of the triangle (let's call it A) will be located above the base. Since the triangle is equilateral, we need to find the x-coordinate of vertex A such that the distance from A to both B and C is equal to 2a.
Let the coordinates of vertex A be (x,0).
Step 4: Use the Distance Formula
Using the distance formula, we can set up the following equations:
1. Distance AB:
AB=√(0−x)2+(a−0)2=√x2+a2
Setting this equal to 2a:
√x2+a2=2a
2. Squaring both sides:
x2+a2=(2a)2
x2+a2=4a2
x2=4a2−a2=3a2
3. Taking the square root:
x=±√3a
Step 5: Write the Coordinates of the Vertices
Now we have the coordinates of all three vertices:
- A(√3a,0) or A(−√3a,0)
- B(0,a)
- C(0,−a)
Thus, the vertices of the triangle are:
- A(√3a,0)
- B(0,a)
- C(0,−a)
Final Answer
The vertices of the equilateral triangle are:
1. A(√3a,0)
2. B(0,a)
3. C(0,−a)
---
The base of the triangle is along the y-axis, and the midpoint of the base is at the origin (0, 0). Since the length of the base is 2a, each half of the base will be a.
Since the base lies along the y-axis and is centered at the origin, the coordinates of the vertices of the base can be determined as follows:
- The lower vertex will be at (0,−a)
- The upper vertex will be at (0,a)
- B(0,a)
- C(0,−a)
The third vertex of the triangle (let's call it A) will be located above the base. Since the triangle is equilateral, we need to find the x-coordinate of vertex A such that the distance from A to both B and C is equal to 2a.
Using the distance formula, we can set up the following equations:
AB=√(0−x)2+(a−0)2=√x2+a2
Setting this equal to 2a:
√x2+a2=2a
x2+a2=(2a)2
x2+a2=4a2
x2=4a2−a2=3a2
x=±√3a
Now we have the coordinates of all three vertices:
- A(√3a,0) or A(−√3a,0)
- B(0,a)
- C(0,−a)
- A(√3a,0)
- B(0,a)
- C(0,−a)
The vertices of the equilateral triangle are:
1. A(√3a,0)
2. B(0,a)
3. C(0,−a)
Topper's Solved these Questions
Similar Questions
The base of an equilateral triangle with side 2a lies along the y–axis such that the mid–point of the base is at the origin. Find vertices of the triangle.
View SolutionThe base of an equilateral triangle with side 20cm lies along x-axis such that the mid-point of the base is at the origin. Find the vertices of the triangle
View Solution
Knowledge Check
What is the area of equilateral triangle if the base BC = 6 ?
 A9√3B18√3C26√3D30√3
A9√3B18√3C26√3D30√3
Two uniform spheres A (Hollow) and B (solid) of same radius R (√125GmPr, where P is _____
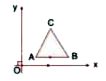
View SolutionThe base AB of two equilateral triangles ABC and ABC' with side 2a lies along the X -axis such that the mid-point of AB is at the origin as shown in . Find the coordinates of the vertices C and C' of the triangles.
View SolutionLet the point P lies in the interior of an equilateral triangle with side lengths 2 units, each. Find the sum of the shortest distances from P to the sides of the triangle.
View SolutionIf (-4,3) and (4,3) are two vertices of an equilateral triangle, then find the coordinates of the third vertex, given that the origin lies in the interior of the triangle.
View SolutionEquation of the base of an equilateral triangle is 3x+4y=9 and its vertex is at point (1,2) .Find the equations of the other sides and the length of each side of the triangle .
View SolutionThe side AB of an equilateral triangle ABC is parallel to the x-axis. Find the slopes of all its sides.
View SolutionThe base QR of an equilateral triangle PQR lies on X-axis. The co-ordinates of the point Q are (-4,0) and origin is the mid-point of the base. Find the co-ordinates of the points P and R.
View Solution
