Using integration, find the area of the region enclosed between the two circles $x^{2} + y^{2} = 4$ and ${(x - 2)}^{2} + y^{2} = 4 .$

Video Solution

Text Solution

Verified by Experts

Equations of the given circles are $x^{2} + y^{2} = 4 (eq 1) and {(x - 2)}^{2} + y^{2} - 4 (eq 1)$
Equation (1) is a circle with centre O at the origin and radius 2. Equation (2) is a circle with centre C (2, 0) and radius 2.
Solving equations (1) and (2), we have
$x = 1 and y = \pm \sqrt{3}$ Thus, the points of intersection of the given circles are A (1,3) and A′ (1,–3)

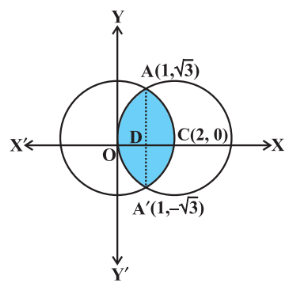
Required area of the enclosed region OACA′O between circles= 2 [area of the region ODCAO]
= 2 [area of the region ODAO + area of the region DCAD]
$2 [\int_{0}^{2} y d x + \int_{1}^{2} y d x]$
$2 [\int_{0}^{1} (\sqrt{4 - {(x - 2)}^{2}} d x + \int_{1}^{2} \sqrt{4 - x^{2}} d x]$
now by using formula $\int \sqrt{x^{2} - x^{2}} = \frac{x}{2} \sqrt{x^{2} - a^{2}} + \frac{a^{2}}{2} {sin}^{- 1} (\frac{x}{a}) + C$
by solving above integration and putting the value of the limits we will get
$(- \sqrt{3} - \frac{2 π}{3} + 2 π) + (2 π - \sqrt{3} - \frac{2 π}{3})$
so by solving and simplifying this we will get $\frac{8 π}{3} - 2 \sqrt{3}$ as a final answer

|

Updated on:21/07/2023

Class 12 MATHS APPLICATION OF INTEGRALS

Topper's Solved these Questions

Similar Questions

NCERT ENGLISH-APPLICATION OF INTEGRALS-SOLVED EXAMPLES

Home
Ask Doubt
Profile