Find the area of the segment AYB of circle, if radius of the circle centered at O is 21 cm and ∠AOB = 120o
To find the area of the segment AYB of a circle with a radius of 21 cm and an angle AOB of 120 degrees, we can follow these steps:
Step 1: Calculate the Area of the Sector AOB
The area of a sector of a circle can be calculated using the formula:
Area of Sector=θ360×πr2
Where:
- θ is the angle in degrees,
- r is the radius of the circle,
- π is approximately 22/7.
Given:
- θ=120∘,
- r=21 cm.
Plugging in the values:
Area of Sector AOB=120360×227×(21)2
Calculating:
=13×227×441
=22×44121=462 cm2
Step 2: Calculate the Area of Triangle AOB
To find the area of triangle AOB, we can use the formula:
Area of Triangle=12×base×height
We need to find the base (AB) and the height (OM).
Finding the Length of AB
To find AB, we can use the sine function in triangle AOB. Since AO and OB are both radii of the circle:
AO=OB=21 cm
Using the sine of half the angle (60 degrees):
sin(60∘)=AMAO
Where AM is half the length of AB. Thus:
sin(60∘)=√32=AM21
Solving for AM:
AM=21×√32=21√32
Thus, the length of AB is:
AB=2×AM=2×21√32=21√3 cm
Finding the Height OM
Using the Pythagorean theorem in triangle AOM:
AO2=AM2+OM2
Substituting the known values:
212=(21√32)2+OM2
Calculating:
441=441×34+OM2
441=13234+OM2
OM2=441−13234=1764−13234=4414
OM=212 cm
Area of Triangle AOB
Now we can find the area of triangle AOB:
Area of Triangle AOB=12×AB×OM
=12×21√3×212
=441√34 cm2
Step 3: Calculate the Area of Segment AYB
The area of the segment AYB is given by:
\text{Area of Segment AYB} = \text{Area of Sector AOB} - \text{Area of Triangle AOB
Substituting the values:
=462−441√34
Final Answer
Thus, the area of the segment AYB is:
Area of Segment AYB=462−441√34 cm2
The area of a sector of a circle can be calculated using the formula:
- θ is the angle in degrees,
- r is the radius of the circle,
- π is approximately 22/7.
- θ=120∘,
- r=21 cm.
To find the area of triangle AOB, we can use the formula:
To find AB, we can use the sine function in triangle AOB. Since AO and OB are both radii of the circle:
Using the Pythagorean theorem in triangle AOM:
Now we can find the area of triangle AOB:
The area of the segment AYB is given by:
\text{Area of Segment AYB} = \text{Area of Sector AOB} - \text{Area of Triangle AOB
Thus, the area of the segment AYB is:
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure, O is the center of the circle. What is average of the numbers a, b, c and d?
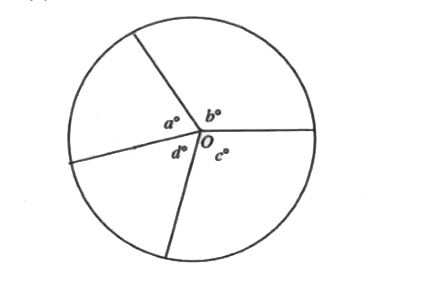 A45B60C90D180
A45B60C90D180
Find the area of the segment of a circle, given that the angle of the sector is 120o and the radius of the circle is 21 cm. (Take π=22/7)
View SolutionFind the area of the shaded region in Fig. 12.20, if radii of the two concentric circles with centre O are 7 cm and 14 cm respectively and ∠AOC=40∘
View SolutionIf the area of a sector of a circle is 720 of the area of the circle, then the sector angle is equal to (a) 110o (b) 130o (c) 100o (d) 126o
View SolutionIn Figure, two circles intersect at A and B . The centre of the smaller circle is O and it lies on the circumference of the larger circle. If ∠APB=700, find ∠ACB
View SolutionFind the area of a circle whose radius is (a)7 cm (b) 2.1 m (c)7 km
View SolutionIn Figure, O is the centre of circle of radius 28 cm. Find the area of minor segment ASB .
View SolutionIn Fig. 10.36, A,B and C are three points on a circle with centre O such that ∠BOC= 30o and ∠AOB= 60o. If D is a point on the circle other than the arc ABC, find ∠ADC.
View Solution
