Diagonal AC of a parallelogram ABCD bisects ∠A. Show that
(i) it bisects ∠C also,
(ii) ABCD is a rhombus.
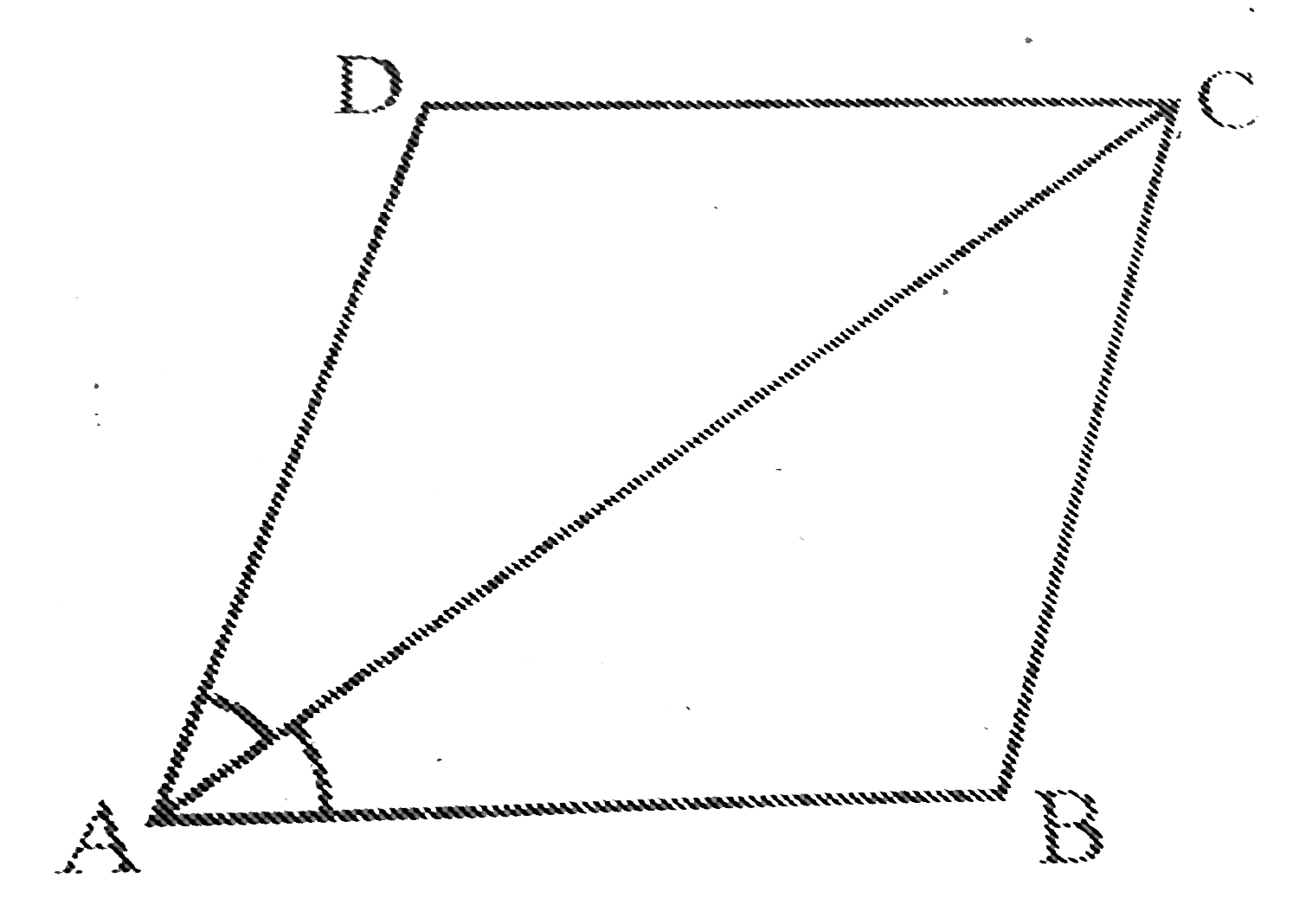
Given: The diagonal AC of a parallelogram ABCD bisects ∠A.
We can use alternate interior angles property to show that the diagonal AC bisects ∠C and by showing all sides are equal, it can be proved ABCD is a rhombus.
i) ABCD is a parallelogram.
∠DAC=∠BCA (Alternate interior angles) ....................(1)
∠BAC=∠DCA (Alternate interior angles) ....................(2)
However, it is given that AC bisects ∠A.
∠DAC=∠BAC ....................(3)
From equations (1), (2), and (3), we obtain
∠DCA=∠BAC=∠DAC=∠BCA ....................(4)
Thus, ∠DCA=∠BCA
Hence, AC bisects ∠C.
ii) From Equation (4), we obtain
∠DAC=∠DCA
DA=DC (Side opposite to equal angles are equal)
However, DA=BC and AB=CD (Opposite sides of a parallelogram are equal)
Thus, AB=BC=CD=DA
Hence, ABCD is a rhombus.
Topper's Solved these Questions
- QUADRILATERALSBOOK - NCERT ENGLISHCHAPTER - QUADRILATERALSEXERCISE - SOLVED EXAMPLES
8 Videos
- QUADRILATERALSBOOK - NCERT ENGLISHCHAPTER - QUADRILATERALSEXERCISE - Exercise 8.2
7 Videos
- PROBABILITYBOOK - NCERT ENGLISHCHAPTER - PROBABILITYEXERCISE - SOLVED EXAMPLES
10 Videos
- STATISTICSBOOK - NCERT ENGLISHCHAPTER - STATISTICSEXERCISE - Exercise 14.2
9 Videos
Similar Questions
Diagonal AC of a paraleligram ABCD bisects ∠A (sec figure). Show that:
(i) it bisects ∠C also (ii) ABCD is a rhombus.
View SolutionThe diagonals of a parallelogram bisect each other.
View Solutionif diagonals of a parallelogram bisect each other,prove that its a rhombus
View SolutionThe diagonal BD of a parallelogram ABCD bisects angles B and D. Prove that ABCD is a rhombus.
View SolutionProve that the diagonals of a parallelogram bisect each other.
View SolutionIn a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Find AB:AD
View SolutionPoints M and N are taken on the diagonal AC of a parallelogram ABCD such that AM= CN. Prove that BMDN is a parallelogram.
View SolutionIn a parallelogram ABCD, the bisector of ∠A also bisects BC at X. Prove that AD=2AB.
View SolutionABCD is a parallelogram E is mid-point of AB and DE bisects angle D. Prove that
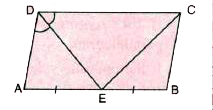
CE bisects angle C
View SolutionP and Q are points on opposite sides AD and BC of a parallelogram ABCD such that PQ passes through the point of intersection O of its diagonals AC and BD. Show that PQ is bisected at O.
View Solution
