Asha and Samuel have bookshelves of the same size partly filled with books. Asha’s shelf is 56 th full and Samuel’s shelf is 25th full. Whose bookshelf is more full? By what fraction?
To determine whose bookshelf is more full and by what fraction, we need to compare the fractions representing the fullness of Asha's and Samuel's bookshelves.
Step 1: Identify the fractions
Asha's bookshelf is 56 full, and Samuel's bookshelf is 25 full.
Step 2: Find a common denominator
To compare these two fractions, we need to find a common denominator. The denominators are 6 and 5. The least common multiple (LCM) of 6 and 5 is 30.
Step 3: Convert the fractions
Now, we convert both fractions to have a denominator of 30.
- For Asha's bookshelf:
56=5×56×5=2530
- For Samuel's bookshelf:
25=2×65×6=1230
Step 4: Compare the fractions
Now we can compare 2530 and 1230. Clearly, 2530 is greater than 1230.
Step 5: Find the difference
To find out by what fraction Asha's bookshelf is more full than Samuel's, we subtract Samuel's fraction from Asha's fraction:
2530−1230=25−1230=1330
Conclusion
Asha's bookshelf is more full than Samuel's bookshelf by 1330.
---
Asha's bookshelf is 56 full, and Samuel's bookshelf is 25 full.
To compare these two fractions, we need to find a common denominator. The denominators are 6 and 5. The least common multiple (LCM) of 6 and 5 is 30.
Now, we convert both fractions to have a denominator of 30.
56=5×56×5=2530
25=2×65×6=1230
Now we can compare 2530 and 1230. Clearly, 2530 is greater than 1230.
To find out by what fraction Asha's bookshelf is more full than Samuel's, we subtract Samuel's fraction from Asha's fraction:
2530−1230=25−1230=1330
Asha's bookshelf is more full than Samuel's bookshelf by 1330.
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - FRACTIONSEXERCISE - EXERCISE 7.23videos
FRACTIONS
- BOOK - NCERT ENGLISHCHAPTER - FRACTIONSEXERCISE - EXERCISE 7.39videos
FRACTIONS
- BOOK - NCERT ENGLISHCHAPTER - FRACTIONSEXERCISE - SOLVED EXAMPLES13videos
FRACTIONS
- BOOK - NCERT ENGLISHCHAPTER - DECIMALSEXERCISE - EXERCISE 8.32videos
DECIMALS
- BOOK - NCERT ENGLISHCHAPTER - INTEGERSEXERCISE - EXERCISE 6.25videos
INTEGERS
Similar Questions
Asha and Samuel have bookshelves of the same size partly find with books. Asha's shelf is 6 5 th full and Samuel's shelf is 5 2 th full. Whose bookshelf is more full? By what fraction?
View Solution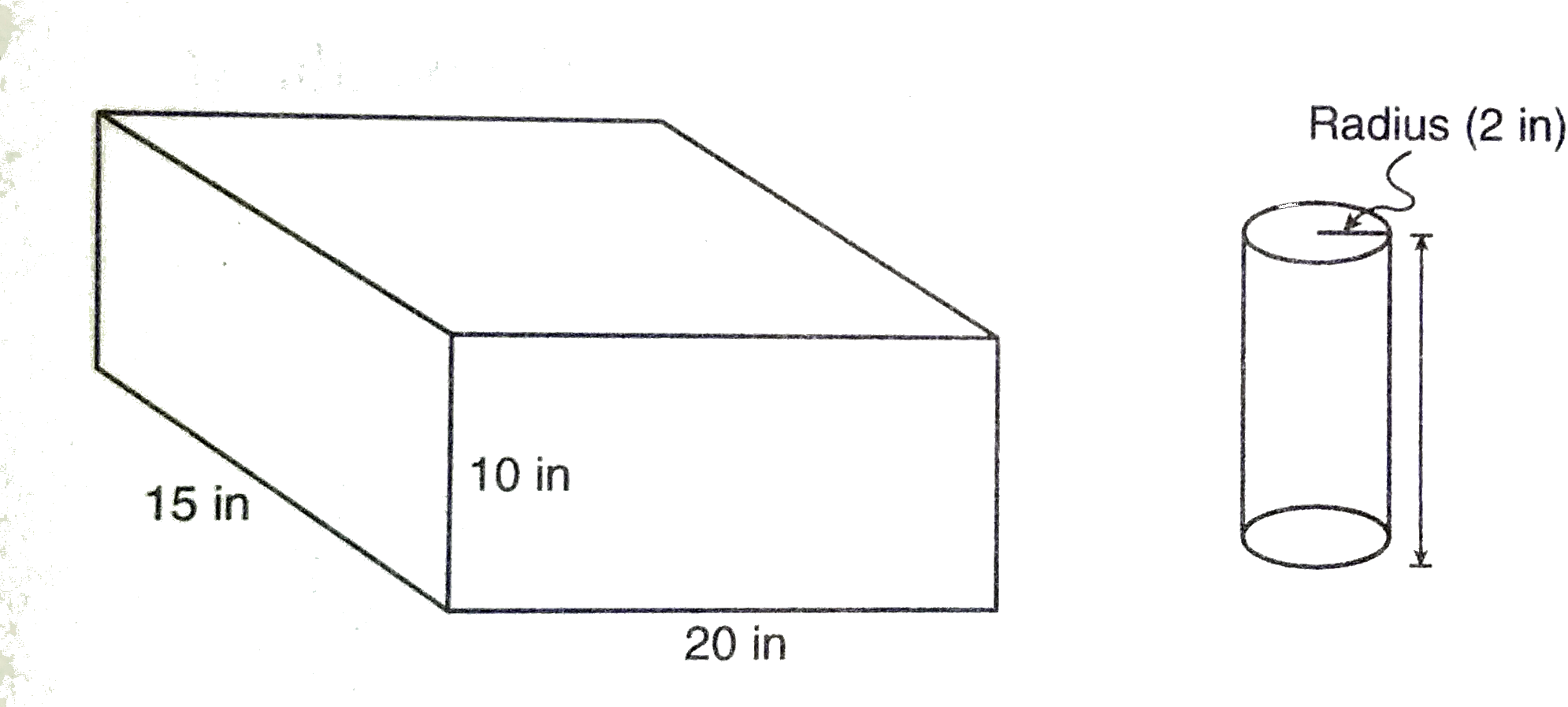
In the figure above, a rectangular container with the dimensions 10 inches by 15 inches by 20 inches is to be filled with water, using a cylindrical cup whose radius is 2 inches and whose height is 5 inches. What is the maximum number of full cups of water that can be placed into the container without the water overflowing the container?View Solution
Knowledge Check
- Question 1 - Select One
A storage facility is currently offering a special rate to customers who sign contracts for 6 months or more. According to this special rate, the first month's rent is $1, and for each month after the first month, customers pay the regular monthly rental rate. The table below shows the storage unit sizes avialble, the floor dimensions, and the regular monthly rental rate. All the units have the same heigher.

Size 5 units can be subdivided to form other sizes of units. What is the greatest number of Size 1 units that can be formed from a single size 5 unit?A2B4C8D16 - Question 2 - Select One
Tiny Exhibit, Big Impact
The first time I visited the Art Institute of Chicago, I expected to be impressed by its famous large paintings. (12) ul(" On one hand") , I couldn’t wait to view (13) painter, Georges Seurat’s–––––––––––––––––––––––––––, 10-foot-wide A Sunday Afternoon on the Island of La Grande Jatte in its full size. It took me by surprise, then, when my favorite exhibit at the museum was one of (14 ) it’s tiniest,–––––––––––– the Thorne Miniature Rooms.
Viewing the exhibit, I was amazed by the intricate details of some of the more ornately decorated rooms. I marveled at a replica of a salon (a formal living room) dating back to the reign of French king Louis XV. (15) Built into the dark paneled walls are bookshelves stocked with leather-bound volumes. The couch and chairs, in keeping with the style of the time, are characterized by elegantly curved arms and (16) legs, they–––––––––– are covered in luxurious velvet. A dime-sized portrait of a French aristocratic woman hangs in a golden frame.
This exhibit showcases sixty-eight miniature rooms inserted into a wall at eye level. Each furnished room consists of three walls, the fourth wall is a glass pane through which museumgoers observe. The rooms and their furnishings were painstakingly created to scale at 1/12th their actual size, so that one inch in the exhibit correlates with one foot in real life. A couch, for example, is seven inches long, and (17) that is based on a seven-foot-long couch–––––––––––––––––––––––––––––––––––––––––––––. Each room represents a distinctive style of European, American, or Asian interior design from the thirteenth to twentieth centuries.
The plainer rooms are more sparsely (18) furnished. Their–––––––––––––––––– architectural features, furnishings, and decorations are just as true to the periods they represent. One of my favorite rooms in the whole exhibit, in fact, is an 1885 summer kitchen. The room is simple but spacious, with a small sink and counter along one wall, a cast-iron wood stove and some hanging pots and pans against another wall, and (19) a small table under a window of the third wall––––––––––––––––––––––––––––––––––––––––––––––––––. Aside from a few simple wooden chairs placed near the edges of the room, the floor is open and obviously well worn.
As I walked through the exhibit, I overheard a 20 visitors’ remark, “You know, that grandfather clock actually runs. Its glass door swings open, and the clock can be wound up.” 21 Dotted with pin-sized knobs, another visitor noticed my fascination with a tiny writing desk and its drawers. “All of those little drawers pull out. And you see that hutch? Can you believe it has a secret compartment?” Given the exquisite craftsmanship and level of detail I’d already seen, I certainly could.ANO CHANGEBFor instance,CHowever,DSimilarly,
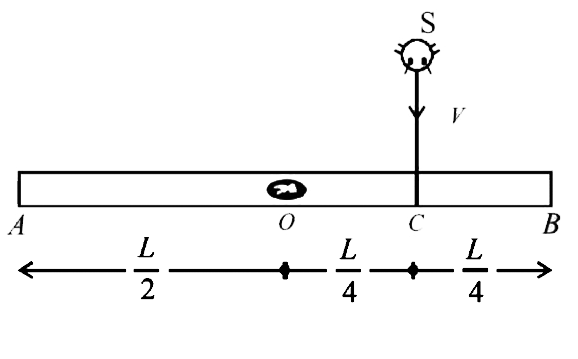
A thin uniform bar lies on a frictionless horizonta surface and is free to move in any way on th surface. Its mass is 0.16 kg and length √3meters. Two particless, each of mass 0.08 kg, are moving on the same surface and towards the bar in a direction perpendicular to the bar, one with a velocity of 10 m/s, and other with 6m/s as shown in fig. The first particle strikes the bar at point A and the other at point B. Points A and B are at a distane of 0.5m from the centre of the bar. The particles strike the bar at the same instant of time and stick to the bar on collision. Calculate the loss of the kinetic energy of the system in the above collision process.
 View Solution
View Solution_S01_071_Q01.png)
A glass full of water is placed on a weighing scale, which reds 10 N, A coin with weight 1 N is gently released into the water. At finrst the coin accelerates as it falls and about halfway down the glass, the coin reaches terminal velocity eventually the coin rests on te bottom of the glass, accelerationdue to gravity is 10m/s2. The scale roads
(a). 10.5N when the coin accelerates at 5m/s2)
(b). 11.5 N when the coin decelerates at 5m/s2
(c). 11N, when the coin moves with terminal velocity
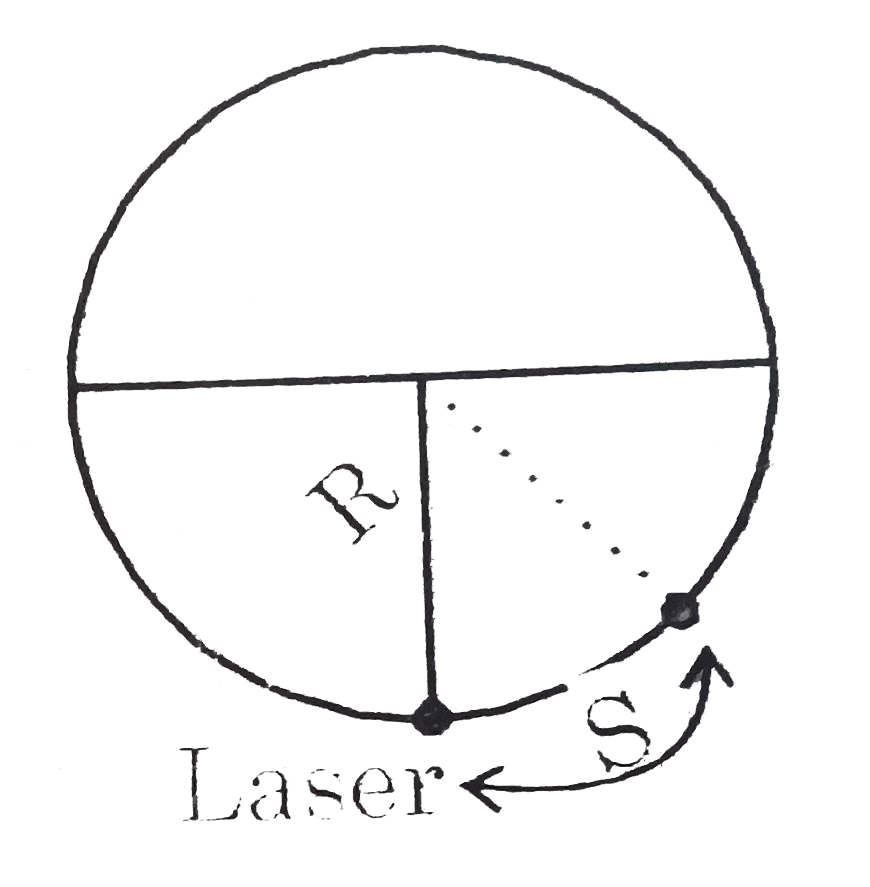
(d). 11N when the coin rests on the bottom.View SolutionA horizontal cylinderical tank 6πm in diameter is half full of water. The space above the water is filled with a pressurized gas of unknown refractive index. A small laser can move along the curved bottom of the water and aims a light beam towards the centre of the water surface. When the laser has moved a distance s=1m or more (measured from curved face) from the lowest point in water, no light enters the gas. The refractive index of gas is (μwater=4/3)
 View Solution
View SolutionAn elevator at rest which is at 10th floor of a building is having a plane mirror fixed to its floor. A particle is projected with a speed (√2)m/s and at 45∘ with the horizontal as shown in the figure. At the very instant of projection, the cable of the elevator breaks and the elevator starts falling freely. what will be the separation between the particles and is image 0.5 s after the instant of projection?
 View Solution
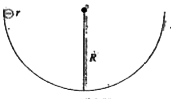
View SolutionThe radius of a large hemisphere whose" axis of symmetry is vertical is R = 50 cm. A small sphere of radius r= 5cm rolls without slipping with a speed of 2cm/s. The mass of the sphere is 100 gm. If it start's at the top from rest (i) what is the kinetic energy at the bottom? (ii) What fraction is rotational ? (iii) What fraction is translational?
 View Solution
View SolutionAn observer observer a fish moving upwards in a cylindrical container of cross section area 1m2 filled with water up to a height of 5m. A hole is present at the botton of the container having cross section areea 1/1000m2. Find out the speed of the image of fish observed by observer when the botton hole is just opened. (Given: The fish is moving with the speed of 6m/s towards the observer, μ of water =4/3)
View Solution

