Question

The radius of a large hemisphere whose" axis of symmetry is vertical is R = 50 cm. A small sphere of radius r= 5cm rolls without slipping with a speed of 2cm/s. The mass of the sphere is 100 gm. If it start's at the top from rest (i) what is the kinetic energy at the bottom? (ii) What fraction is rotational ? (iii) What fraction is translational?

Explanation

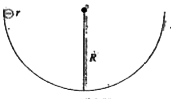
Radius of the small sphere = `R = 0.5 m`
Radius of the small sphere =r = 0.05 m
Mass of small sphere m=0.100 kg
Speed = v = 0.02 m/s
Translational kinetic energy `=K_(r) =1/2 mv^(2)`
Rotational kinetic energy `=K_(r) = 1/2 Iomega^(2)`
`=1/2 xx (2/5 mr^(2)) xx v^(2)/r^(2) = 1/5 mv^(2)`
Total K.E. of the small sphere `=K = K_(l) + K_(r) = 1/2 mv^(2) + 1/5 mv^(2)`
`K = 7/10 mv^(2) = 7/10 xx 0.100 xx (0.02)^(2)`
`K = 2.8 xx 10^(-5) J`
(ii) `K_(r)/K =((1//5)mv^(2))/((7//10) mv^(2)) = 2/7`
`(2/7)` of the total energy is rotational
`K_(t)/K =((1/2)mv^(2))/((7//10)mv^(2)) = 5/7`
`(5/7)` of the total energy is translational.