In the above figure both RISK and CLUE are parallelograms. Find the value of x.
To find the value of x in the given problem involving the parallelograms RISK and CLUE, we can follow these steps:
1. Identify the Properties of Parallelograms:
- In a parallelogram, opposite angles are equal, and adjacent angles are supplementary (i.e., they add up to 180 degrees).
2. Analyze Parallelogram RISK:
- Let’s denote the angles of parallelogram RISK as follows:
- ∠K=120∘
- ∠S is adjacent to ∠K.
- According to the property of parallelograms, we have:
∠K+∠S=180∘
- Substituting the value of ∠K:
120∘+∠S=180∘
- Solving for ∠S:
∠S=180∘−120∘=60∘
3. Analyze Parallelogram CLUE:
- Let’s denote the angles of parallelogram CLUE:
- ∠E=∠L
- Given that ∠L=70∘, we have:
∠E=70∘
4. Use the Triangle Angle Sum Property:
- In triangle ESO, the angles are ∠E, ∠S, and x. According to the angle sum property of triangles:
∠E+∠S+x=180∘
- Substituting the known values:
70∘+60∘+x=180∘
5. Solve for x:
- Combine the known angles:
130∘+x=180∘
- Isolate x:
x=180∘−130∘=50∘
Final Answer:
The value of x is 50∘.
---
- In a parallelogram, opposite angles are equal, and adjacent angles are supplementary (i.e., they add up to 180 degrees).
- Let’s denote the angles of parallelogram RISK as follows:
- ∠K=120∘
- ∠S is adjacent to ∠K.
- According to the property of parallelograms, we have:
∠K+∠S=180∘
- Substituting the value of ∠K:
120∘+∠S=180∘
- Solving for ∠S:
∠S=180∘−120∘=60∘
- Let’s denote the angles of parallelogram CLUE:
- ∠E=∠L
- Given that ∠L=70∘, we have:
∠E=70∘
- In triangle ESO, the angles are ∠E, ∠S, and x. According to the angle sum property of triangles:
∠E+∠S+x=180∘
- Substituting the known values:
70∘+60∘+x=180∘
- Combine the known angles:
130∘+x=180∘
- Isolate x:
x=180∘−130∘=50∘
The value of x is 50∘.
Topper's Solved these Questions
- UNDERSTANDING QUADRILATERALSBOOK - NCERT ENGLISHCHAPTER - UNDERSTANDING QUADRILATERALSEXERCISE - EXERCISE 3.2
6 Videos
- SQUARES AND SQUARE ROOTSBOOK - NCERT ENGLISHCHAPTER - SQUARES AND SQUARE ROOTSEXERCISE - EXERCISE 6.4
9 Videos
- VISUALISING SOLID SHAPESBOOK - NCERT ENGLISHCHAPTER - VISUALISING SOLID SHAPESEXERCISE - EXERCISE 10.3
8 Videos
Similar Questions
Knowledge Check
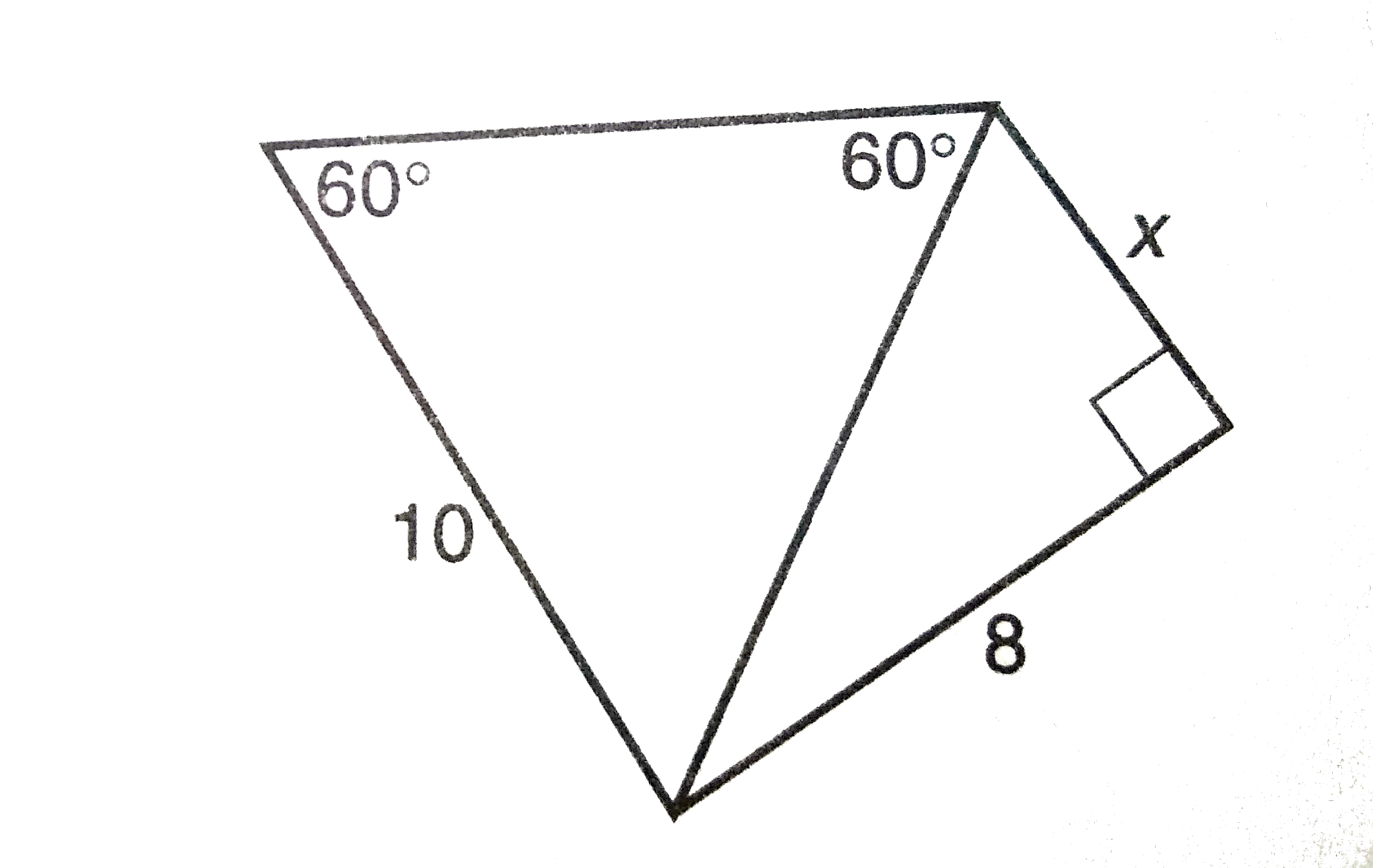
In the figure above, x=A4B6C4√2D4√3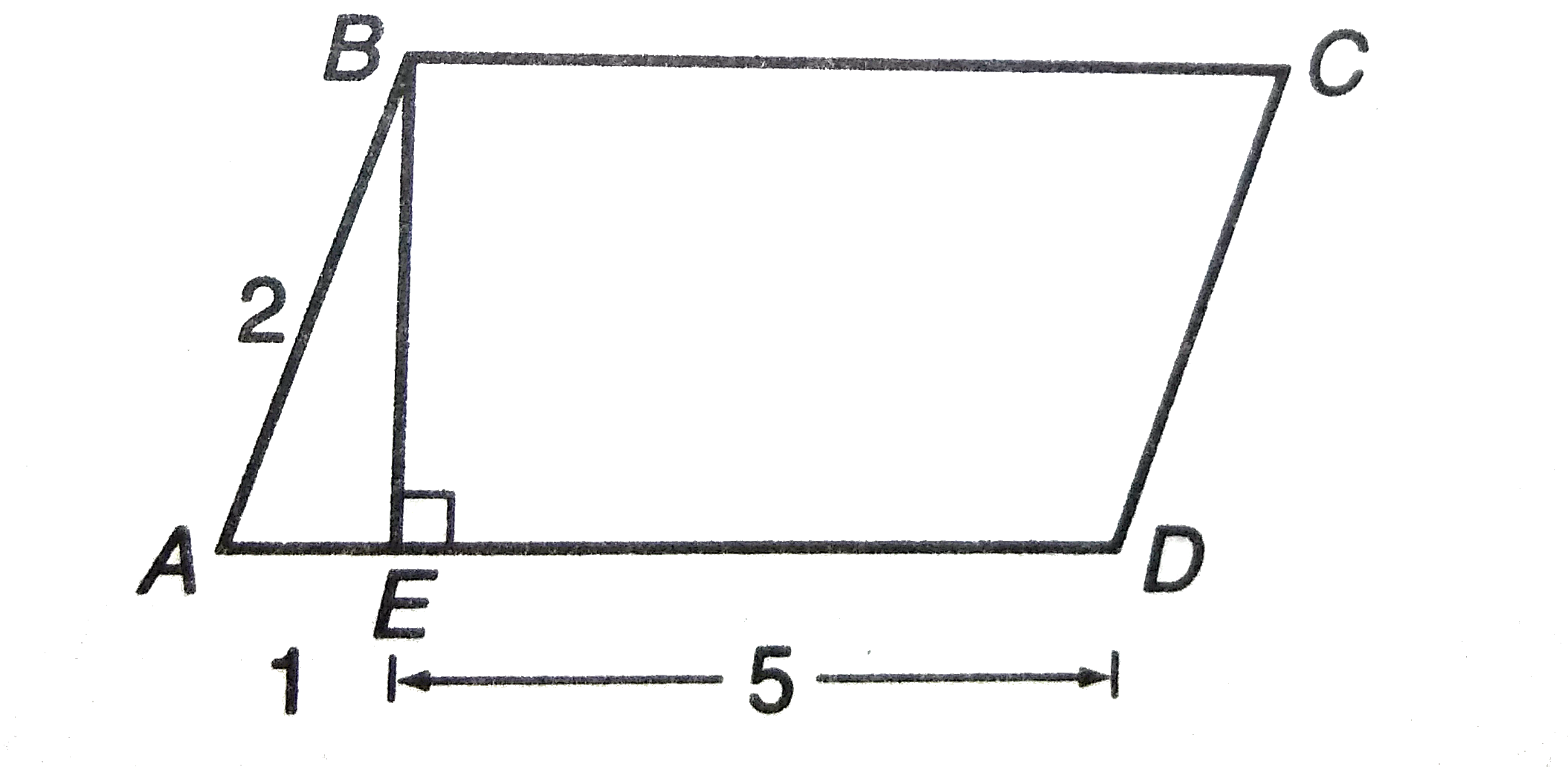
In the figure above, what is the area of parallelogram ABCD?A4√2B4√3C6√2D6√3
In the given figure, ABCD is a parallelogram. Find the values of x, y and z
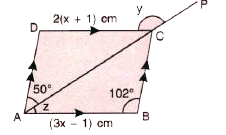
View SolutionIn the adjacent figure HOPE is a parallelogram. Find the angle measures x, y and Z. State the geometrical truths you use to find them. (Figure)
View SolutionIn Figure, BEST is a parallelogram. Find the values of x, y and z.
View SolutionIn the given figure, ABCD is a parallelogram. Find the area of ΔAED
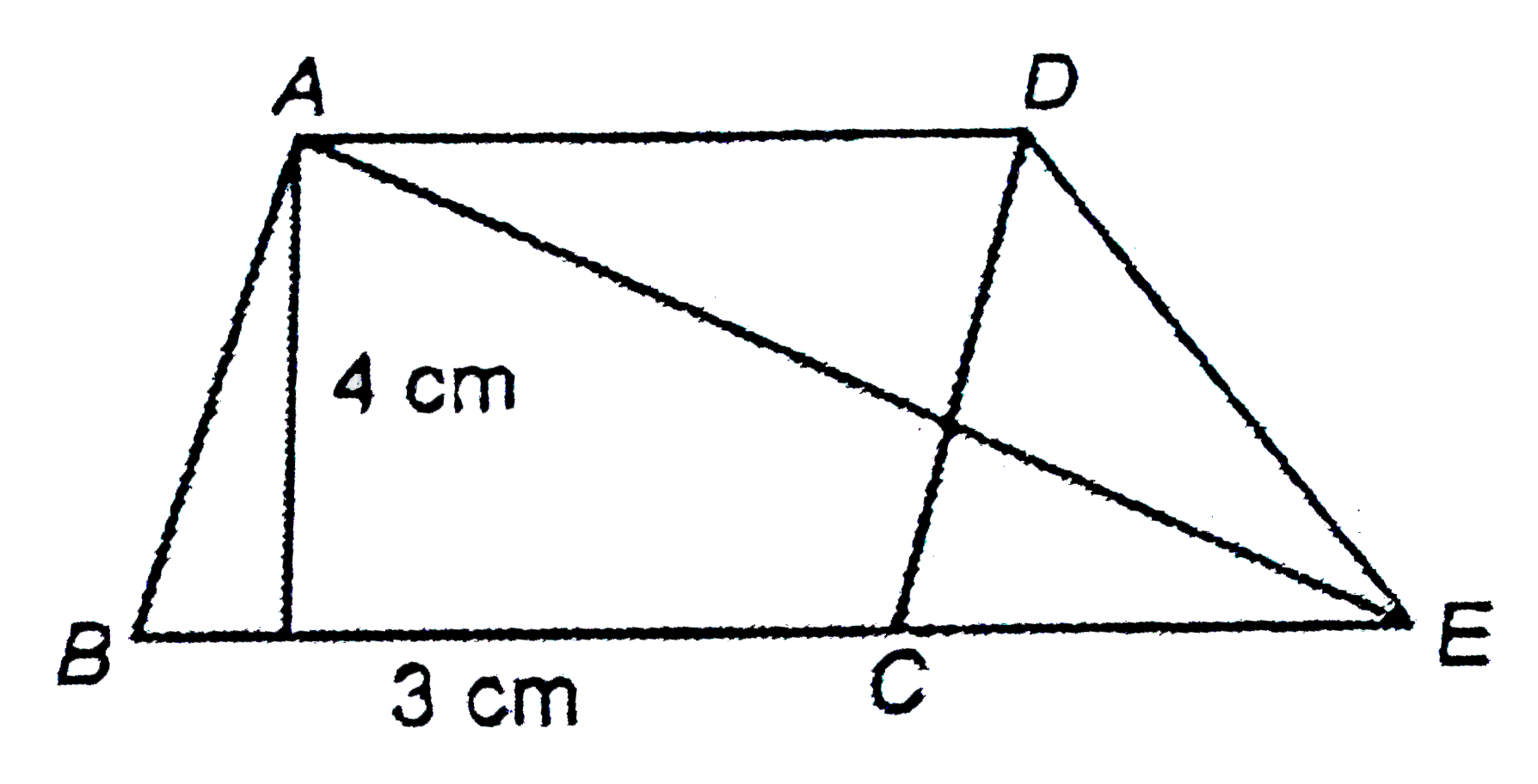
View SolutionIn the following figures GUNS and RUNS are parallelograms. FinD x and y . (Figures)
View SolutionIn Fig 3.26, BEST is a parallelogram. Find the values x, y and z.
View Solution
