Is a square prism same as a cube? Explain.
The correct Answer is:No, a square prism does not have to necessarily be a cube, as a square prism has a square base but the height need not be of the same dimension.
No, a square prism does not have to necessarily be a cube, as a square prism has a square base but the height need not be of the same dimension.
Topper's Solved these Questions
Similar Questions
Area of a square is same as that of a rectangle. The length and the breadth of the rectangle are respectively 5 cm more and 4 cm less than the side of the square. Find the side of the square.
View SolutionThe are of a square is the same as that of a rectangle. If the side of a square is 60 m and the breadth of the rectangle is 48 m, find the length of the rectangle.
View SolutionExplain the difference between a square pyramid and a square prism.
View SolutionIf square of x varies as cube of y and x=3 when y=4, the value of y at 1√3 will be..
View SolutionThe square on the diagonal of a cube has an area of 192cm2Calculate :
the side of the cube.
View SolutionThe square on the diagonal of a cube has an area of 192cm2Calculate :
the total surface area of the cube.
View SolutionThe square on the diagonal of a cube has an area of 1875 sq. cm. Calculate:
the side of the cube
View SolutionThe square on the diagonal of a cube has an area of 1875 sq. cm. Calculate:
the total surface area of the cube.
View SolutionA candidate in order to study the process of osmosis has taken 3 potato cubes and put them in 3 different beakers containing 3 different solutions. After 24 hours, in the first beaker, the potato cube increased in size, in the second beaker, the potato cube decreased in size and in the third beaker there was no change in the size of the potato cube. The following diagram shows the result of the same experiment :
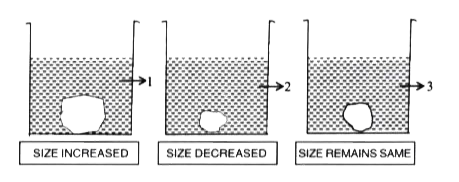
In beaker 3, the size of the potato cube remains the same. Explain the reason in brief.
View SolutionThe side of a square is 6cm. A rectangle has the same perimeter as that of the square.
Find the area of the square.
View Solution