In ΔPQR, D is the mid-point of ¯¯¯¯¯¯QR.then¯¯¯¯¯¯¯PM is ___________,PD is___________. Is QM=MR?
To solve the problem step by step, we will analyze the triangle ΔPQR where D is the midpoint of ¯¯¯¯¯¯¯¯¯QR.
Step 1: Identify the properties of PM
Since D is the midpoint of ¯¯¯¯¯¯¯¯¯QR, we can conclude that PM is perpendicular to QR. This means that PM acts as the height (or altitude) from point P to line QR.
Hint: Remember that the altitude of a triangle is a line segment from a vertex perpendicular to the opposite side.
Step 2: Identify the properties of PD
Since D is the midpoint of ¯¯¯¯¯¯¯¯¯QR, the line segment PD connects vertex P to the midpoint D of side QR. This line segment is known as a median of the triangle.
Hint: A median of a triangle is a line segment joining a vertex to the midpoint of the opposite side.
Step 3: Determine if QM=MR
To check if QM is equal to MR, we can use the property of midpoints. Since D is the midpoint of QR, we have:
QD=DR
This implies that QD is equal to DR. We can express QD in terms of QM and MD:
QD=QM+MD
Similarly, we can express DR in terms of MR and MD:
DR=MR−MD
Since QD=DR, we can set the two expressions equal to each other:
QM+MD=MR−MD
Step 4: Rearranging the equation
Now, we can rearrange the equation to isolate QM:
QM+MD+MD=MR
QM+2MD=MR
This shows that:
QM=MR−2MD
Step 5: Conclusion
Since MR is greater than 2MD (because MD is a positive length), we can conclude that:
QM≠MR
Thus, QM is not equal to MR.
Final Answers:
- PM is the altitude.
- PD is the median.
- QM is not equal to MR.
Since D is the midpoint of ¯¯¯¯¯¯¯¯¯QR, we can conclude that PM is perpendicular to QR. This means that PM acts as the height (or altitude) from point P to line QR.
Since D is the midpoint of ¯¯¯¯¯¯¯¯¯QR, the line segment PD connects vertex P to the midpoint D of side QR. This line segment is known as a median of the triangle.
To check if QM is equal to MR, we can use the property of midpoints. Since D is the midpoint of QR, we have:
Now, we can rearrange the equation to isolate QM:
QM+2MD=MR
Since MR is greater than 2MD (because MD is a positive length), we can conclude that:
- PM is the altitude.
- PD is the median.
- QM is not equal to MR.
Topper's Solved these Questions
- BOOK - NCERT ENGLISHCHAPTER - THE TRIANGLE AND ITS PROPERTIESEXERCISE - EXERCISE 6.22videos
THE TRIANGLE AND ITS PROPERTIES
- BOOK - NCERT ENGLISHCHAPTER - THE TRIANGLE AND ITS PROPERTIESEXERCISE - EXERCISE 6.58videos
THE TRIANGLE AND ITS PROPERTIES
- BOOK - NCERT ENGLISHCHAPTER - SYMMETRYEXERCISE - EXERCISE 14.22videos
SYMMETRY
Similar Questions
Knowledge Check
- Question 1 - Select One
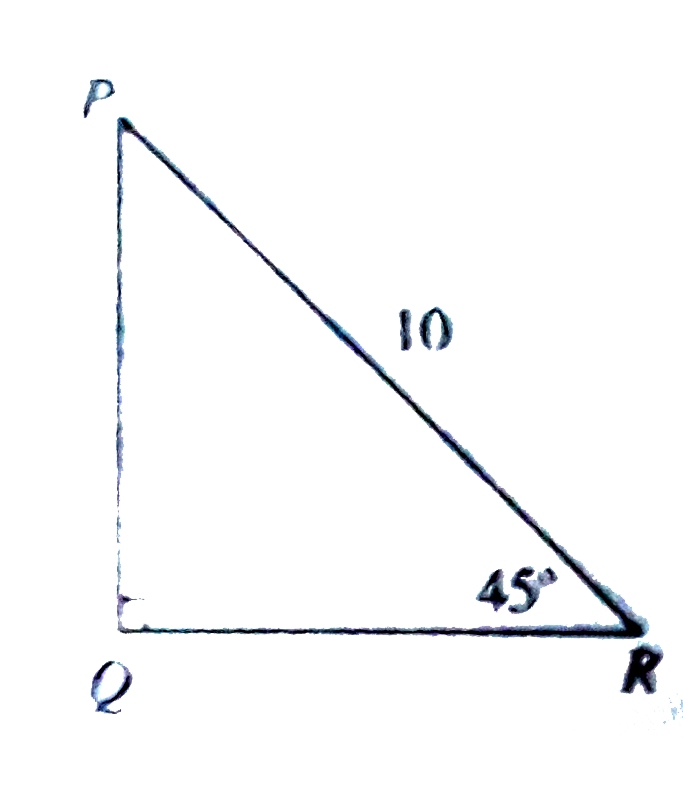
What is the length of ¯¯¯¯¯¯QR ?A5B5√22C10√2D5√2 - Question 2 - Select One
Given right triangle ΔPQR below, what is the length of ¯¯¯¯¯¯PQ?
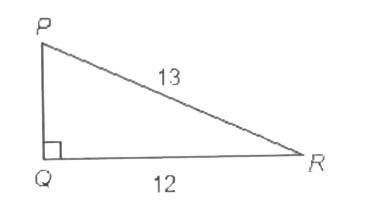 A√2B√5C5D7
A√2B√5C5D7 - Question 3 - Select One
The points P,Q,R and S lie in that order on a straight line . The midpoint of ¯¯¯¯¯¯QS is R and the midpoint of ¯¯¯¯¯PS is Q . The length of ¯¯¯¯¯¯QR is x feet and the length of ¯¯¯¯¯¯PQ is 4x−16 feet . What is the length , in feet , of ¯¯¯¯¯PS?
A32B20C16D8
In ΔPQR, MN is parallel to QR and PMMQ=23
(i) Find MNQR
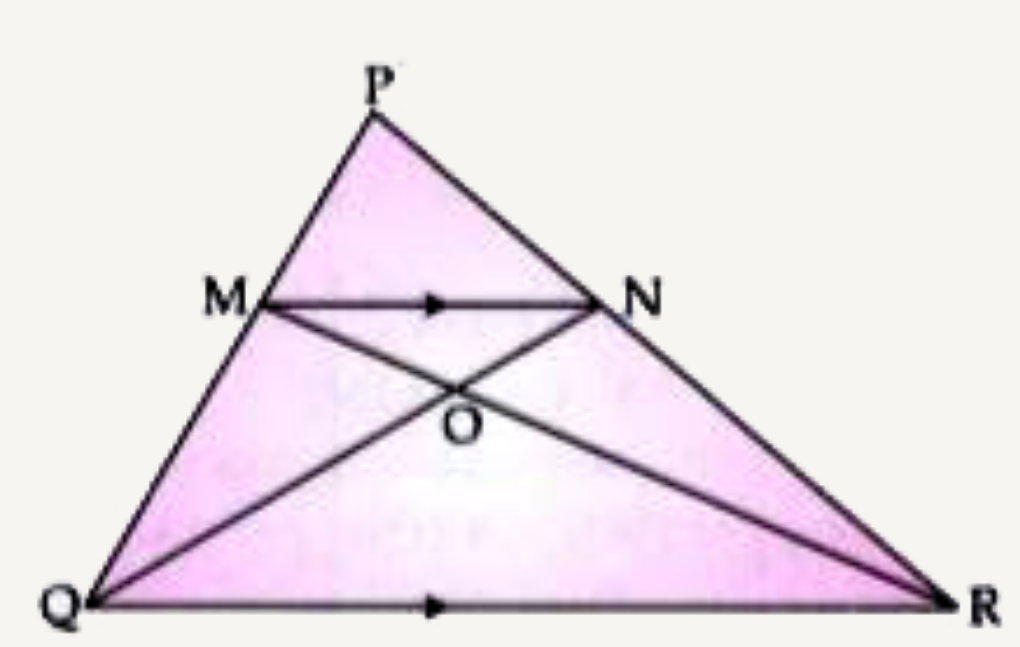 View Solution
View SolutionIn ΔPQR,Pq=PR and S is the mid-point of PQ. A line drawn from S parallel to QR, intersects the line PR at T. Prove that PS = PT.
View SolutionA, B, C, and D all lie on a number line. C is the midpoint of ¯¯¯¯¯¯AB and D is the midpoint of ¯¯¯¯¯¯AC.
Quantity AQuantity BThe ratio of ¯¯¯¯¯¯AD to ¯¯¯¯¯¯CBThe ratio of ¯¯¯¯¯¯AC to ¯¯¯¯¯¯ABView SolutionIn the figure below, ABCD is a rectangle, EFGH is a square, and ¯¯¯¯¯¯CD is a diameter of a semicircle. Point K is the midpoint of ¯¯¯¯¯¯CD. Point J is the midpoint of both ¯¯¯¯¯¯AB and ¯¯¯¯¯¯EF. Points E and F lie on ¯¯¯¯¯¯AB. The 3 given lengths are in meters.
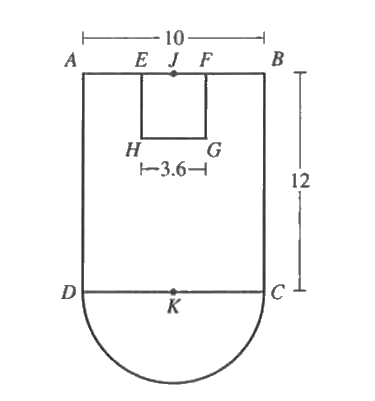
What is the length, in meters, of are ¯¯¯¯¯¯CD?View SolutionIn the figure below, ABCD is a rectangle, EFGH is a square, and ¯¯¯¯¯¯CD is a diameter of a semicircle. Point K is the midpoint of ¯¯¯¯¯¯CD. Point J is the midpoint of both ¯¯¯¯¯¯AB and ¯¯¯¯¯¯EF. Points E and F lie on ¯¯¯¯¯¯AB. The 3 given lengths are in meters.
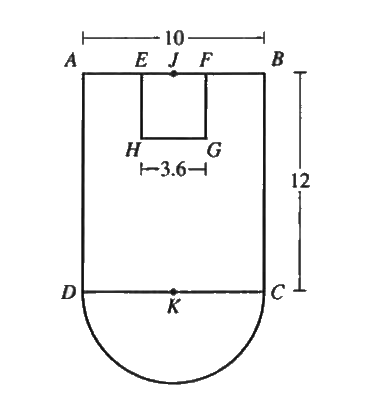
What is the length, in meters, of ¯¯¯¯¯¯JD?View Solution

