Find the value of the unknown interior angle x in the following figures:
To find the value of the unknown interior angle x in the given triangle, we will follow these steps:
1. Identify the Given Angles:
- Angle BAC=50∘
- Angle CBA=x (unknown)
- External angle ACD=115∘
2. Use the Exterior Angle Theorem:
- According to the Exterior Angle Theorem, the exterior angle ACD is equal to the sum of the two opposite interior angles BAC and CBA.
- This can be written as:
ACD=BAC+CBA
3. Substitute the Known Values:
- Substitute the known values into the equation:
115∘=50∘+x
4. Solve for x:
- Rearranging the equation to isolate x:
x=115∘−50∘
- Now, perform the subtraction:
x=65∘
5. Conclusion:
- The value of the unknown interior angle x is 65∘.
Final Answer:
The value of the unknown interior angle x is 65∘.
---
- Angle BAC=50∘
- Angle CBA=x (unknown)
- External angle ACD=115∘
- According to the Exterior Angle Theorem, the exterior angle ACD is equal to the sum of the two opposite interior angles BAC and CBA.
- This can be written as:
ACD=BAC+CBA
- Substitute the known values into the equation:
115∘=50∘+x
- Rearranging the equation to isolate x:
x=115∘−50∘
- Now, perform the subtraction:
x=65∘
- The value of the unknown interior angle x is 65∘.
The value of the unknown interior angle x is 65∘.
Topper's Solved these Questions
Similar Questions
Find the value of the unknown exterior angle x in the following diagrams:
View SolutionFind the values of the unknowns x and y in the following diagrams

View SolutionFind the value of x in the following figure:
View SolutionFind the unknown marked angles in each of the following figures :
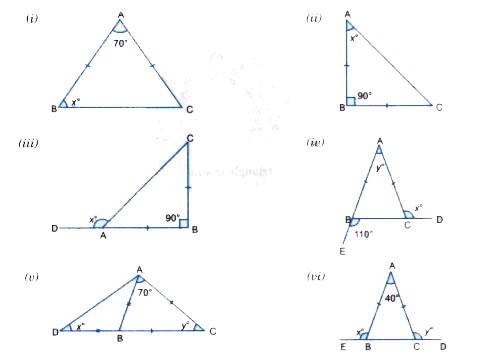
View SolutionFind the unknown angles marked in each of the following figures :
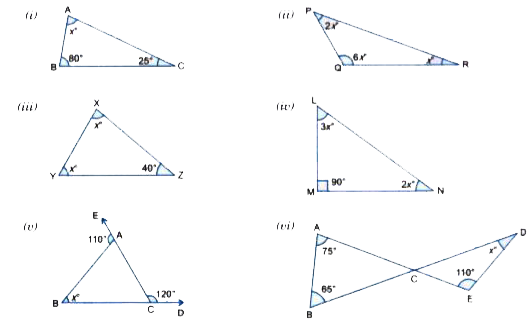
View SolutionFind the angle measure x in the following figures.
View SolutionFind the value of the unknown x in the following diagrams:
View SolutionFind the value of x in the figure.

View SolutionFind the value of the 'x' in the following figure:
View SolutionFind the values of the angles x, y, and z in each of the following:
View Solution
