In a chamber, a uniform magnetic field of 6⋅5G(1G=10−4T) is maintained. An electron is shot into the field with a speed of 4⋅8×106ms−1 normal to the field. (i) Explain why the path of the electron is a circle. Determine the radius of the circular orbit. (e=1⋅6×10−19C,me=9⋅1×10−31kg).
To solve the problem step-by-step, we will first explain why the path of the electron is circular and then calculate the radius of the circular orbit.
Step 1: Explain why the path of the electron is circular
When a charged particle, like an electron, moves through a magnetic field, it experiences a force known as the Lorentz force. This force is given by the equation:
F=q(v×B)
Where:
- F is the Lorentz force,
- q is the charge of the particle,
- v is the velocity of the particle,
- B is the magnetic field.
In this case, the electron is shot into the magnetic field with a velocity that is normal (perpendicular) to the magnetic field. The key points are:
- The Lorentz force acts perpendicular to both the velocity of the electron and the magnetic field.
- Since the force is always directed towards the center of the circular path (perpendicular to the velocity), it causes the electron to move in a circular path.
Thus, the path of the electron is circular due to the continuous perpendicular force acting on it.
Step 2: Determine the radius of the circular orbit
To find the radius of the circular orbit, we can equate the magnetic force to the centripetal force required to keep the electron in circular motion. The magnetic force (F) is given by:
F=qvB
The centripetal force (Fc) required to keep the electron in circular motion is given by:
Fc=mv2r
Where:
- m is the mass of the electron,
- v is the velocity of the electron,
- r is the radius of the circular path.
Setting the magnetic force equal to the centripetal force, we have:
qvB=mv2r
From this equation, we can solve for the radius r:
r=mvqB
Step 3: Substitute the values
Now we will substitute the known values into the equation:
- Charge of the electron, q=1.6×10−19C
- Mass of the electron, m=9.1×10−31kg
- Velocity of the electron, v=4.8×106m/s
- Magnetic field, B=6.5G=6.5×10−4T
Substituting these values into the formula for r:
r=(9.1×10−31kg)×(4.8×106m/s)(1.6×10−19C)×(6.5×10−4T)
Step 4: Calculate the radius
Calculating the numerator:
9.1×10−31×4.8×106=4.368×10−24kg⋅m/s
Calculating the denominator:
1.6×10−19×6.5×10−4=1.04×10−22C⋅T
Now, substituting these values back into the equation for r:
r=4.368×10−241.04×10−22≈0.042m
Thus, the radius of the circular orbit is approximately:
r≈4.2×10−2m
Final Answer
The radius of the circular orbit of the electron is approximately 0.042m or 4.2cm.
---
- F is the Lorentz force,
- q is the charge of the particle,
- v is the velocity of the particle,
- B is the magnetic field.
- Since the force is always directed towards the center of the circular path (perpendicular to the velocity), it causes the electron to move in a circular path.
- m is the mass of the electron,
- v is the velocity of the electron,
- r is the radius of the circular path.
- Mass of the electron, m=9.1×10−31kg
- Velocity of the electron, v=4.8×106m/s
- Magnetic field, B=6.5G=6.5×10−4T
Topper's Solved these Questions
Similar Questions
De - Broglie wavelength of an electron accelerated by a voltage of 50 V is close to
(|e|=1.6×10−19C,me=9.1×10−31)
View SolutionThe speed of the electron in the hydrogen atom is approximately 2.2×106m/s. What is the centripetal force acting on the electron ifthe radius of the circular orbit is 0.53×10−10m?Mass of the electron is 9.1×10−31kg
View Solution
Knowledge Check
An electron is moving in a cyclotron at a speed of 3.2×107ms−1 in a magnetic field of 5×10−4T perpendicular to it. What is the frequency of this electron? (q=1.6×10−19C,mc=9.1×10−31kg)
A1.4×105HzB1.4×107HzC1.4×106HzD1.4×109HzAn electron of energy 1800 eV describes a circular path in magnetic field of flux density 0.4 T. The radius of path is (q=1.6×10−19C,me=9.1×10−31kg)
A2.58×10−4mB3.58×10−4mC2.58×10−3mD3.58×10−4m
In an atom, an electron is moving with a speed of 600m/s with an accuracy of 0.005% . Certainty with which the position of the electron can be localized is :
(h=6.6×10−34kgm2s−1 ,
mass of electron (em)=9.1×10−31kg).
View SolutionA proton of mass 1.67×10−27 kg and charge 1.6×10−19C is shot a uniform magnetic field, and perpendicular to the field with a velocity 5×106m/s. If the magnetic induction of the field is 1 tesla, find
(i) The radius of the circular path
View SolutionIf de Broglie wavelength of an electron is 0.5467 Å,
find the kinetic energy of electron in eV. Given h=6.6×10−34 Js ,
e=1.6×10−19 C, me=9.11×10−31 kg.
View SolutionAn α-particle of mass 6.65×10−27kg is travelling at right angles to a magnetic field with a speed of 6×105ms−1. The strength of the magnetic field is 0.2T. Calculate the force on the α-particle and its acceleration.
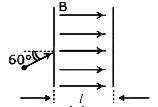
View SolutionThe figure shows a region of length ‘l’ with a uniform magnetic field of 0.3 T in it and a proton entering the region with velocity 4×105 ms−1 making an angle 60∘ with the field. If the proton completes 10 revolution by the time it cross the region shown, ‘l’ is close to (mass of proton =1.67×10−27 kg , charge of the proton =1.6×10−19 C )
View SolutionAn electron revolves in a circule at the rate of 1019 rounds per second. The rquivlent current is (e=1.6×10−19C)
View Solution
