According to early model of an atom,the atom is considered it to have a positively charged point nucleus of charge Ze surrounded by a uniform density of negative charge up to a radius R. The atom as a whole is neutral. The electric field at a distancer from the nucleus is (r<R)
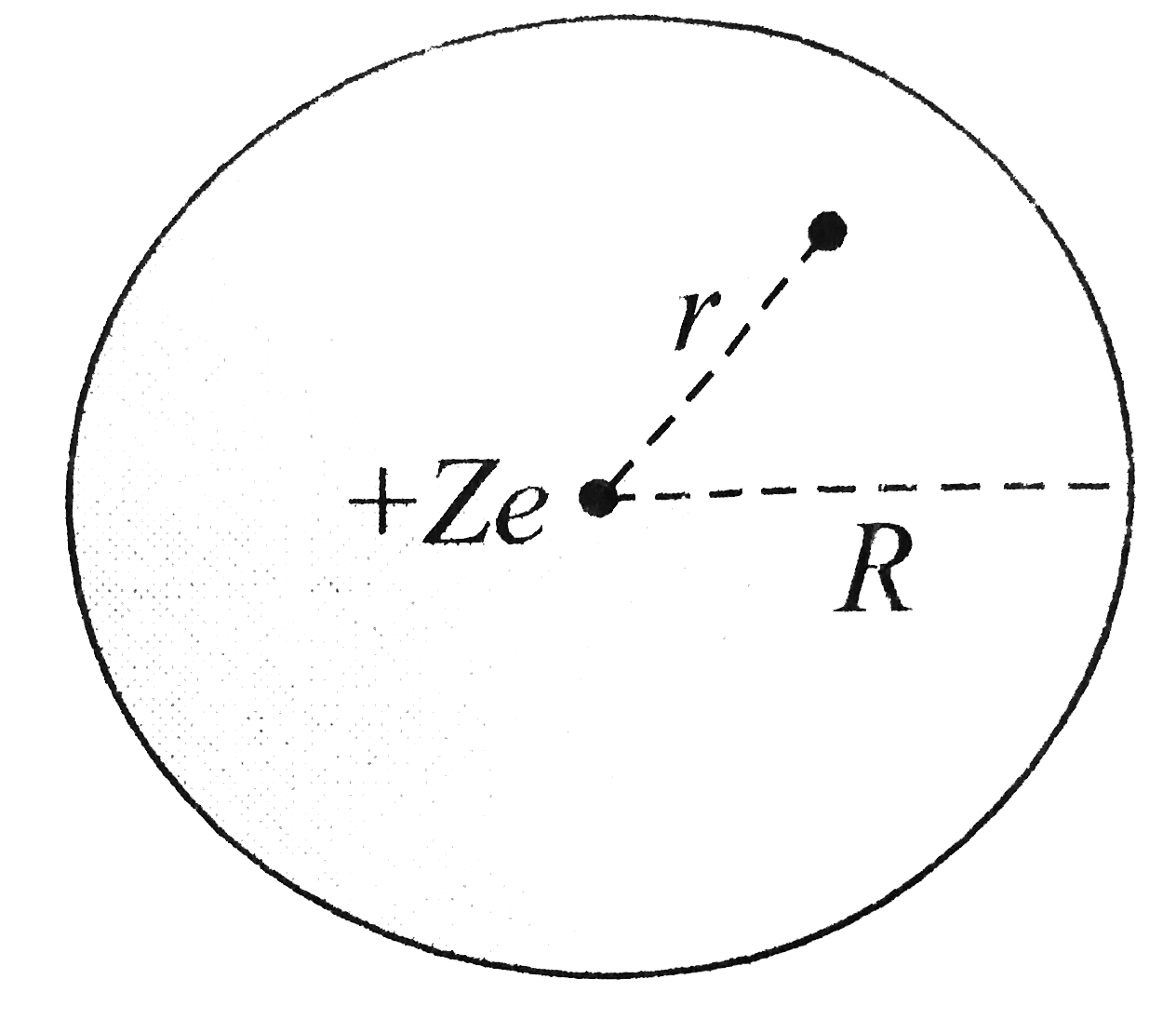
The charge distribution for this model of the atom is as shown in Fig. 1.32. The total negative charge in the uniform spherical charge distribution of radius R must be –Z e, since the atom (nucleus) of charge Z e + negative charge) is neutral. This immediately gives us the negative charge density r, since we must have
4πR23ρ=0−Ze
4πR23ρ=0−Ze
orρ=−3Ze4πR2 To find the electric field E(r) at a point P which is a distance r away from the nucleus, we use Gauss’s law. Because of the spherical symmetry of the charge distribution, the magnitude of the electric field E(r) depends only on the radial distance, no matter what the direction of r. Its direction is along (or opposite to) the radius vector r from the origin to the point P. The obvious Gaussian surface is a spherical surface centred at the nucleus. We consider two situations, namely, r<Randr>R.
(i) r<R: The electric flux f enclosed by the spherical surface is
ϕ=E(r)×4πr2
where E(r)is the magnitude of the electric field at r. This is beacuse the field at any point on the spherical Gaussian surface has the same direction as the normal to the surface there, and has the same magnitude at all points on the surface. The charge q enclosed by the Gaussian surface is the positive nuclear charge and the negative charge within the sphere of radius r, i.e.q=ze+4πr33ρ
Substituting for the charge density ρ obtained earlier, we have
q=Ze−Zer3R3
Gauss's law then gives.
E(r)=Ze4πε01r2−rR3:r<Ra
The electric field is directed radially outward.
(ii) r>R: In this case, the total charge enclosed by the Gaussian spherical surface is zero since the atom is neutral. Thus, from Gauss’s law,
E(r)×4πr2=0orE(r)=0:r>R
At r=R, both cases give the same result E=0
Topper's Solved these Questions
- ELECTRIC CHARGES AND FIELDSBOOK - NCERT ENGLISHCHAPTER - ELECTRIC CHARGES AND FIELDSEXERCISE - Exercise
34 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - NCERT ENGLISHCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - All Questions
32 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - NCERT ENGLISHCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise
17 Videos
Similar Questions
An early model for an atom considered it to have a positively charged point nucleus of charge Ze, surrounded by a uniform density of negative charge upto a radius R. The atom as a whole is neutral. The electric field at a distance r from the nucleus is (r < R)

View SolutionConsider a uniformly charged ring of radius R. Find the point on the axis where the electric field is maximum.
View Solution
Knowledge Check
There is a solid sphere of radius R having uniformly distributed charge throughout it. What is the relation between electric field E and distance r from the centre (r is less than R) ?
AE∝r−2BE∝r−1CE∝rDE∝r2
A hallow metal sphere of radius R is uniformly charged. The electric field due to the sphere at a distance r from the centre:
View SolutionA conducting sphere of radius R is charged to a potential of V volts. Then the electric field at a distance r(>R) from the centre of the sphere would be
View SolutionConsider an atom with atomic number Z as consisting of a positive point charge at the centre and surrounded by a distribution of negative electricity uniformly distributed within a sphere of radius R. The electric field at a point inside the atom at a distance r from the centre is
View SolutionA long thin flat sheet has a uniform surface charge density σ. The magnitude of the electric field at a distance .r. from it is given by
View SolutionA point charge q is placed at the origin . How does the electric field due to the charge very with distance r from the origin ?
View SolutionA ring of radius R is placed in the plane its centre at origin and its axis along the x-asis and having uniformly distributed positive charge. A ring of radius r(<<R) and coaxial with the larger ring is moving along the axis with constant velcity , then the variation of electrical flux (ϕ) passing through the smaller ring with position will be best represent by :
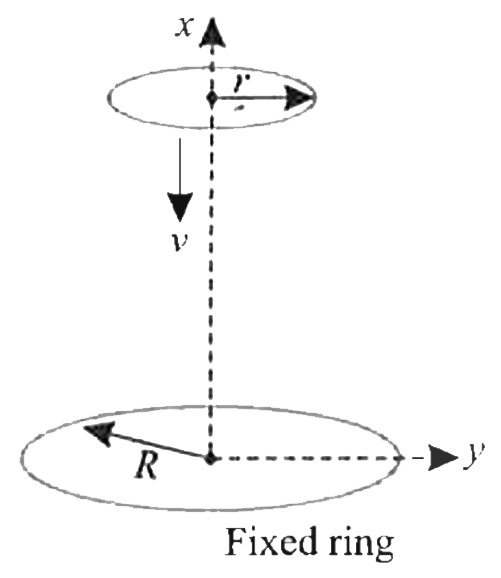
View SolutionIf the potential at the centre of a uniformly charged hollow sphere of radius R is V, then electric field at a distance r from the centre of sphere will be (r>R).

View Solution
