An electric iron of resistance 20Ω takes a current of 5A. Calculate the heat developed in 30s.
To solve the problem of calculating the heat developed by an electric iron with a resistance of 20 ohms that takes a current of 5 A over a period of 30 seconds, we can follow these steps:
Step 1: Identify the given values
- Resistance (R) = 20 Ω (ohms)
- Current (I) = 5 A (amperes)
- Time (T) = 30 s (seconds)
Step 2: Use the formula for heat developed
The heat developed (H) in an electrical circuit can be calculated using the formula:
H=I2×R×T
where:
- H = heat developed (in joules)
- I = current (in amperes)
- R = resistance (in ohms)
- T = time (in seconds)
Step 3: Substitute the values into the formula
Now, we will substitute the known values into the formula:
H=(5A)2×(20Ω)×(30s)
Step 4: Calculate I2
First, calculate I2:
I2=52=25
Step 5: Substitute I2 into the formula
Now substitute I2 back into the heat formula:
H=25×20×30
Step 6: Perform the multiplication
Now, calculate the product:
1. Calculate 25×20=500
2. Then, calculate 500×30=15000
Step 7: Conclusion
Thus, the heat developed in 30 seconds is:
H=15000J
Final Answer:
The heat developed in 30 seconds is 15000 joules.
---
- Resistance (R) = 20 Ω (ohms)
- Current (I) = 5 A (amperes)
- Time (T) = 30 s (seconds)
The heat developed (H) in an electrical circuit can be calculated using the formula:
H=I2×R×T
where:
- H = heat developed (in joules)
- I = current (in amperes)
- R = resistance (in ohms)
- T = time (in seconds)
Now, we will substitute the known values into the formula:
H=(5A)2×(20Ω)×(30s)
First, calculate I2:
I2=52=25
Now substitute I2 back into the heat formula:
H=25×20×30
Now, calculate the product:
1. Calculate 25×20=500
2. Then, calculate 500×30=15000
Thus, the heat developed in 30 seconds is:
H=15000J
The heat developed in 30 seconds is 15000 joules.
Topper's Solved these Questions
Similar Questions
An electric bulb of resistance 500Ω, draws a current of 0.4 A. Calculate the power of the bulb and the potential difference at its end.
View SolutionAn electric heater of resistance 8Ω draws 15A from the service mains for 2 hours. Calculate the rate at which heat is developed in the heater.
View SolutionAn ammeter of resistance 0.6Ω can measure current upto 1.0 A. Calculate (i) The shunt resistance required to enable the ammeter to measure current upto 5.0 A (ii) The combined resistance of the ammeter and the shunt.
View SolutionAn electrical appliance having a resistance of 200 Ω is operated at 200 V. Calculate the energy consumed by the appliance in 5 minutes in joule
View SolutionAn electrical appliance having a resistance of 200 Ω is operated at 200 V. Calculate the energy consumed by the appliance in 5 minutes
in kWh.
View SolutionCalculate the potential difference across the ends of a wire of resistance 2Ω'whenacurrentof1.5 A passes through it.
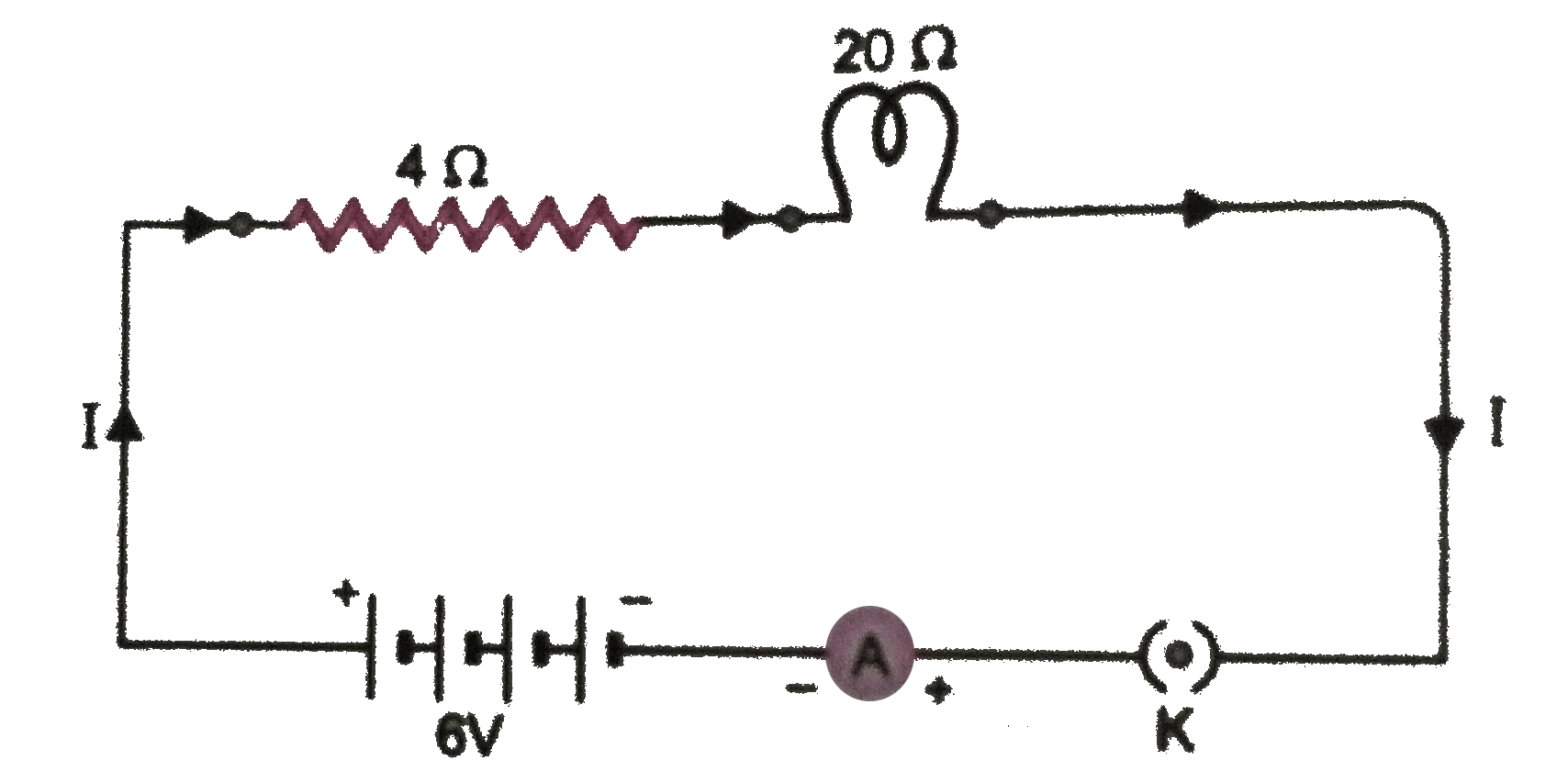
View SolutionAn electric lamp, whose resistance is 20Ω and a conductor of 4Ω resistance are connected to a 6V battery as shown in (Fig. 3.18) Calculate.
(a) the total resistance of the circuit,
(b) the current through the circuit, and
( c) the potential difference across the electric lamp and the conductor.
 .
.
View SolutionAn electric lamp of resistance 2Ω and a conductor of resistance 4Ωare connected to a
6V Battery as shown in the circuit. Calculate:
(a) the totak resistance of the circuit,
(b) the current through the circuit,
(c) the potential difference across the (i) electric lamp
and (ii) conductor, and
(d) power of the lamp.
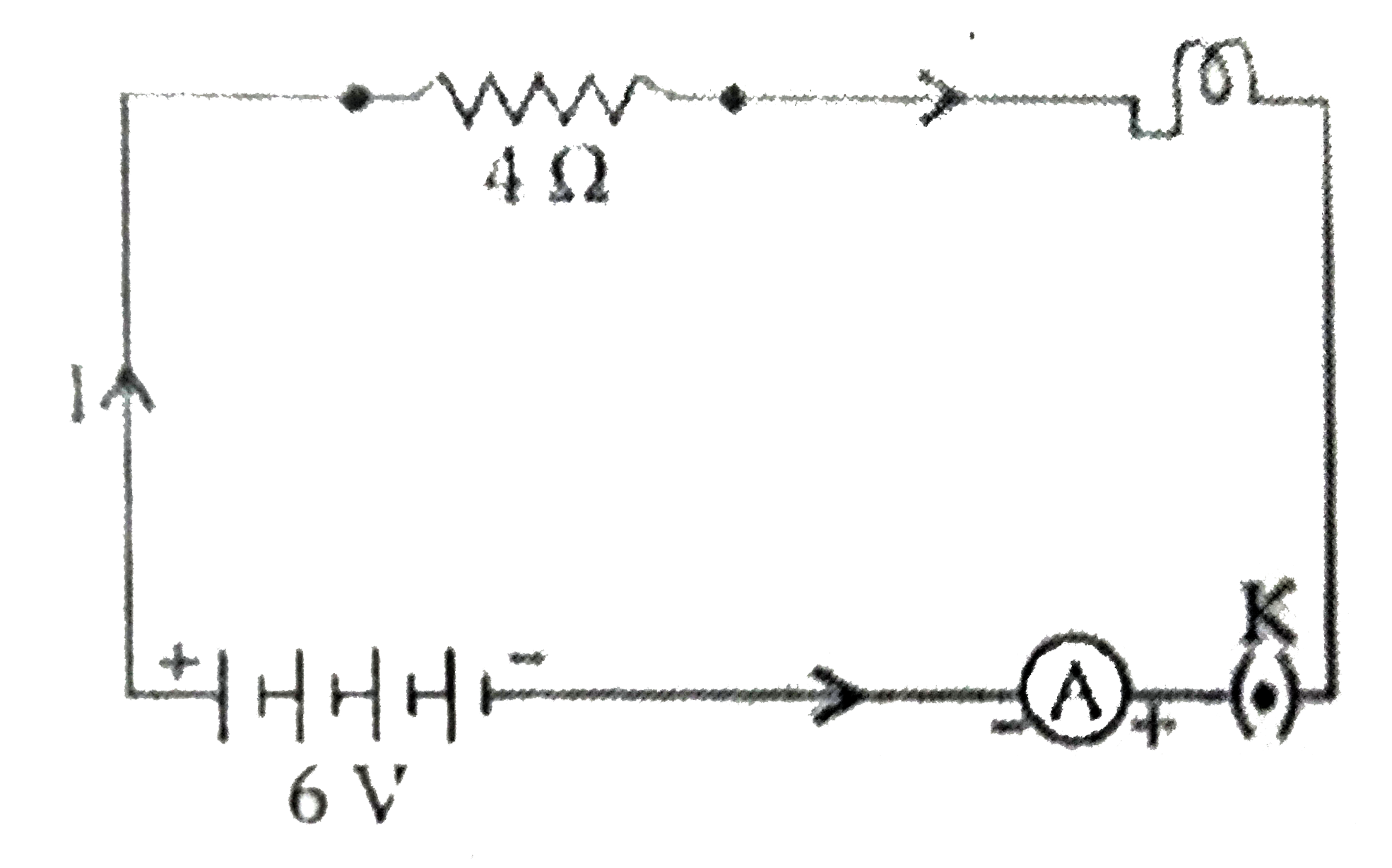
View SolutionCalculate the potential difference required across a conductor of resistance 5 Ω to pass a current of 1.5 A through it.
View SolutionA battery of emf 2.0 V and internal resistance 0.50(Ω)supplies a current of 100 mA. Find (a) the potential defference across the terminals of the battary and (b) the thermal energy develop in the battery in 10 s.
View Solution
