Theorem 6.5 : If a transversal intersects two lines such that a pair of interior angles on the same side of the transversal is supplementary, then the two lines are parallel.
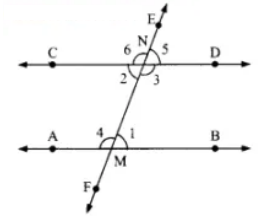
Since ray ND stands on line EF,
∠3+∠5=180 (linear pair of angles)
But, ∠1=∠5 (Corresponding angles as AB∣∣CD)
From above equations, we get
∠1+∠3=180
Again ray CN stands on EF,
∠2+∠6=180 (Linear pair of angles)
But ∠4=∠6 (Corresponding angles as AB∣∣CD)
∠2+∠4=180
Hence, we can say if a transversal intersects two parallel lines, then each pair of interior angles are supplementary
Topper's Solved these Questions
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 6.6
1 Videos
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 6.7
1 Videos
- NCERT THEOREMSBOOK - NCERT ENGLISHCHAPTER - NCERT THEOREMSEXERCISE - THEOREM 6.4
1 Videos
- LINES AND ANGLESBOOK - NCERT ENGLISHCHAPTER - LINES AND ANGLESEXERCISE - Exercise 6.1
6 Videos
- NUMBER SYSTEMSBOOK - NCERT ENGLISHCHAPTER - NUMBER SYSTEMSEXERCISE - EXERCISE 1.4
2 Videos
Similar Questions
Theorem 6.4 : If a transversal intersects two parallel lines, then each pair of interior angles on the same side of the transversal is supplementary.
View SolutionTheorem 6.3 : If a transversal intersects two lines such that a pair of alternate interior angles is equal, then the two lines are parallel.
View SolutionTheorem 6.2 : If a transversal intersects two parallel lines, then each pair of alternate interior angles is equal.
View SolutionIf a transversal intersects two lines such that the bisectors of a pair of corresponding angles are parallel, then prove that the two lines are parallel.
View SolutionIf two parallel lines are intersected by a transversal, prove that the bisectors of the interior angles on the same side of transversal intersect each other at right angles.
View SolutionIf the bisectors of a pair of alternate angles formed by a transversal with two given lines are parallel, prove that the given lines are parallel.
View SolutionIn the adjoining figure, identify(i) the pairs of corresponding angles.(ii) the pairs of alternate interior angles.(iii) the pairs of interior angles on the sameside of the transversal.(iv) the vertically opposite angles.
View SolutionTwo straight lines are cut by a transversal. If the bisectors of a pair of co-interior angles are perpendicular to each other , prove the two straight lines are parallel to each other.
View SolutionRead the following statements which are taken as axioms
(i) If a transversal intersects two parallel lines, then corresponding angles are not necessarily equal.
(ii) If a transversal intersect two parallel lines, then alternate interior angles are equal.
Is this system of axioms consistent ? Justify your answer.
View SolutionTheorem 6.5 : If one angle of a triangle is equal to one angle of the other triangle and the sides including these angles are proportional, then the two triangles are similar.
View Solution
