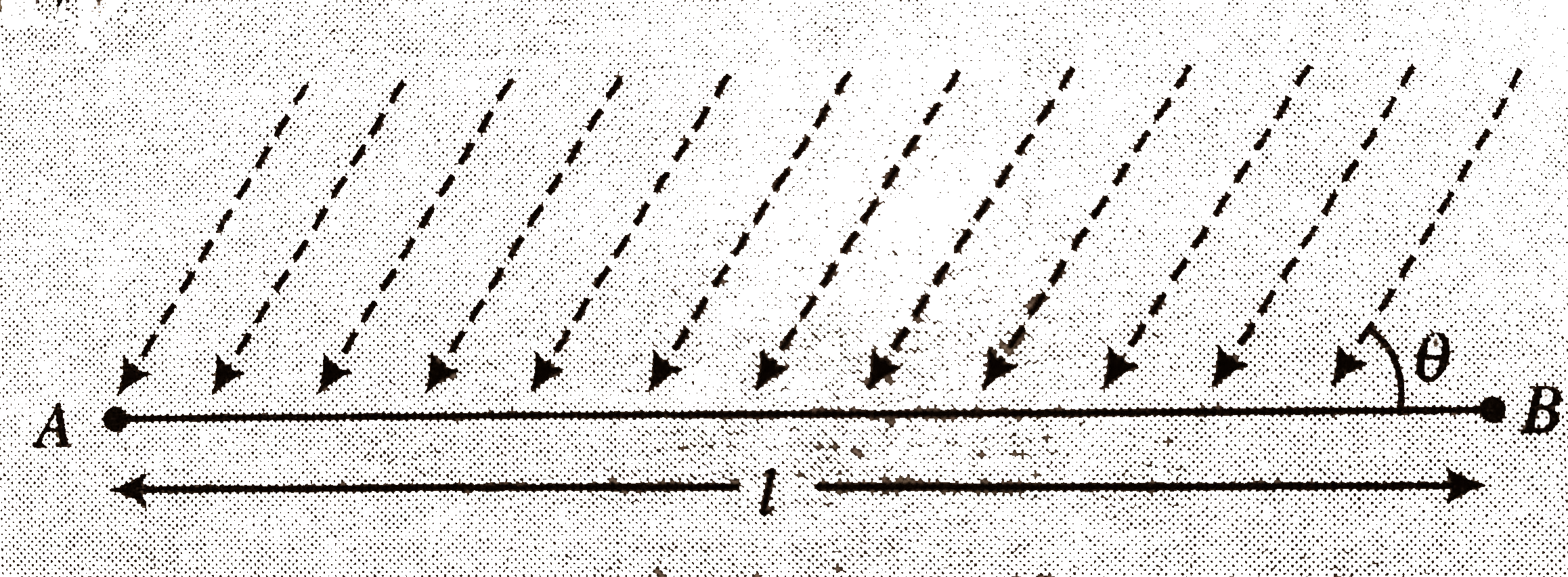
An aeroplane flies along a straight path A to B and back again the distances between a and B is 1 and the aeroplane maintains the constant speed v . There is steady wind with a speed u at an angle theta with line AB . determine the expression for the total time of the trip .

Text Solution

Verified by Experts

Velocity of plane along AB = V cos $α - u cos θ$
and for no - drift from line
$A B : v sin α = u sin θ \Rightarrow sin α = \frac{u sin θ}{v}$
time taken from A to B $t_{A B} = \frac{t}{v cos α - u cos θ}$
B to A
velocity of plane along BA $= v cos α + u cos θ$
and for no drift from line AB $: v sin α = u sin θ \Rightarrow sin α = \frac{u sin θ}{v}$
time taken from B to A : $v sin α = u sin θ \Rightarrow sin α = \frac{u sin θ}{v}$
time taken from B to $A : t_{B A} = \frac{t}{v cos α + u cos θ}$
total time teken $= T_{A B} + t_{B A} = \frac{2 v l cos α}{v^{2} {cos}^{2} α - u^{2} {cos}^{2} θ} = \frac{2 v t \frac{\sqrt{(u^{2} {sin}^{2} θ)}}{v^{2}}}{v^{2} - u^{2}}$

Updated on:21/07/2023

An aeroplane flies along straight line from A to B and backs again to the same point. There is a steady wind speed v. The distance between A and B is l still air speed of the aeroplane is V, then:
ATotal time for the round trip, if the wind blows along the line AB, is $\frac{2 V l}{v^{2} - v^{2}}$
BTotal time for the round trip, if the wind blows perpendicular to the line AB, is $\frac{2 l}{\sqrt{V^{2} - v^{2}}}$
CTotal time for the round trip depends on the direction of wind.
DTotal time for the round trip is independent of direction of wind.
The aeroplane are so designed that the speed of air.
AOn top side is more than on lower side
BOn top side is less than on lower side
CIs same on both sides
DIs turbulent
An eaeroplane is to go along straight line from A to B, and back again. The relative speed with respect to wind is V. The wind blows perpendicular to line AB with speed v. The distance between A and B is l. The total time for the round trip is:
A $\frac{2 l}{\sqrt{V^{2} - v^{2}}}$
B $\frac{2 v l}{V^{2} - v^{2}}$
C $\frac{2 V l}{V^{2} - v^{2}}$
D $\frac{2 l}{\sqrt{V^{2} + v^{2}}}$