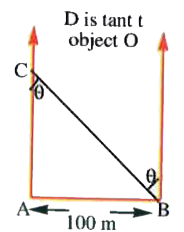
A man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object in line with AC. He then walks perpendicular to AC up to B, a distance of 100 m, and looks at O and C again. Since O is very distant, the direction BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle = 40∘ (θ is known as 'parallax') estimate the distance of the tower C from his original position A.
 Solution in Telugu
Solution in Telugu
Parallax angle θ=40∘,AB=ACtanθ
AC = AB/ tanθ=100m/tan40∘
=100 m /0.8391 = 119 m.
Topper's Solved these Questions
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE -1 A
187 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - EXERCISE- 1 B
29 Videos
- UNITS AND MEASUREMENTSBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTSEXERCISE - QUESTIONS FOR DESCRIPTIVE ANSWERS
7 Videos
- UNITS AND MEASUREMENTBOOK - AAKASH SERIESCHAPTER - UNITS AND MEASUREMENTEXERCISE - PRACTICE EXERCISE
45 Videos
- WAVE MOTIONBOOK - AAKASH SERIESCHAPTER - WAVE MOTIONEXERCISE - PRACTICE SHEET ADVANCED (INTEGER TYPE QUESTIONS)
10 Videos
Similar Questions
Atomic clock generally used in the national standards, is based on the periodic vibrations produced in a
View Solution(A): The magnitude of a physical quantity does not change when the system of units is changed from S.I system to C.G.S system.
(R): The magnitude of a physical quantity is independent of system of units
View SolutionIn the formula X=3YZ2,XandZ have dimensions of capacitance and magnetic induction respectively . Find the dimensions of Y in MKSQ system.
View SolutionThe ratio of S.I. unit of universal gravitational constant to C.G.S. unit is
View SolutionA source emitting sound of frequency 180 Hz is placed in front of a wall at a distance of 2m from it. a detector is also placed in front of the wall at the same distance from it. The minimum distance between the source and the detector is x mtrs for which the detector shows a maxima of sound. Speed of sound in air = 360 m/s
View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicualr to AC upto B, a distaance of 100m and looks at O and C again. Since O is very distant, the direction of BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle theta = 40^@ ( theta "is known as parallax"). Estimate the distance fo the tower C from his original position A.
View Solutionकिसी टावर के पाद से 160 मी . दूर स्थित बिंदु से टावर का उन्नयन कोंढ टावर के पाद की ओर 100 मी . दूरी चलने पर दो गुना हो जाता है । टावर की ऊंचाई ज्ञात करे ।
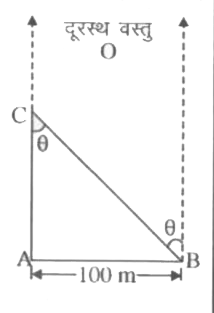
View Solutionएक व्यक्ति अपने पास की किसी मीनार की अपने से दूरी का आकलन करना चाहता है । वह मीनार C के सामने किसी बिंदु A पर खड़ा होता है और AC की सीध में बहुत दूर स्थित किसी बिंदु O को देखता है । फिर वह, AC के लंबवत 100 m दूर स्थित बिंदु B तक चलता है और वहाँ से O एवं C को फिर देखता है । क्योंकि O बहुत अधिक दूरी पर है , BO एवं AO की दिशाएँ व्यावहारिक रूप में एक ही हैं, लेकिन वह पाता है कि C की दृष्टि रेखा मूल दृष्टि रेखा के सापेक्ष θ=40∘ पर घूम गई है (θ को लम्बन कहा जाता है ) उसकी मूल स्थिति A से मीनार C की दूरी का आकलन कीजिए ।
View Solutionएक व्यक्ति अपने पास की किसी मीनार की अपने से दूरी आकलन करना चाहता है। वह मीनार C के सामने किसी बिंदु A पर खड़ा होता है और AC की सीध में बहुत दूर स्थित किसी बिंदु O को देखता है। फिर वह AC के लंबवत 100 m दूर स्थित बिंदु B तक चलता है। और वहाँ से O एवं C को फिर देखता है। क्योंकि O बहुत अधिक दूरी पर है, BO एवं AO की दिशाएँ व्यावहारिक रूप में एक ही हैं, लेकिन वह पता है कि C की दृष्टि रेखा मूल दृष्टि रेखा के सापेक्ष θ=40∘ पर घूम गई है। (θ को लंबवत कहा जाता है ) उसकी मूल स्थिति A से मीनार C की दूरी का आकलन कीजिए।
View SolutionA man standing at a point P is watching the top of elevation of 30∘. The man walks some distance towards the tower and then his angle of elevation of thetop of the toweris 60∘. If the height of the tower 30 m, then the distance he moves is
View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicular to AC up to B, a distance of 100 m, and looks at O and C again. Since O is very distant , the direction BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle θ=40∘ (θ is known as 'Parallax') estimate the distance of the tower C from his original position A.
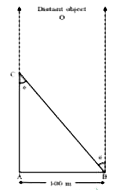
View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicular to AC up to B, a distance of 100 m, and looks at O and C again. Since O is very distant , the direction BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle theta = 40^(@) ( theta is known as 'Parallax') estimate the distance of the tower C from his original position A.
View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object in line with AC. He then walks perpendicular to AC up to B, a distance of 100 m, and looks at O and C again. Since O is very distant, the direction BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle = 40∘ (θ is known as 'parallax') estimate the distance of the tower C from his original position A.

View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object in line with AC. He then walks perpendicular to AC up to B, a distance of 100 m, and looks at O and C again. Since O is very distant, the direction BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle = 40∘ (θ is known as 'parallax') estimate the distance of the tower C from his original position A.

View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in front of the tower C and spots a very distant object O in line with AC. He then walks perpendicualr to AC upto B, a distaance of 100m and looks at O and C again. Since O is very distant, the direction of BO is practically the same as AO, but he finds the line of sight of C shifted from the original line of sight by an angle θ=40∘(θ is known as parallax). Estimate the distance fo the tower C from his original position A.
View SolutionA man wishes to estimate the distance of a nearby tower from him. He stands at a point A in fron of the tower C and spots a very distant objct O in line with AC. He then walks pependicular to AC up to B, a distance of 100 m and look at O and C again. Since O is very distant the direction BO is practivally the same as AO, but he finds the line of slight by of C shifted fro the original line of slight by an angle θ=30∘(θ is known as parallax) estimate the distance of the tower C from his original position A.
View Solution
