Question

The huge advantage of using the conservation of energy instead of Newton's laws of motion is that we can jump from the initial state to the final state without considering all the intermediate motion. Here is an example. In Fig. 8-25, a child of mass m is released from rest at the top of a water slide, Assuming that the slide is frictionless because of the water on it, find the child's speed at the bottom of the slide.

Explanation

(1) We cannot find her speed at the bottom by using her acceleration along the slide as we might have in earlier chapters because we do not know the slope (angle) of the slide. However, because that speed is related to her kinetic energy, perhaps we can use the principle of conservation of mechanical energy to get the speed . Then we would not need to know the slope. (2) Mechanical energy is conserved in a system if the system is isolted and if only conservative forces cause energy transfers within it. Let.s check.
Forces: Two forces act on the child. The gravitational force, a conservative force, does work on her. The normal force on her from the slide does no work because its direction at any point during the descent is always perpendicular to the direction in which the child moves.

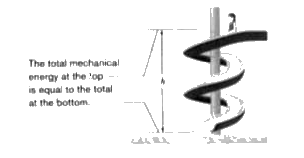
Figure 8-25 A child slides down a water slide as she descends a height h.
System: Because the only force doing work on the child is the gravitational force, we choose the child-Earth system as our system, which we can take to be isolaed.
Thus, we have only a conservative force doing work in an isolated system, so we can use the principle of conservation of mechanical energy.
Calculations: Let the mechanical energy be `E_("mec.t)` when the child is at the top of the slide and `E_("mec.b")` when she is at the bottom. Then the conservation principle tells us
`E_("mec.b")=E_("mec.t")`
To show both kinds of mechanical energy, we have `K_(b) +U_(b) =K_(t)+U_(t)`,
or `1/2 mv_(b)^(2) + mgy_(b)=1/2 mv_(t)^(2)+mgy`.
Dividing by m and rearranging yield
`v_(b)^(2)=v_(t)^(2)+2g(y_(t)-y_(b))`.
Putting `v_(t)=0` nd `y_(t)-y_(b)=h` leads to
`v_(b)=sqrt(2gh)=sqrt((2)(9.8 m//s^(2))(8.5 m))`
`=13m//s`.
This is the same speed that the child would reach if she fell 8.5 m vertically. On an actual slide, some frictional forces would act and the child would not be moving quite so fast.
Comments: Although this problem is hard to solve directly with Newton.s laws, using conservation of mechanical energy makes the solution much easier. However, if we were asked to find the time taken for the child to reach the bottom of the slide, energy methods would be of no use, we would need to know the shape of the slide, and we would have a difficult problem.