Find the ratio of the area of the bigger circle and smaller circle.
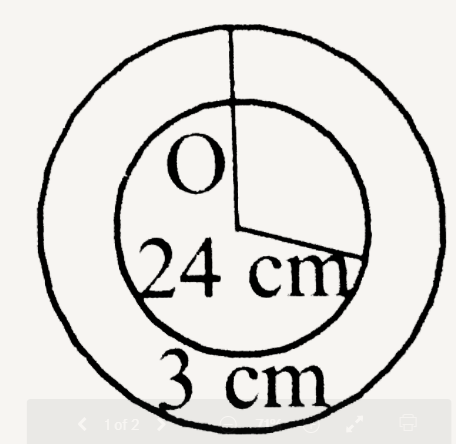
I. The radius of the smaller circle is 24 cm.
II. The difference between the radii of bigger and the smaller circles is 3 cm.
if the data in statement I alone are sufficient to answer the questin, while the data in statement II alone are not sufficient to answer the question.
if the data in statement II alon are sufficient to answer the question, while the data in statement I alone are not sufficient to answer the question.
if the data either in statement I alone or in statement II alone in sufficient to answer the question.
if the data in both the statements I and II together are necessary to answer the question.
From both the statements,
r1=24cm
r2−r1=3 cm
⇒r2−r1+3=24+3=27 cm
∴Required ratio=πr22πr21=r22r21=272242=8164
So data in both the statements together are necessary to answer the question
Topper's Solved these Questions
Similar Questions
In two concentric circles, a chord of length 8cm of the larger circle touches the smaller circle. If the radius of the larger circle is 5cm then find the radius of the smaller circle.
View SolutionIn the figure, the radius of the larger circle is 2 cm and the radius of the smaller circle is 1 cm and the larger circle passes through the centre of the smaller circleThe length (in cm) of the chord AB is :
View Solution
Knowledge Check
The area of a circle is proportional to the square of its radius. A small circle of radius 3 cm is drawn within a larger circle of radius 5 cm. Find the ratio of the area of the annular zone to the area of the larger circle. (Area of the annular zone is the difference between the area of the larger circle and that of the smaller circle).
A9:16B9:25C16:25D16:27The difference between the radii of the bigger circle and smaller circle is 14 cm and the difference between their areas is 1056cm2. Radius of the smaller circle is
A7 cmB5 cmC9 cmD3 cmTwo circles touch each other externally. The distance between their centres is 8 cm. If the radius of one circle is 3 cm, then the radius of the other circle is:
दो वृत्त एक दूसरे को बाह्य रूप से स्पर्श करते हैं। उनके केंद्रों के बीच की दूरी 8 सेमी है। यदि एक वृत्त की त्रिज्या 3 सेमी है, तो दूसरे वृत्त की त्रिज्या है:A7.5 cmB9 cmC8 cmD10 cm
The adjoining figure shows two circles with the same centre. The radius of the larger circle is 10 cm and the radius of the smaller circle is 4 cm.
View SolutionThe difference between the radii of the smaller circle and the bigger circle is 7 cm and the difference between the areas of the two circles is 1078 sq cm. What is the radius of the smaller circle in cm?
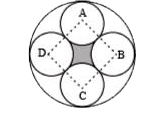
View SolutionIn the figure below, the radius of the bigger circle is (√2+1) cm and the radius of all the smaller circles are equal. Each of the smaller circles touches two of the other three smaller circles and the larger circle as shown. Find the area (in cm2) of the shaded portion.
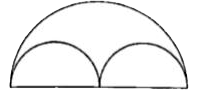
View SolutionIn the given figure there are 3 semicircles , the radii of each smaller circle is equal. If the radius of the larger circle be 22 cm , then the area of the shaded region is :
View SolutionThe circumference of two circles is 44 metres and 440 metres respectively. What is the difference between the area of the larger circle and the smaller circle ?
View Solution
