A square loop of side 12 cm with its sides parallel to X and Y axes is moved with a velocity of 8cm s−1 in the positive x-direction in an environment containing a magnetic field in the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient of 10−3T cm−1 along the negative x-direction (that is it increases by 10−3T cm−1 as one moves in the negative x-direction), and it is decreasing in time at the rate of 10−3Ts−1. Determine the direction and magnitude of the induced current in the loop if its resistance is 4.50mΩ .Solution in Gujrati
Here magnetic field changes with position and time both. Hence we have two values of induced emf say ε1 and ε2 respectively. Situation given in the statement is shown in the figure given below.
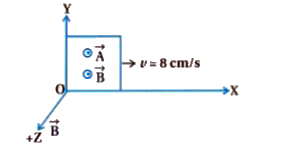
ε1=−dϕdt=−ddt(ABcos0∘)(∵→A∣∣→B)
∴ε1=−AdBdt
=−A(dBdx)(dxdt)
=−l2dBdxv
=−(0.12)2(−10−310−2)(8×10−2) ( ∵dBdx along +X axis is −10−3Tcm)
∴ε1=1.152×10−4 V....(1)
ε2=−dϕdt=−ddt(AB)
∴ε2=−AdBdt
=−l2dB/(dt)
=−(0.12)2(−10−3)
∴ε2=0.144×10−4 V ...(2)
Induced current I=effective induced emf(ε)equivalent resistance (R)
∴I=ε1+ε2R ( ∵ Here ε1>0,ε2>0⇒ε=ε1+ε2)
∴I=(1.152+0.144)×10−44.5×10−3
∴I=2.88×10−2 A
Here, as the loop moves along + X axis, outward magnetic flux linked with it goes on decreasing and so according to Lenz.s law, induced current should flow anticlockwise in the loop so that outward magnetic flux can increase.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Numerical From DARPAN Based on Textbook
10 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section C Ncert Exemplar Solution (MCQs)
16 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Section B (Numerical) Textual Illustrations
26 Videos
- ELECTRIC CHARGES AND FIELDS BOOK - KUMAR PRAKASHANCHAPTER - ELECTRIC CHARGES AND FIELDS EXERCISE - SECTION D MCQS ASKED IN COMPETITIVE EXAMES (MCQS AKSED IN BOARD EXAM AND GUJCET)
14 Videos
- ELECTROMAGNETIC WAVESBOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - BOARD.S QUESTION PAPER MARCH - 2020 (PART - B) SECTION - C
5 Videos
Similar Questions
A conducting circular loop is placed in a uniform magnetic field of 0.04 T with its plane perpendicular to the field. Some how, the radius of the loop starts shrinking at a constant rate of 2 mm/s. Find the induced emf in the loop at an instant when the radius becomes 2 cm solution.
View SolutionA square of side L meters lies in the xy-plane in a region, where the magnetic field is given by →B=B0(2ˆi+3ˆj+4ˆk)T where B0 is constant. The magnitude of flux passing through the square is
View Solution
Consider Experiment:
(a) What would you do to obtain a large deflection of the galvanometer ?
(b) How would you demonstrate the presence of an induced current in the absence of a galvanometer ?
View SolutionWhen air is replaced by dielectric medium of constant K, the maximum force of attraction between two charges separated by distance d, ..........
View SolutionDetermine the current in each branch of the given network.

View SolutionA square loop of side 12cm with its sides parallel to x and y- axes is moved with a velocity 8cm/s along positive x-direction in an environment containing magnetic field along +ve z-direction. The field has a gradient of 10−3tesla/em along −ve x-direction (increasing along −ve x-axis) and also decreases with time at the rate of 10−3tesla/s. The emf induced in the loop is
View SolutionA square loop of side 12 cm with its sides parallel to X and Y axes is moved with a velocity of 8 cm s^(-1) in the positive x-direction in an environment containing a magnetic field in the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient of 10^(-3) T cm^(-1) along the negative x-direction (that is it increases by 10^(-3) T cm^(-1 ) as one moves in the negative x-direction), and it is decreasing in time at the rate of 10^(-3) T s^(-1) . Determine the direction and magnitude of the induced current in the loop if its resistance is 4.50 mOmega .
View SolutionA squre loop of side a= 12 cm with its sides parallel to x, and y-axis is moved with velocity, V=8 cm/s in the positive x direction in a magnetic field along the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient delB//delx=-10^(-3) T//cm along the x-direction, and it is changing in time at the rate delB//delt=7T//sec in the loop if its resistance is R =4.5 Omega . Find the current .
View SolutionA square loop of side 12 cm with its sides parallel to X and velocity of 8 cm s in the positive x-direction in an environment containing a magnetic field in the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient of 10 T cm along the negative x-direction (that is it increases by 103 T cm- as one moves in the negative x-direction), and it is decreasing in time at the rate of 103 Ts. Determine the direction and magnitude of the induced current in axes is moved with a the loop if its resistance is 4.50 m2. Sol. Given, side of loop a = 12 cm . Area of loop (A) = a = (12) = 144 cm = 144 x 10 m? (. Area of square = (side))
View SolutionA square loop of side 12 cm with its sides parallel to X and Y axes is moved with a velocity of 8 cm. s^(-1) in the positive x-direction in an environment containing a magnetic field in the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient of 10^(-3)T.cm^(-1) along the negative x-direction and is decreasing in time at the rate of 10^(-3)T.s^(-1) . Determine the direction and magnitude of induced current in the loop if its resistance is 4.50 m Omega .
View Solution12 cm भुजा वाला वर्गाकार लूप जिसकी भुजाएं X एवं Y अक्षो के समांतर है , x-दिशा में 8cms∧(−1) की गति से चलाया जा रहा है । लूप तथा उसकी गति का परिवेश धनात्मक z-दिशा के चुम्बकीय क्षेत्र का है। चुंबकीय क्षेत्र न तो एकसमान है और न ही समय के साथ नियत है । इस क्षेत्र की ऋणात्मक दिशा में प्रवणता 10−3Tcm−1 है (अर्थात ऋणात्मक x-अक्ष की दिशा में इकाई सेंटीमीटर दूरी पर क्षेत्र के मान में 10−3Tcm−1 की वृद्धि होती है), तथा क्षेत्र के मान में 10−3Ts−1 की दर से कमी भी हो रही है । यदि कुंडली का प्रतिरोध 4.50mΩ हो तो प्रेरित धारा का परिमाण एवं दिशा ज्ञात कीजिये।
View SolutionA squre loop of side a= 12 cm with its sides parallel to x, and y-axis is moved with velocity, V=8 cm/s in the positive x direction in a magnetic field along the positive z-direction. The field is neither uniform in space nor constant in time. It has a gradient ∂B/∂x=−10−3T/cm along the x-direction, and it is changing in time at the rate ∂B/∂t=7T/sec in the loop if its resistance is R=4.5Ω. Find the current .
View SolutionA square loop of side 12cm with its sides parallel to x and y- axes is moved with a velocity 8cm/s along positive x-direction in an environment containing magnetic field along +ve z-direction. The field has a gradient of 10−3tesla/em along −ve x-direction (increasing along −ve x-axis) and also decreases with time at the rate of 10−3tesla/s. The emf induced in the loop is
View SolutionA rectangular loop of wire is placed in the XY-plane with its side of length 3 cm parallel to the X-axis and the side of length 4 cm parallel to the Y-axis. It is moving in the positive X-direction with the speed 10 cm/s. A magnetic field exists in the space with its direction parallel to the Z-axis. The field decreases by 2×10−3 T/cm along the positive X-axis and increases in time by 2×10−2 T/s. The induced emf in the wire is
View SolutionA square loop of side 12 cm with its sides parallel to X and Y axes is moved with a velocity of 8cms^(-1) in the possitive x-direction in an environment containing a magnetic field in the positive z- direction. The field is neither uniform in space nor constant in time. It has a gradient of 10^(-3)Tcm^(-1) along the negative x-direction (that is it increases by 10^(-3)T as one moves in the negative x-direction), and it is decreasing in time at the rate of 10^(-3)Ts^(-1) . Determine the direction and magnitude of the induced current in the loop of its resistance is 4.50mOmega .
View Solution
