When an object is placed normally on the principal axis of a spherial mirror at a distance 'a' from its pole, its image is formed at a distance 'v' from the pole of mirror such that 1v+1u=1f, where f = focal length of given mirror.
The relation is called mirror formula and is true for all types of mirrors under all conditions. However, values of u, v and f must be put with proper signs are per the sign convention followed. If 'h' be the height of the linear object and h' the height of image, then ratio h'h is called linear magnification or lateral magnification and its value m is given as :
m=h'h=−vu
(e) Find an expression for longitudinal magnification of a small object placed linearly along the axis of a spherical mirror.
Let linear size of object be du andlinear size of its image be dv.
As per mirror formula, we have
1v+1u=1f
On differentiation, we get
−dvv2−duu2=0
⇒ dvv2=−duu2
∴ Longitudinal magnification =dvdy=−v2u2
Topper's Solved these Questions
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - U-LIKE SERIESCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - MULTIPLE CHOICE QUESTIONS
39 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - U-LIKE SERIESCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - FILL IN THE BLANKS
20 Videos
- RAY OPTICS AND OPTICAL INSTRUMENTSBOOK - U-LIKE SERIESCHAPTER - RAY OPTICS AND OPTICAL INSTRUMENTSEXERCISE - SELF ASSESSMENT TEST (SECTION B)
3 Videos
- NUCLEIBOOK - U-LIKE SERIESCHAPTER - NUCLEIEXERCISE - Self Assessment Test
10 Videos
- SEMICONDUCTOR ELECTRONICS : MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - U-LIKE SERIESCHAPTER - SEMICONDUCTOR ELECTRONICS : MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - SECTION B
1 Videos
Similar Questions
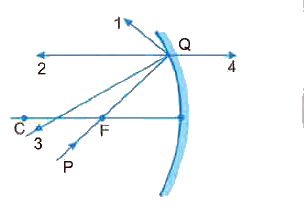
The direction of ray of light incident on a concave mirror is shown by PQ in Fig. The direction in which the ray would travel after reflection is shown by four rays marked 1, 2, 3 and 4. Which of the four rays correctly shows the direction of reflected ray?
View SolutionAn object viewed directly through a rectangular slan 12 cm thick appears to be raised by 4 cm. The refractive index of the material of slab is ________.
View SolutionA beam of light converges at aoint P. Now a convex lens is placed in the path of the convergent beam at 15 cm from P. At what point does a beam converge if the convex lens has a focal length 10 cm?
View SolutionThe binding energy of 21H is 1.112 MeV per nucleon and an alpha-particle 42He has a binding energy of 7.047 MeV per nucleon. In the fusion reaction 21H+ 21H→ 42He+Q, the energy released is
View SolutionThree photo diodes D1,D2andD3 are made of semiconductors having band gaps of 2.5 eV, 2eV and 3eV respectively. Which of them will not be able to detect light of wavelength 600 nm?
View SolutionThe focal f to a mirror is given by 1f=1u+1v where u and v represent object and image distance respectively then
View SolutionThe mirror formula is (1)/(f) = (1)/(v) + (1)/(u) where symbols have standard meaning. How will focal length of mirror change when u is changed by moving the object towards or away from the mirror?
View SolutionThe formula governing reflection of light from a spherical mirror is
1v+1u=2R, where
u = distance of object from pole of mirror,
υ = distance of image from pole of mirror
f = focal length of mirror,
R = radius of curvature of mirror.
This is known as mirror formula and is applicable equally to concave mirror and convex mirror.
m=IO=υu
Read the above passage and answer the following questions :
(i) An object is held at a distance of 30cm in front of a concave mirror of radius of curvature 40cm. Calculate distance of the image from the object ? What is linear magnification of the mirror ?
(ii) The object is moved to a distance of 40cm in front of the mirror. How is focal length of mirror affected ?
(iii) What values of life do you learn from the mirror formula ?
View SolutionAn object is placed at a distance u from a concave mirror and its real image is received on a screen placed at a distance of v from the mirror. If f is the focal length of the mirror, then the graph between 1/v versus 1/u is
View SolutionIf u represents object distance from pole of spherical mirror and V represents image distance from pole of mirror and f is the focal length of the mirror, then a straight line u=v will cut u versus v graph at
View SolutionA real object is placed at a distance f from the pole of a convex mirror, infront of the convex mirror. If focal length of the mirror is f, then distance of the image from the pole of the mirror is
View SolutionAn object is placed at a distance f in the front of a convex mirror. If focal length of the mirror is f, then distance of image from pole of the mirror is
View SolutionStatement I : Focal length of a spherical mirror depends on the distances of object and image from the mirror.
Statement II:1f=1v+1u
View SolutionWhen an object is placed normally on the principal axis of a spherial mirror at a distance 'a' from its pole, its image is formed at a distance 'v' from the pole of mirror such that 1v+1u=1f, where f = focal length of given mirror.
The relation is called mirror formula and is true for all types of mirrors under all conditions. However, values of u, v and f must be put with proper signs are per the sign convention followed. If 'h' be the height of the linear object and h' the height of image, then ratio h'h is called linear magnification or lateral magnification and its value m is given as :
m=h'h=−vu
(a) A planer object of length 3 cm and breadth 2 cm is held normal to the principal axis of a concave mirror of focal length 20 cm at a distance of 30 cm from its pole. Where is its image formed?
View SolutionWhen an object is placed normally on the principal axis of a spherial mirror at a distance 'a' from its pole, its image is formed at a distance 'v' from the pole of mirror such that 1v+1u=1f, where f = focal length of given mirror.
The relation is called mirror formula and is true for all types of mirrors under all conditions. However, values of u, v and f must be put with proper signs are per the sign convention followed. If 'h' be the height of the linear object and h' the height of image, then ratio h'h is called linear magnification or lateral magnification and its value m is given as :
m=h'h=−vu
What is the surface area of image of linear object formed by the given concave mirror?
View Solution
