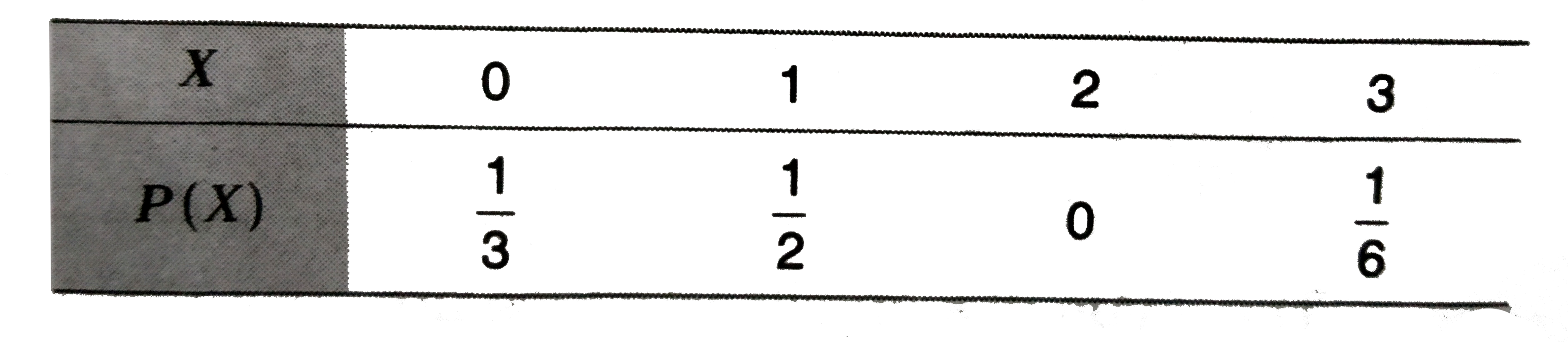
If mandσ2 are the mean and variance of the random variable X, whose distribution is given by:
X=x:0123P(X=x):1312016 Solution in Telugu
m=σ2=2
m=1,σ2=2
m=σ2=1
m=2,σ2=1
The correct Answer is:C
Step by step video, text & image solution for If m and sigma^(2) are the mean and variance of the random variable X, whose distribution is given by: {:(X=x:,0,1,2,3),(P(X=x):,(1)/(3),(1)/(2),0,(1)/(6)):} by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Topper's Solved these Questions
- BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLE EXERCISE - EXERCISE - II76videos
RANDOM VARIABLE
- BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLE EXERCISE - PRACTICE EXERCISE34videos
RANDOM VARIABLE
- BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLE EXERCISE - ADDITIONAL EXERCISE25videos
RANDOM VARIABLE
- BOOK - AAKASH SERIESCHAPTER - QUADRATIC EQUATIONS & EXPRESSIONSEXERCISE - PRACTICE EXERCISE88videos
QUADRATIC EQUATIONS & EXPRESSIONS
- BOOK - AAKASH SERIESCHAPTER - RANDOM VARIABLESEXERCISE - Exercise 4.49videos
RANDOM VARIABLES
Similar Questions
A person who tosses an unbiased coin gains two points for turning up a head and loses one point for a tail. If three coins are tossed and the total score X is observed, then the range of X is
02:31View SolutionA random variable X ahs the following distribution
x1234P(X=x)c2c3c4c
The value of c is02:50View SolutionThe probability function of a random variable X is given by
p(x)=13, if x = -1, 0, 1
=0, otherwise.
Find the distribution function of X.03:25View SolutionIf 3x+22x+3=4x+33x+4, then x =
02:31View SolutionA random variable X takes four values with probabilities 1+3x4,(1−x),1+2x4 and 1−4x4. Find the set of values of x for the variable X to have probability distribution.
03:31View SolutionThe mean and variance of a random variable X having a binomial distribution are 4and2 respectively. The P(X=1) is
01:26View SolutionIf m and σ2 are the mean and variance of the random variable X whose distribution is given by
 01:50View Solution
01:50View SolutionThe probability distribution of a random variable X is given below:
X=x0123P(X=x)110210310410
Then the variance of X isView SolutionIf mandσ2 are the mean and variance of the random variable X, whose distribution is given by:
X=x:0123P(X=x):131201603:30View SolutionThe mean and variance of a random variable X having a binomial distribution are 4 and 2 respectively, then P(X=1) is
Theoretical Distributions03:15View SolutionIf a random variable X has a Poisson distribution such that P(X=1)=P(X=2) then
I: its mean is 2 II: its variance is 104:03View SolutionIf a random variable X has a poisson distribution such that P(X=1)=P(X=2), its mean and variance are
View SolutionLet X be a random variable such that P(X=−2)=P(X−1)=P(X=2)=P(X=1)=16 and P(X=0)=13. Find the mean and variance of X.
07:09View SolutionThe variance of the random variable X having the following distribution is X=x:−2−1012P(X=x):1616131616
06:17View SolutionThe mean and variance of a random variable X having a binomial distribution are 4and2 respectively. The P(X=1) is
01:42View SolutionIn binomial distribution of random variable X if mean = np and variances = npq then
P(X=k)P(X=k−1)=……View Solution

