A binary star system consists of two stars. One star has twice the mass of the other. The star rotates about their common centre of mass. Which of the following statement is correct?
Star having the smaller mass has twice angular momentum compared to heavier star.
Both stars have same angular momentum about centre of mass.
Both stars have same linear speed.
Both the stars have same kinetic energy.
The correct Answer is:A
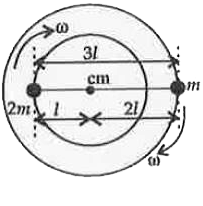
Let the distance between the two stars be 3l. Then, Angular momentum of mass m about common centre of mass
Angular momentum of mass 2m about common centre of mass =
There ratio is
Topper's Solved these Questions
- GRAVITATIONBOOK - MTG GUIDECHAPTER - GRAVITATIONEXERCISE - NEET CAFE TOPICWISE PRACTICE QUESTIONS (UNIVERSAL LAW OF GRAVITATION)
15 Videos
- GRAVITATIONBOOK - MTG GUIDECHAPTER - GRAVITATIONEXERCISE - NEET CAFE TOPICWISE PRACTICE QUESTIONS (ACCELERATION DUE TO GRAVITY AND ITS VARIATION WITH ALTITUDE AND DEPTH)
19 Videos
- GRAVITATIONBOOK - MTG GUIDECHAPTER - GRAVITATIONEXERCISE - AIPMT/NEET MCQS
32 Videos
- BEHAVIOUR OF PERFECT GAS AND KINETIC THEORYBOOK - MTG GUIDECHAPTER - BEHAVIOUR OF PERFECT GAS AND KINETIC THEORYEXERCISE - AIPMT / NEET (MCQs)
11 Videos
- KINEMATICS BOOK - MTG GUIDECHAPTER - KINEMATICS EXERCISE - AIPMT/ NEET MCQs
31 Videos
Similar Questions
Knowledge Check
In a binary star system one star has thrice the mass of other. The stars rotate about their common centre of mass then :
ABoth stars have same angular momentum about common centre of massBBoth stars have angular momentum of same magnitude about common centre of massCBoth stars have same angular speedsDBoth stars have same linear speedsA binary star system consists of two stars A and B which have time period TAandTB,radiusRBa and mass MaandMB. Then
Aif TA>TB then RA>RBBif TA>TB then MA>MBC(TATB)2=(RARB)3DTA=TBTwo stars of masses m and 2m at a distance d rotate about their common centre of mass in free space. The period of revolution is :
A12π√d33GmB2π√d33GmC12π√3Gmd3D2π√3Gmd3
A pair of stars rotates about a common centre of mass. One of the stars has a mass M and the other has mass m such that =2m. The distance between the centres of the stars is d ( d being large compare to the size of eithe star).
The period of rotation of the stars about their common centre of mass ( in terms of d,m,G) is
View SolutionIn a double star system one of mass m1 and another of mass m2 with a separation d rotate about their common centre of mass. Then rate of sweeps of area of star of mass m1 to star of mass m2 about their common centre of mass is
View SolutionA pair of stars rotates about their centre of mass One of the stars has a mass M and the other has mass m such that M=2m The distance between the centres of the stars is d (d being large compared to the size of either star) .
The period of rotation of the stars about their common centre of mass (in time of d ,m ,G) is .
View Solution_E01_055_Q01.png)
For the double star system, the two stars having masses m1 and m2are separated b a distance r. They are revolgin about their common centre of mass in radii r1 and r2 respectively. Which of the following is/are incorrect.?
View SolutionIn a double star system one of mass m1 and another of mass m2 with a separation d rotate about their common centre of mass. Then rate of sweeps of area of star of mass m1 to star of mass m2 about their common centre of mass is
View Solution
