Four charges are arranged at the corners of a square ABCD of side d as show in figure.
(a) Find the work required to put together this arrangement.
(b) A charge q0 is brought to the centre E of the square the four charges being held fixed at its corners. How much extra work is needed to do this ?
 Solution in Gujrati
Solution in Gujrati
Suppose sides of square ABCD are AB = BC =CD= DA = d. Hence AC= BD=d √2
(i) Work needed to bring charge +q to A when no charge is present on square is W1=0
(ii) Work needed to bring -q to B when +q is at A
W2=kq(−q)AB=−kq2d
(iii) Now work needed to bring charge +q to C when +q is at A and -q is at B
W3=k(+q)(+q)AC+(k−q)(+q)BC
∴W3=kq2d√2−kq2d
(iv) Now work done to bring -q to D when +q is at A ,-q at B and +q at C ,
W4=K(+q)(−q)AD+k(−q)(−q)BD+k(+q)(−q)CD
W4=−kq2d+kq2d√2−kq2d
Hence, work done to bring given charge on square,
W=W1+W2+W3+W4
∴W=0−kq2d+kq2d√2−kq2d+kq2d√2−kq2d
=−4kq2d+2kq2d√2
=−kq2d[4−√2]
W=−q24π∈0d[4−√2]
Hence, the work done depends only on the arrangement of the charges.
(b) E is the centre of square ABCD. Suppose, r is the distance of every vertex and hence
r=d√2 . If V is the potential at E .
V=k(+q)d/√2+k(−q)d/√2+k(+q)d/√2+k(−q)d/√2
∴V=0
∴ The work done to bring charge q0 at the point E
W=q0×V
=q0×0
∴W=0
Hence, no work is required to bring any charge to the centre of square. But, not on how they are brought to square vertices.
Topper's Solved these Questions
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Section B ( Numerical From Textual Exercise )46videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - Section B ( Numerical From DARPAN. Based on Textbook )19videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITANCEEXERCISE - ( Section -A) Try Yourself50videos
ELECTROSTATIC POTENTIAL AND CAPACITANCE
- BOOK - KUMAR PRAKASHANCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - BOARD.S QUESTION PAPER MARCH - 2020 (PART - B) SECTION - C5videos
ELECTROMAGNETIC WAVES
- BOOK - KUMAR PRAKASHANCHAPTER - MAGNETISM AND MATTEREXERCISE - SECTION D Multiple Choice Questions (MCQs) (MCQs asked in Competitive Exams) MCQs asked in Board Exam and GUJCET7videos
MAGNETISM AND MATTER
Similar Questions
Two charge 5×10−8C and −3×10−8 C are located 16 cm apart. At what point(s) on the line joining the two charges is the electric potential zero ? Take the potential at infinity to be zero.
06:15View SolutionAn electric field is represented by →E=Axˆi where A = 10Vm2 . Find the potential of the origin with respect to the point (10,20) m.
05:30View SolutionWhat are conservative force , non - conservative force , conservative field and non - conservative field ?
02:08View SolutionDetermine the current in each branch of the given network.
 04:14View Solution
04:14View SolutionAt a place on earth the vertical component of earth's magnetic field is √3 times its horizontal component. The angle of dip at this place is …...
07:04View SolutionFour charges are arranged at the corners of a square ABCD, as shown in the adjoining figure, The force on a positive charge kept at the centre O is
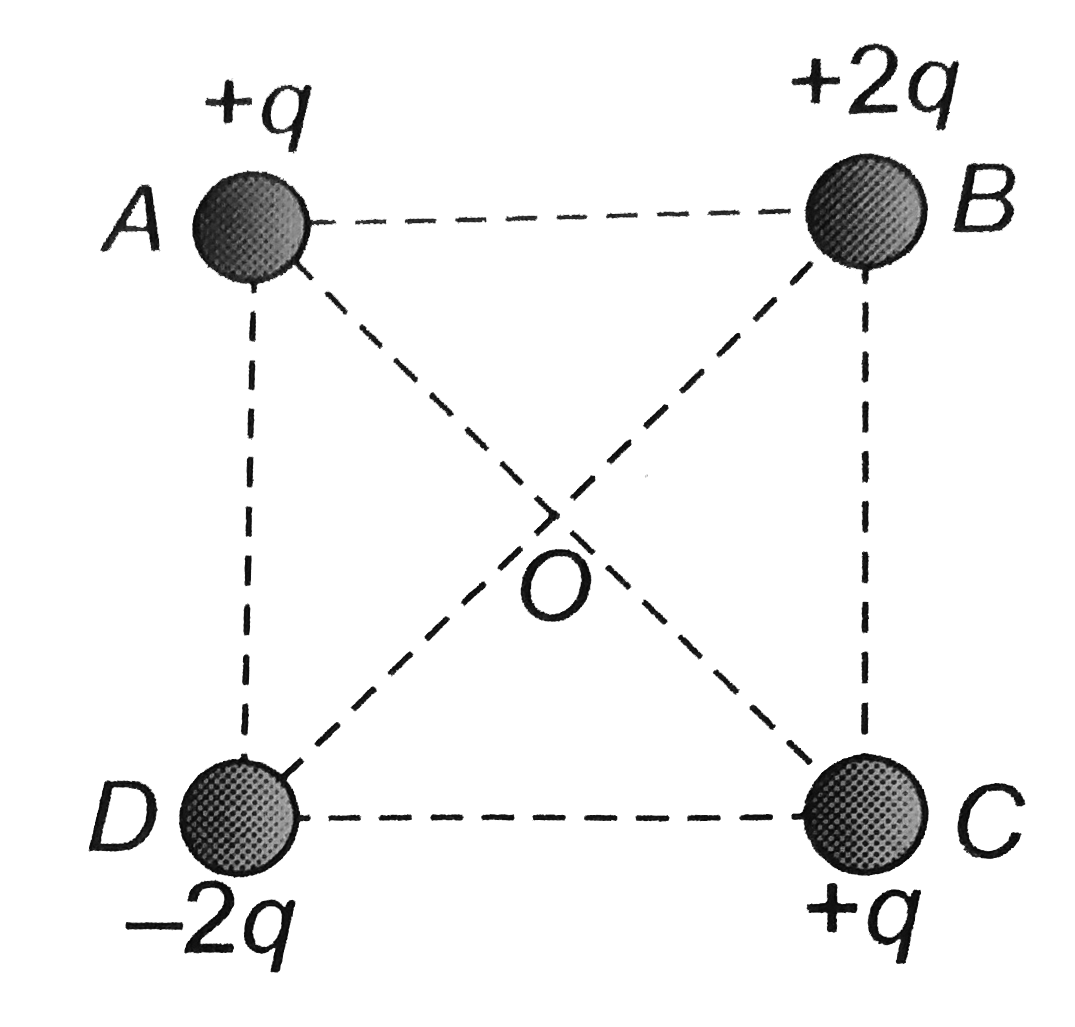 08:16View Solution
08:16View SolutionFour charges are arranged at the corners of a square ABCD pf side d, as shown in Fig. Find the work required to put together this arrangement (b) A charge q0 brought to the center E of the square, the four charges being held fixed at the corners . How much extra work in needed to do this ?
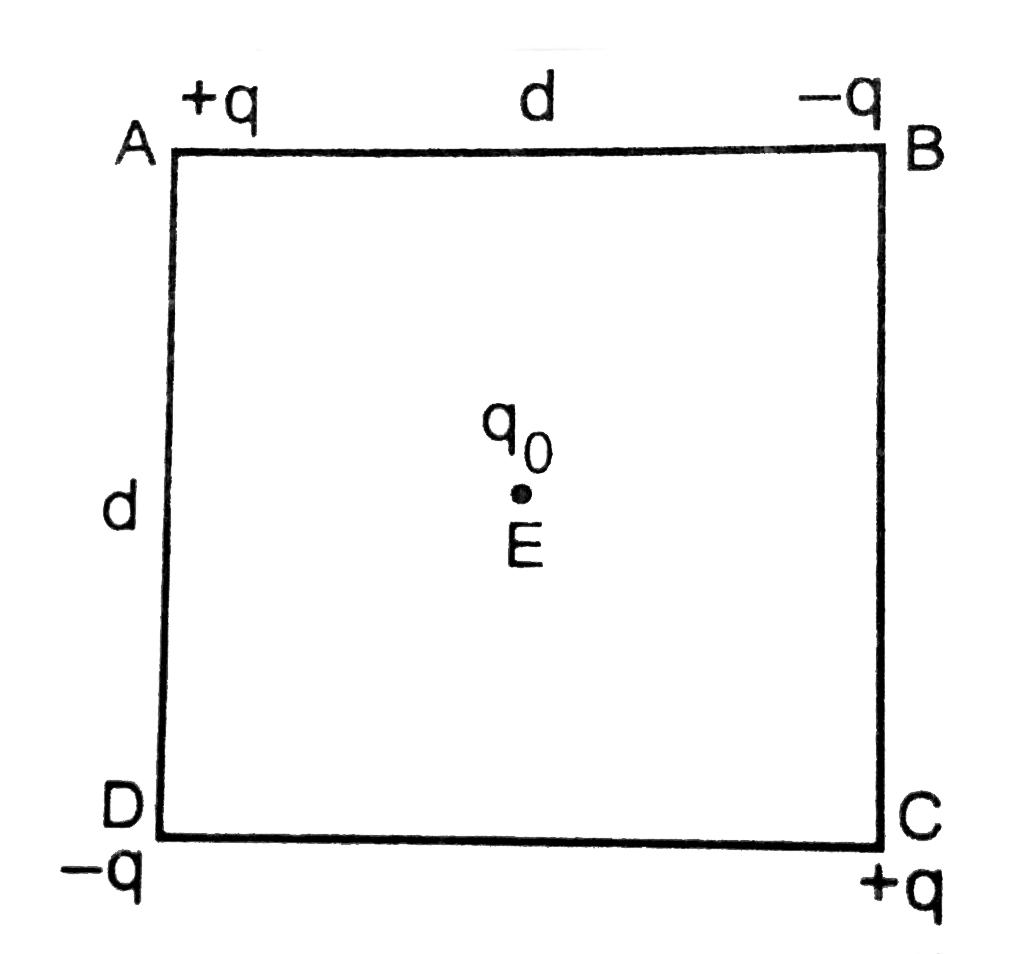 09:20View Solution
09:20View SolutionFour charges are arranged at the corners of a square ABCD as shown in the figure. The force on the charge kept at the centre O will be:
02:08View SolutionFour charges are arranged at the corners of a square ABCD, as shown. The force on a positive charge kept at the centre of the square is
 08:56View Solution
08:56View Solutionचित्र 2.15 में दर्शाए अनुशार चार आवेश भुजा d वाले किसी वर्ग ABCD के शीर्षों पर व्यवस्थित किए गए हैं। (a) इस व्यवस्था को एक साथ बनाने में किया गया कार्य ज्ञात कीजिए । (b) कोई आवेश q0 वर्ग के केंद्र E पर लाया जाता है तथा चारों आवेश अपने शीर्षों पर दृढ़ रहते हैं । ऐसा करने के लिए कितना अतिरिक्त कार्य करना पड़ता है ?
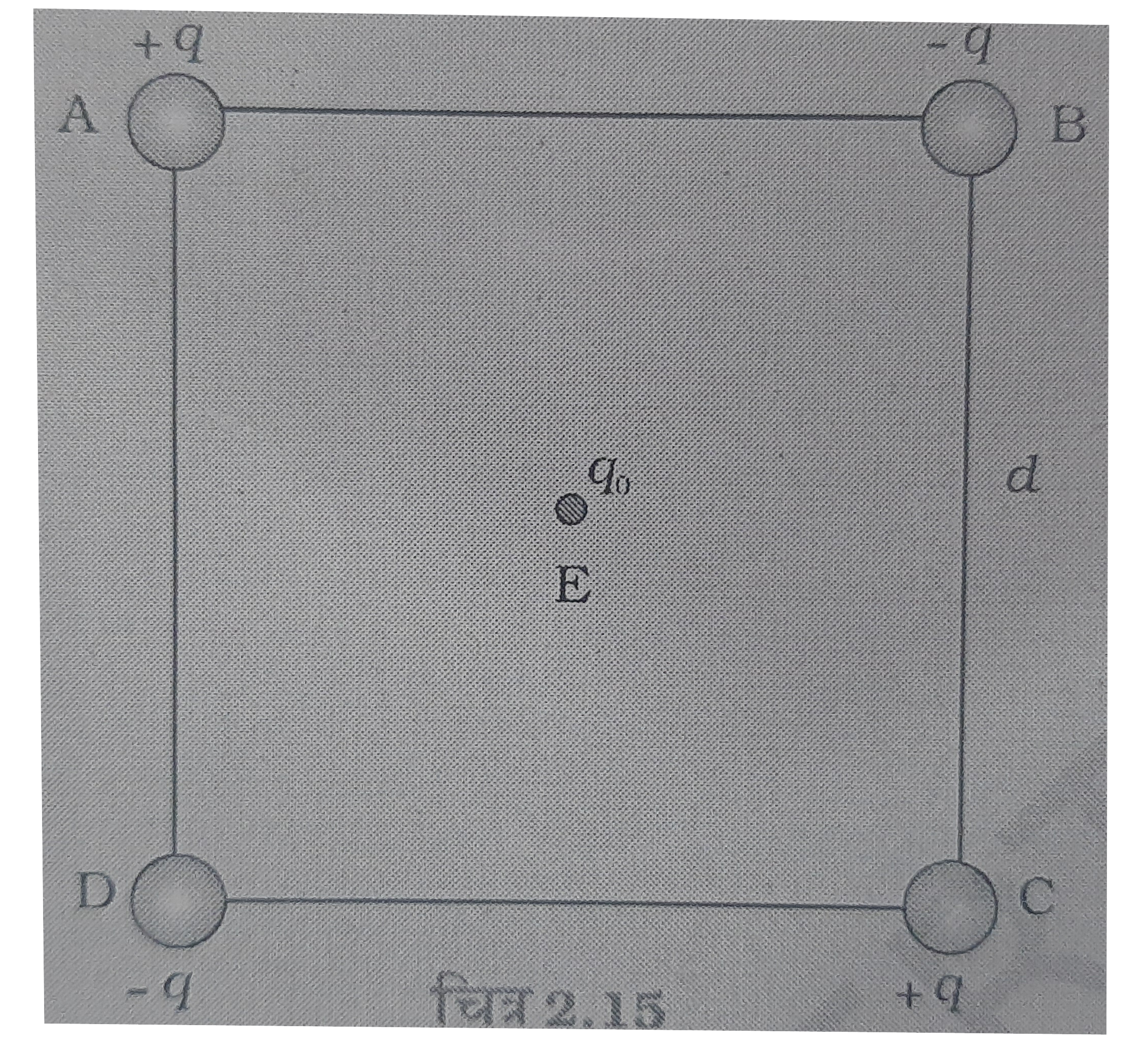 08:10View Solution
08:10View SolutionFour charges are arranged at the corners of a square ABCD of side d. as shown in fig. Find the work required to put together this arrangement. (b) A charge q0 is brought to the centre of the square, the four being held fixed at its corners. How much extra work is needed to do this ?
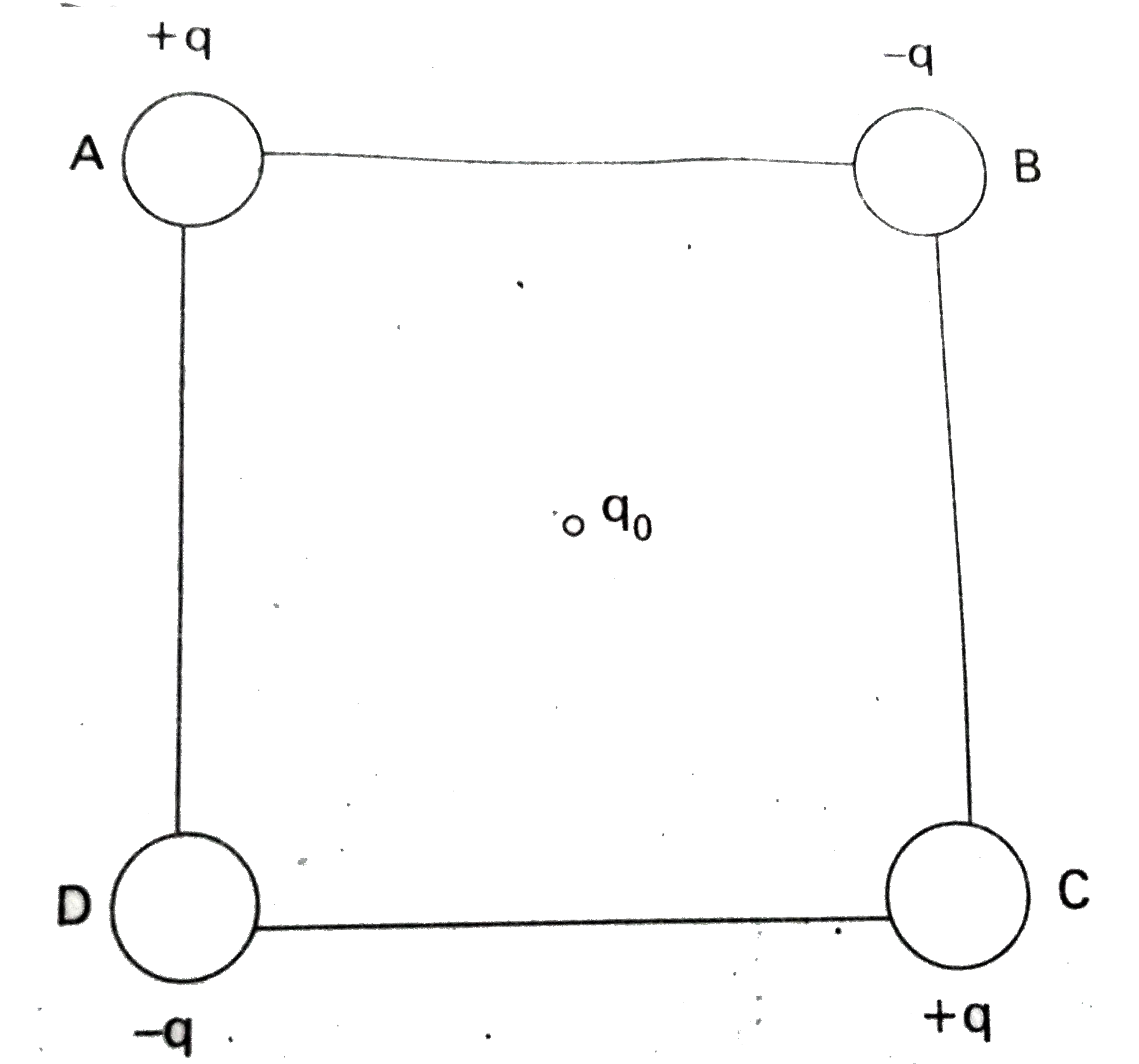 04:06View Solution
04:06View Solutionचित्र में दर्शाए अनुसार चार आवेश d भुजा वाले किसी वर्ग ABCD के शीर्षों पर व्यवस्थित किए गए है । (a). इस व्यवस्था को एक साथ बनाने में किया गया कार्य ज्ञात कीजिए (b) कोई आवेश q0 वर्ग के केंद्र E पर लाया जाता है तथा चारों आवेश अपने शीर्षों पर दृढ़ रहते हैं। ऐसा करने के लिए कितना अतिरिक्त कार्य करना पड़ता है?
 04:03View Solution
04:03View SolutionFour charges are arranged at the corners of a square ABCD as shown in the figure. The force on the charge kept at the centre O will be:
 03:10View Solution
03:10View SolutionFour charges are arranged at the corners of the square PQRS of side a as shown in the figure . (a) Find the work required to assemble these charges in the given configuration . (b) Suppose a charge q is brought to the center of the square by keeping the four charges fixed at the corners how much extra work is required for this ?
04:43View SolutionFour charges are arranged at the comers of a square ABCD of side d, as shown in Fig.
a. Find the work required to put together this arrangement
b. A charge q0 is brought to the centre E of the square, the four charges being held fixed at its corners. How much extra work is needed to do this?n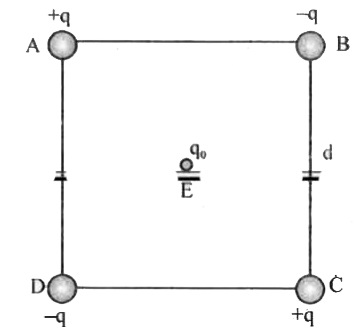 08:02View Solution
08:02View Solution

