A particle located at x=0 at time t=0, starts moving along with the positive x−direction with a velocity 'v' that varies as v=a√x. The displacement of the particle varies with time as
t3
t2
t
t1/2
The correct Answer is:B
v=α√x or dxdt=α√x or dx√x=α.dt
or ∫dx√x=α∫dt or 2x1/2=αt+C1
where C1 is the constant of integration .
Given : x=0 at t=0,∴C1=0
∴2x1/2=αt or x=(α2)2t2 or x∝t2
Topper's Solved these Questions
- BOOK - MTG GUIDECHAPTER - KINEMATICS EXERCISE - Topicwise Practice Questions (ACCELERATION)12videos
KINEMATICS
- BOOK - MTG GUIDECHAPTER - KINEMATICS EXERCISE - Topicwise Practice Questions (GRAPHICAL STUDY OF MOTION)16videos
KINEMATICS
- BOOK - MTG GUIDECHAPTER - KINEMATICS EXERCISE - Topicwise Practice Questions (DISTANCE AND DISPLACEMENT)2videos
KINEMATICS
- BOOK - MTG GUIDECHAPTER - GRAVITATIONEXERCISE - AIPMT/NEET MCQS32videos
GRAVITATION
- BOOK - MTG GUIDECHAPTER - LAWS OF MOTIONEXERCISE - AIPMT /NEET (MCQ)24videos
LAWS OF MOTION
Similar Questions
A particle located at "x=0" at time "t=0" starts moving along the positive "x" - direction with a velocity "v" that varies as "v=alpha sqrt(x)".The displacement "(x)" of the particle varies with time as "(alpha" is constant)
View SolutionA particle located at position x=0, at time t=0, starts moving along the positive x-direction with a velocity v2=αx (where alpha is a positive constant). The displacement of particle is proportional to
View Solution
Knowledge Check
- Question 1 - Select One
A particle located at x = 0 at time t = 0, starts moving along the positive x-direction with a velocity that varies as v=p√x. The displacement of the particle varies with time as (where, p is constant)
At3Bt2CtDt1/2 - Question 2 - Select One
If the displacement of a particle varies with time as √x=t+3
Avelocity of the particle is inversely proportional to tBvelocity of particle varies linearly with tCvelocity of particle is proportional to √tDinitial velocity of the particle is zero - Question 3 - Select One
If the displacement of a particle varies with time as √x=t+7 . The -
Avelocity of the particle is inversely proportional to tBvelocity of the particle is proportional to tCvelocity of the particle is proportional to √tDthe particle moves with a constant acceleration
The displacement to particle is zero at t=0andiszatt=t. It starts moving in the positive x-direction with a velocity which varies, v=k√x, wher (k) is a constant. Find the relation for variation of velocity with time.
View SolutionThe dispkacement of particle is zero at t=0 and it is x , at t−t. It starts moving in the positive x-direction with a velocity which varices as v=k√x, where (k) is a constant. Show that velocity is directly proprtional to time.
View SolutionIf the velocity "v" of a particle varies as the square of its displacement "x" then the acceleration varies as
View SolutionTwo particles 1 and 2 start simultaneously from origin and move along the positive X direction. Initial velocity of both particles is zero. The acceleration of the two particles depends on their displacement (x) as shown in fig.
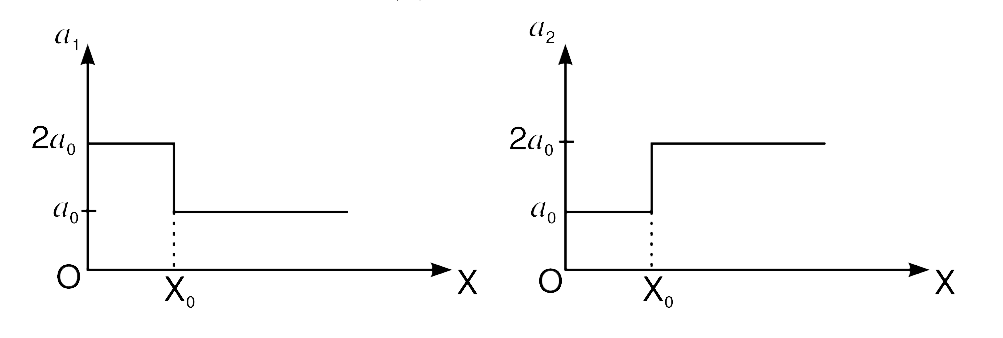
(a) Particles 1 and 2 take t1 and t2 time respectively for their displacement to become x0. Find t2t1.
(b) Which particle will cover 2x0 distance in least time? Which particle will cross the point x=2x0 with greater speed?
(c) The two particles have same speed at a certain time after the start. Calculate this common speed in terms of a0 and x0.View Solution" If the velocity v of a particle varies as the square of its displacement "x" then the acceleration varies as "
View Solution

