[Telugu] For the circle C with the equation x^(2) + y^(2) - 16x

The circle with centre (1,1) and radius 1 is

The circle with centre $(\frac{3}{2}, \frac{1}{2})$ and radius $\sqrt{\frac{3}{2}}$ is

Discuss the relative position of the fol-
lowing pair of circles.
$x^{2} + y^{2} - 4 x - 6 y - 12 = 0$
$x^{2} + y^{2} + 6 x + 18 y + 26 = 0$ .

View Solution

The value of $13 \sum n = 1 (i^{n} + i^{n + 1})$ , where $i = \sqrt{- 1}$ is

View Solution

List-I contains angles in radians and List-II contains the quadrant in which the angle lies.
$∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣ \begin{matrix} List-I & List-II (P) & 2^{c} & (1) & I (Q) & 4^{c} & (2) & II (R) & 6^{c} & (3) & III (S) & 7^{c} & (4) & IV \end{matrix} ∣ ∣ ∣ ∣ ∣ ∣ ∣ ∣$

View Solution

In respect of the equation $[\begin{matrix} 2 & 3 4 & 6 \end{matrix}] [\begin{matrix} x y \end{matrix}] = [\begin{matrix} 5 c - 5 \end{matrix}]$
correctly match List I with List II and select the correct answer using the code given below the lists :
$\begin{matrix} List I & List II (Value of c)) & (Nature of the Equation) A . & 5 & 1 . The equation has no solution. B . & 10 & 2 . The equation has a unique solution. C . & 15 & 3 . The equation has an infinite set of solution. 4 . The equation has two infinite sets of independent solution \end{matrix}$

02:23

View Solution

Let $α and β$ be the roots of the quadratic equation $a x^{2} + b x + c = 0$ . Observe the lists given below:
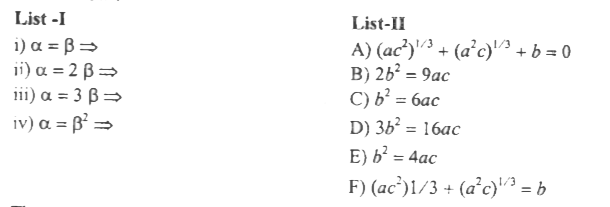
ocr_image
Let correct match of List -I from List -II is

03:55

View Solution

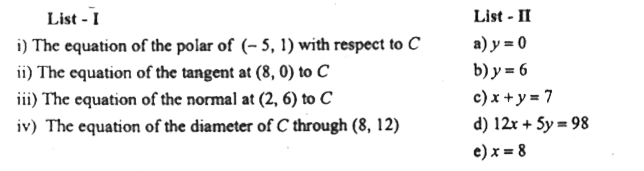
For the circle C with the equation $x^{2} + y^{2} - 16 x - 12 y + 64 = 0$ match the list-I with the list-II given below: ocr_image

View Solution

Given the circle C with the equation $x^{2} + y^{2} - 2 x + 10 y - 38 = 0$ Match the List-I with the List-II given below concening C: ocr_image

08:27

View Solution

For the ellipse (x^(2))/(25)+(y^(2))/(16) = 1 , a list of lines given in List I are to be matched with their equation given in List II.

07:23

View Solution

For the ellipse given by $\frac{{(x - 3)}^{2}}{25} + \frac{{(y - 2)}^{2}}{16} = 1$ , match the equations of the lines given in List I with those on the List Ii.
$\begin{matrix} List I & List II i . The equation of the minor axis & p . 3 x = 34 i i . The equation of a latusrectum & r . x + y = 9 s . x = 6 f . x = 3 u . 3 y = 34 \end{matrix}$

View Solution

Match the items of List - I with those of List - II
$\begin{matrix} List - I & List - II (a) Equation of the tangent drawn at (2, \sqrt{8}) on the curve y^{2} = 4 x is & (i) - 36 (b) Equation of the normal to the curve y^{2} = 16 x, that makes an angle of 45^{\circ} with its axis is & (ii) 4 (c) The chord joining the points (x_{1}, y_{1}) and (x_{2}, y_{2}) on the curve y^{2} = 12 x is a focal chord if y_{1} y_{2} = & (iii) 8 (d) A value of k for which x - 3 = 0 is the directrix of the curve y^{2} - k x + 16 = 0 is & (iv) x = \sqrt{2} y + 2 = 0 (v) x + y - 12 = 0 (vi) x - y - 12 = 0 \end{matrix}$