A random variable X has its range {−1,0,1}. If its mean is 0.2 and P(X=0)=0.2 ,then P(X=1)=
Step by step video & image solution for A random variable X has its range {-1,0,1}. If its mean is 0.2 and P(X=0)=0.2 ,then P(X=1)= by Maths experts to help you in doubts & scoring excellent marks in Class 11 exams.
Similar Questions
A random variable X takes the value -1,0,1. It's mean is 0.6. If P(X=0)=0.2 then P(X=1)=
View SolutionA random variable x has its range {0,1,2} and the probabilities are given by P(x=0)=3k3,P(x=1)=4k−10k2,P(x=2)=5k−1 where k is a constant then k=
View SolutionA random variate X takes the values 0, 1, 2, 3 and its mean is 1.3. If P(X=3)=2P(X=1) and P(X=2)=0.3, then P(X=0) is equal to
View SolutionA random variable Xtakes the values0,1and2.If P(X=1)=P(X=2)andP(X=0)=0.4,then the mean value of the random variableX is
View SolutionA random variable X has its range (1 2 3} IfP(X=1)=αP(X=2)=β,p(X=3)=16 and the mean is53then α,β=
View SolutionIf a random variable x assumes the values 0,1 and 2 with probabilities 0.30,0.50 and 0.20, then its expected value is (A) 1.50 (B) 3 (C) 0.90 (D) 1
View SolutionIf the random variable 1follows a Poisson distribution with mean 3 then P(X=0) is
View SolutionLet x be a random variable such that the probability function of a distribution is given by P(X=0)=12,P(X=J)=13j(j=1,2,3,…..,,∞) then the mean of the distribution and P(X is positive and even) respectively are:
View SolutionA random variable X has the follwing probability distribution:
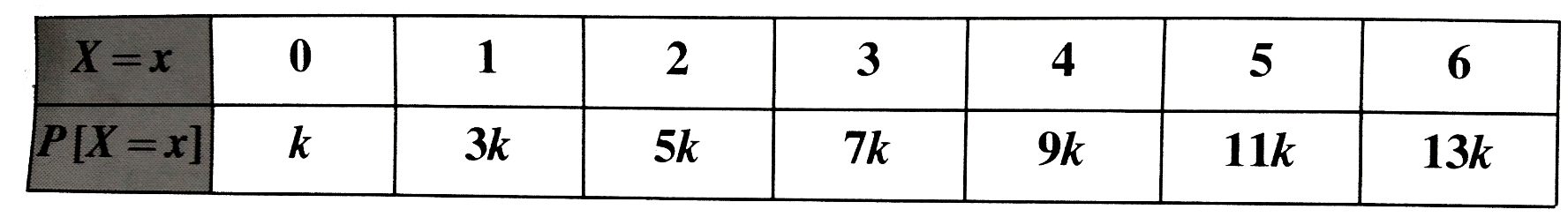
Find P(0<X<4)View SolutionGiven a random variable that takes values X={0,1,2} and has a probability distribution, P(0)=14,P(1)=12,P(2)=14. Then find the variance of X.
View Solution
