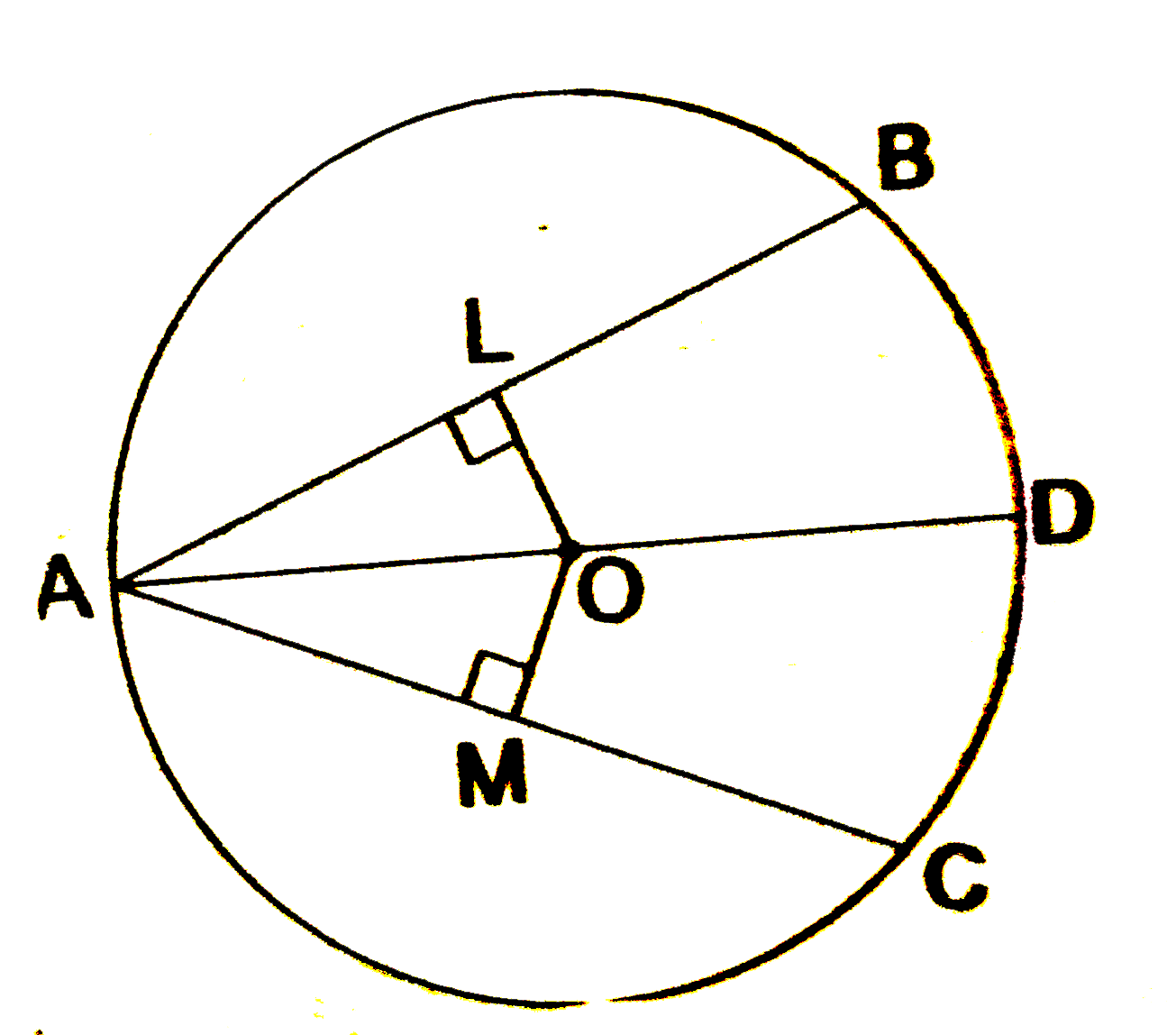
If two chords of a circle are equally inclined to the diameter through their point of intersection, prove that the chords are equal.
Given Two chords AB and AC of a circle C(O,r) and AOD is a diameter such that ∠AOB=∠OAC.
To Prove AB= AC
Construction Draw OL ⊥AB and OM⊥AC.
Proof In ΔOLA and ΔOMA , we have
∠OLA=∠OAC [each equal to 90∘]
OA=OA [common]
∠OAL=∠OAM [given ]
∴ΔOLA=≅ΔOMA
⇒OL=OM
⇒ chords AB and AC are equidistant from O.
⇒AB=AC.
Similar Questions
If two intersecting chords of a circle make equal angles with the diameterpassing through their point of intersection, prove that the chords are equal
View SolutionEqual chords of a circle subtend equal angles at the centre.
View SolutionIf two equal chords of a circle intersect within the circle, prove that the line joining the point of intersection to the center makes equal angles with the chords.
View SolutionEqual chords of a circle are equidistant from circle.
View SolutionIf two chords of a congruent circle are equal; then their corresponding arcs are also equal.
View SolutionEqual chord of circle subtends equal angle on centre.
View SolutionIf two equal chords of a circle in intersect within the circle, prove that : the segments of the chord are equal to the corresponding segments of the other chord. the line joining the point of intersection to the centre makes equal angles with the chords.
View SolutionIf two equal chords of a circle in intersect within the circle, prove that: the segments of the chord are equal to the corresponding segments of the other chord. the line joining the point of intersection to the centre makes equal angles with the chords.
View SolutionIf a line segment joining mid-points of two chords of a circle passes through the centre of the circle, prove that the two chords are parallel.
View SolutionTwo chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the angle bisector of ∠BAC.
View Solution

