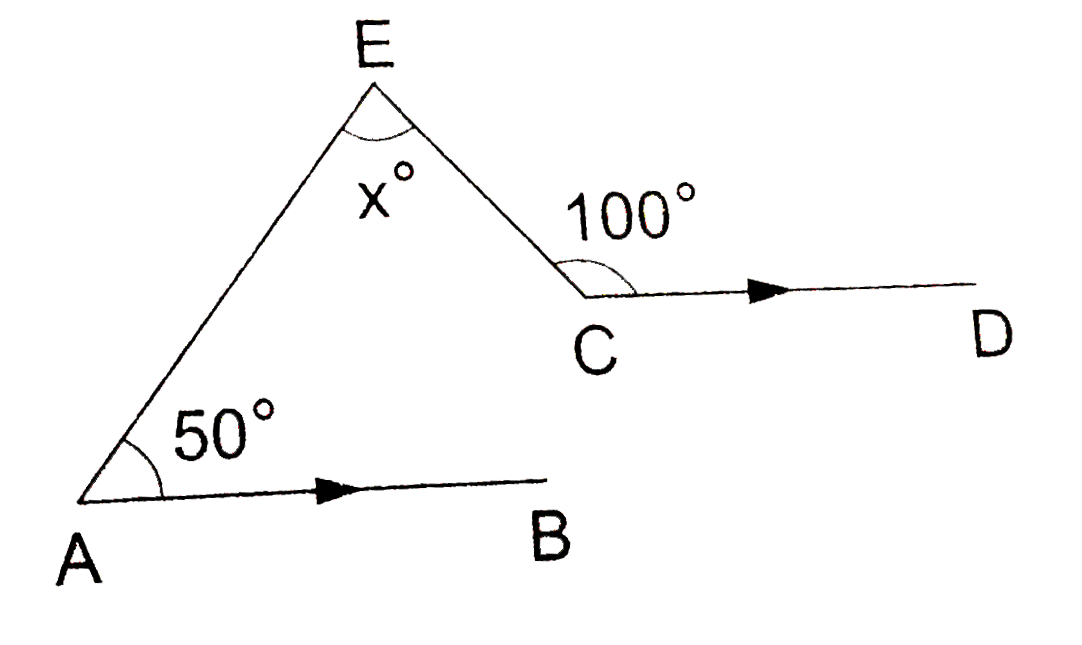
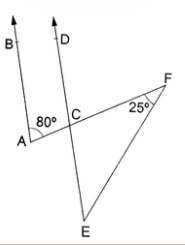
In the given figure, AB∣∣CD,∠EAB=105∘,∠AEC=25∘ and ∠ECD=x∘. Find the value of x.

A
x=120
B
x=140
C
x=130
D
x=150
Video Solution
Text Solution
The correct Answer is:C
From E, draw EF||AB||CD.
Now, EF∣∣CD and CE is the transversal.
∴∠DCE+∠CEF=180∘ [co. int. ∠s]
⇒x∘+∠CEF=180∘
⇒∠CEF=(180∘−x∘).
Again, EF∣∣AB and AE is the transversal.
∴∠BAE+∠AEF=180∘ [co. int. ∠s]
⇒105∘+∠AEC+∠CEF=180∘
⇒105∘+25∘+(180∘−x∘)=180∘
=x∘=130∘.
Hence, x=130.
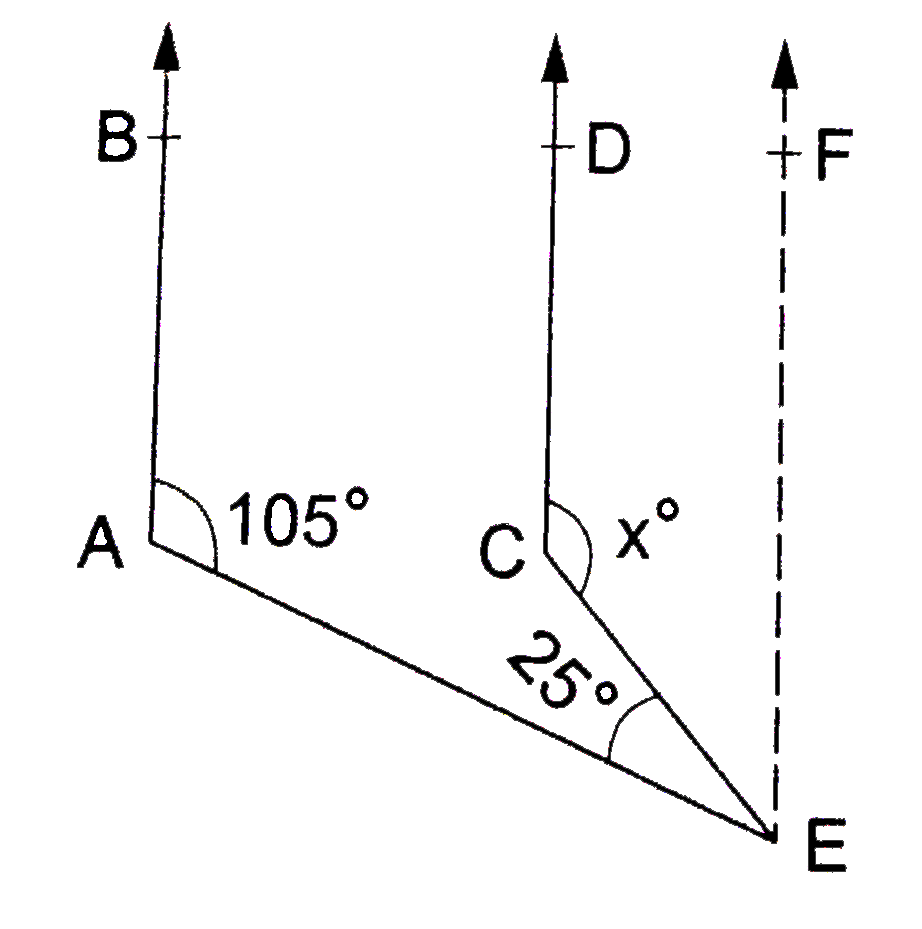
|
Topper's Solved these Questions
- LINES AND ANGLESBook:RS AGGARWALChapter:LINES AND ANGLESExercise:Example
Explore 13 Videos
- LINES AND ANGLESBook:RS AGGARWALChapter:LINES AND ANGLESExercise:Exercise 7A
Explore 12 Videos
- LINEAR EQUATIONS IN TWO VARIABLESBook:RS AGGARWALChapter:LINEAR EQUATIONS IN TWO VARIABLESExercise:Multiple Choice Questions (Mcq)
Explore 24 Videos
- MEAN , MEDIAN AND MODE OF UNGROUPED DATA Book:RS AGGARWALChapter:MEAN , MEDIAN AND MODE OF UNGROUPED DATA Exercise:Multiple Choice Questions (Mcq)
Explore 18 Videos
Similar Questions
Knowledge Check
In the given figure, AB∣∣CD,∠ABE=120∘,∠ECD=100∘ and ∠BEC=x∘. Find the value of x.
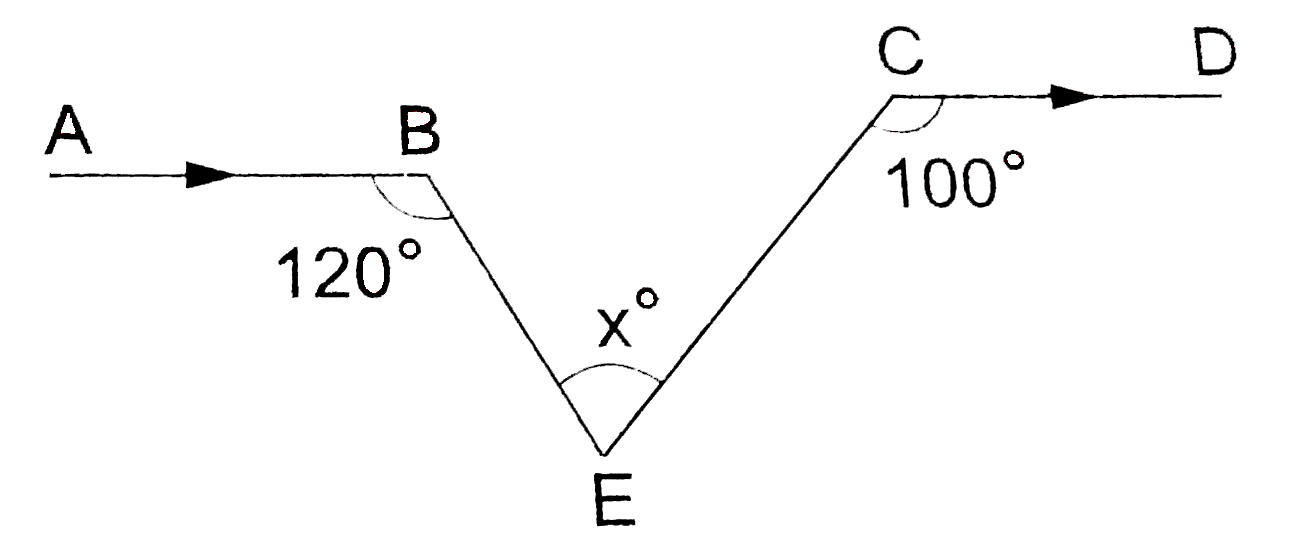 Ax=30Bx=20Cx=50Dx=40
Ax=30Bx=20Cx=50Dx=40In the given figreu AB∣∣CD,∠BAD=30∘and∠ECD=50∘, find ∠CED
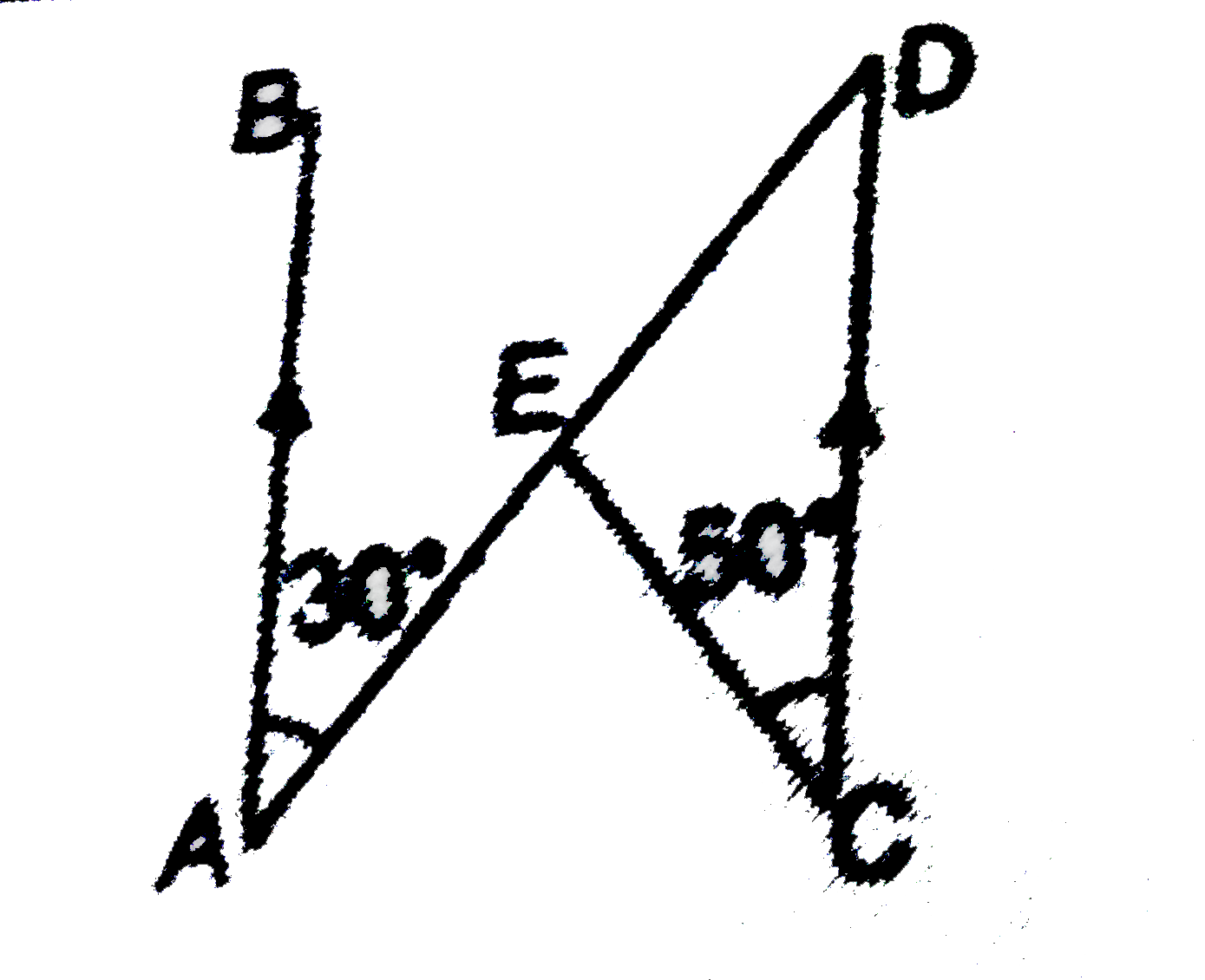 A120∘B30∘C100∘D50∘
A120∘B30∘C100∘D50∘In the given figure , AB∣∣CD. Find x.
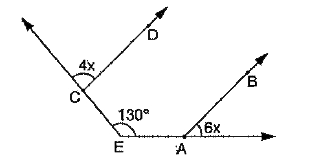 A26∘B13∘C23∘D46∘
A26∘B13∘C23∘D46∘
In the given figure, AB∣∣CD and ∠AOC=x∘. If ∠OAB=104∘ and ∠OCD=116∘, find the value of x.
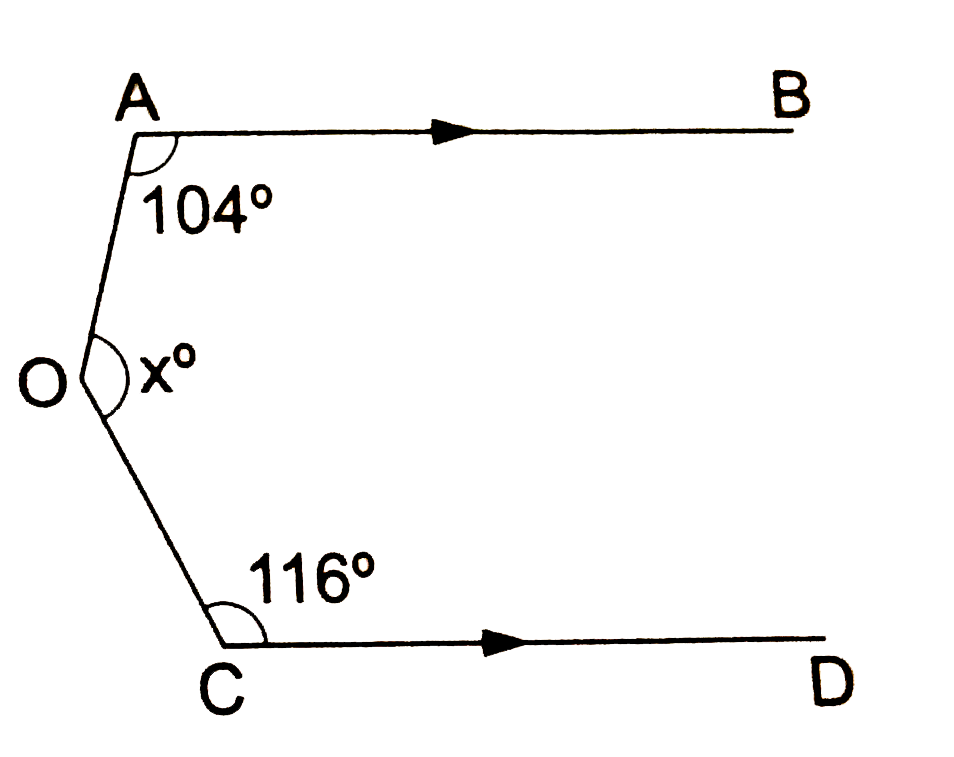
View SolutionIn the given figure, AB∣∣CD. If ∠EAB=50∘ and ∠ECD=60∘ then ∠AEB=?
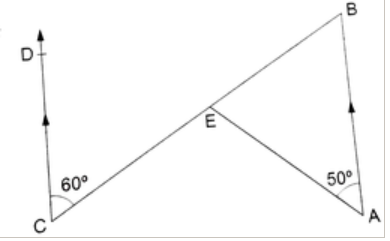
View SolutionIn the given figure if AB||CD ∠APQ=50∘ and ∠PRD=127∘ find x and y.
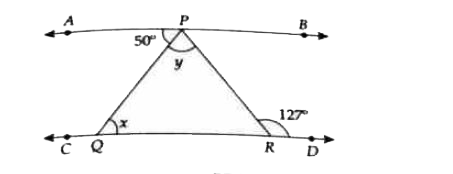
View SolutionIn the given figure, if AB∣∣CD,∠APQ=50∘ and ∠PRD=127∘, find x and y.

View SolutionIn the given figure, AB∣∣CD,∠ABO=40∘,∠CDO=35∘. Find the value of the reflex ∠BOD and hence the value of x.
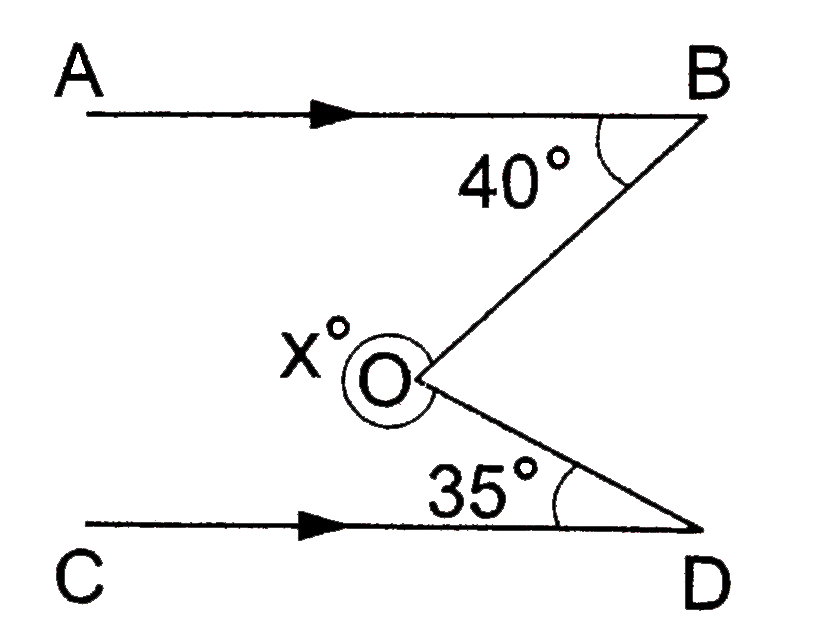
View Solution